Witt theorem

Any isometry between two subspaces and of a finite-dimensional vector space , defined over a field of characteristic different from 2 and provided with a metric structure induced from a non-degenerate symmetric or skew-symmetric bilinear form , may be extended to a metric automorphism of the entire space . The theorem was first obtained by E. Witt [1].

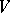Witt's theorem may also be proved under wider assumptions on and [2], [3]. In fact, the theorem remains valid if is a skew-field, is a finite-dimensional left 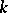-module and 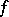is a non-degenerate -Hermitian form (with respect to some fixed involutory anti-automorphism of 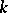, cf. Hermitian form) satisfying the following condition: For any 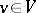there exists an element such that

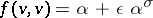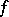(property ). Property holds if, for example, is a Hermitian form and the characteristic of 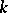is different from 2, or if 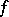is an alternating form. Witt's theorem is also valid if 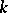is a field and 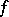is the symmetric bilinear form associated with a non-degenerate quadratic form 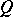on 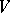. It follows from Witt's theorem that the group of metric automorphisms of 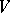transitively permutes the totally-isotropic subspaces of the same dimension and that all maximal totally-isotropic subspaces in 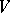have the same dimension (the Witt index of 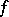). A second consequence of Witt's theorem may be stated as follows: The isometry classes of non-degenerate symmetric bilinear forms of finite rank over with direct orthogonal sum form a monoid with cancellation; the canonical mapping of this monoid into its Grothendieck group is injective. The group is called the Witt–Grothendieck group 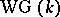of 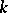; the tensor product of forms induces on it the structure of a ring, which is known as the Witt–Grothendieck of [7].

For other applications of Witt's theorem see Witt decomposition; Witt ring.

References

[1]	E. Witt, "Theorie der quadratischen formen in beliebigen Körpern" J. Reine Angew. Math. , 176 (1937) pp. 31–44
[2]	N. Bourbaki, "Elements of mathematics. Algebra: Algebraic structures. Linear algebra" , Elements of mathematics , 1 , Addison-Wesley (1974) pp. Chapts. 1–2 (Translated from French)
[3]	J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1955)
[4]	S. Lang, "Algebra" , Addison-Wesley (1974)
[5]	E. Artin, "Geometric algebra" , Interscience (1957)
[6]	J.-P. Serre, "A course in arithmetic" , Springer (1973) (Translated from French)
[7]	J. Milnor, "Algebraic 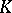-theory and quadratic forms" Invent. Math. , 9 (1969/70) pp. 318–344