Weierstrass representation of a minimal surface

Let 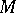be a Riemann surface. A harmonic conformal mapping 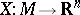then defines a minimal surface in 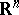, 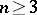(cf. also Harmonic function; Conformal mapping). Let 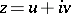be local isothermal coordinates; then

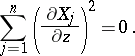Since 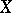is harmonic,

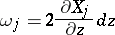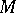is a holomorphic -form on . Hence any (branched) minimal surface in 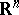can be given by meromorphic -forms 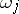satisfying 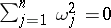, and can be expressed as

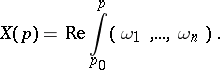(a1)

Such an is well defined on if and only if for any loop in ,

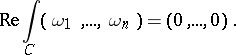(a2)

For 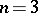, one gets a meromorphic function and a meromorphic -form ,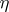

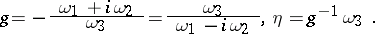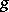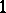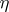On the other hand, given a meromorphic function and a meromorphic -form on 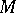, define

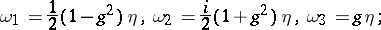(a3)

then . Thus, (a3) together with (a1) defines a minimal surface in 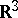and is called the Weierstrass representation of the minimal surface via the Weierstrass data .

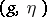The meromorphic function 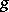has the geometric meaning that it is the composite of the spherical mapping (or unit normal vector) 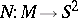and the stereographic projection from the north pole, where

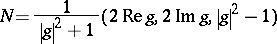and 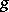is also called the Gauss map of the minimal surface.

The first fundamental form and the Gaussian curvature of the surface 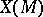can be expressed via ,

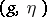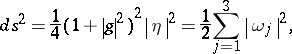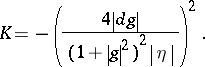Hence 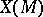is a regular surface if and only if 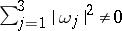on .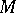

The second fundamental form of can be expressed as

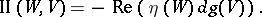Moreover, 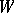is an asymptotic direction if and only if 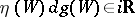, and 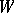is a principal curvature direction if and only if .

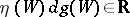The local Weierstrass representation was discovered in the 1860{}s by K. Enneper and K. Weierstrass. R. Osserman gave the general form on a Riemann surface in the 1960{}s, see [a1] for more details.

References

[a1]	R. Osserman., "A survey of minimal surfaces" , Dover (1986)