Unramified character
A character (cf. Character of a group) of the Galois group  of a Galois extension of local fields
of a Galois extension of local fields  that is trivial on the inertia subgroup. Any unramified character can be regarded as a character of the Galois group of the extension
that is trivial on the inertia subgroup. Any unramified character can be regarded as a character of the Galois group of the extension  , where
, where  is the maximal unramified subfield of the extension
is the maximal unramified subfield of the extension  . The unramified characters form a subgroup of the group of all characters. A character of the multiplicative group
. The unramified characters form a subgroup of the group of all characters. A character of the multiplicative group  of a local field
of a local field  that is trivial on the group of units of
that is trivial on the group of units of  is also called unramified. This definition is compatible with the preceding one, because by the fundamental theorem of local class field theory there is for every Abelian extension of local fields
is also called unramified. This definition is compatible with the preceding one, because by the fundamental theorem of local class field theory there is for every Abelian extension of local fields  a canonical reciprocity homomorphism
a canonical reciprocity homomorphism  that enables one to identify the set of characters of the group
that enables one to identify the set of characters of the group 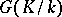 with a certain subgroup of the character group of
with a certain subgroup of the character group of 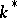 .
.
For a Galois extension of global fields  a character
a character  of the Galois group
of the Galois group  is said to be unramified at a point
is said to be unramified at a point 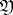 of
of  if it remains unramified in the above sense under restriction to the decomposition subgroup of any point
if it remains unramified in the above sense under restriction to the decomposition subgroup of any point 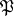 of
of  lying over
lying over 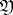 . Similarly, a character
. Similarly, a character  of the idèle class group
of the idèle class group  of
of 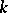 is called unramified at
is called unramified at  if its restriction to the subgroup of units of the completion
if its restriction to the subgroup of units of the completion 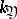 of
of  relative to
relative to 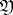 is trivial, where the group
is trivial, where the group  is imbedded in the standard way in
is imbedded in the standard way in  .
.
From global class field theory it follows that these two definitions of being unramified at a point  are compatible, as in the local case.
are compatible, as in the local case.
References
| [1] | A. Weil, "Basic number theory" , Springer (1974) |
Comments
See Ramified prime ideal and Inertial prime number for the notion of inertia subgroup.
Unramified character. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Unramified_character&oldid=11267