Riemann surface
of an analytic function  of a complex variable
of a complex variable 
A surface 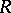 such that the complete analytic function
such that the complete analytic function 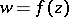 , which is, in general, multiple-valued, can be considered as a single-valued analytic function
, which is, in general, multiple-valued, can be considered as a single-valued analytic function 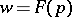 of a point
of a point  on
on 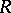 .
.
The concept of a Riemann surface arose in connection with the studies of algebraic functions 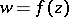 defined by an algebraic equation
defined by an algebraic equation
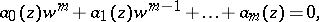 | (1) |
where 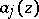 ,
, 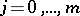 , are polynomials with constant coefficients,
, are polynomials with constant coefficients,  ,
,  . In the works of V. Puiseux (1850–1851) one discovers a clear understanding of multiple-valuedness, characteristic of these functions
. In the works of V. Puiseux (1850–1851) one discovers a clear understanding of multiple-valuedness, characteristic of these functions 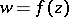 , when to each value of the variable
, when to each value of the variable  ,
, 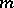 values of the variable
values of the variable 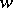 correspond. B. Riemann (1851–1857, see [1]) was the first to show how for any algebraic function one can construct a surface on which this function can be considered as a single-valued rational function of the point. The obtained Riemann surface can be identified with the algebraic curve defined by equation (1). In general, a mutual penetration (sometimes more intensive, sometimes less intensive) of ideas and methods of the theory of functions of a complex variable on the one hand and of algebra and algebraic geometry on the other hand is characteristic of the whole period of further development of the theory of Riemann surfaces, associated with the names of F. Klein, H. Poincaré, P. Koebe, and others. The landmark of this development was the first edition of the book of H. Weyl [18], in which the general concept of an abstract Riemann surface was formulated.
correspond. B. Riemann (1851–1857, see [1]) was the first to show how for any algebraic function one can construct a surface on which this function can be considered as a single-valued rational function of the point. The obtained Riemann surface can be identified with the algebraic curve defined by equation (1). In general, a mutual penetration (sometimes more intensive, sometimes less intensive) of ideas and methods of the theory of functions of a complex variable on the one hand and of algebra and algebraic geometry on the other hand is characteristic of the whole period of further development of the theory of Riemann surfaces, associated with the names of F. Klein, H. Poincaré, P. Koebe, and others. The landmark of this development was the first edition of the book of H. Weyl [18], in which the general concept of an abstract Riemann surface was formulated.
Definition A: A connected topological Hausdorff space  is called an abstract Riemann surface or, simply, a Riemann surface, if it admits a covering by open sets
is called an abstract Riemann surface or, simply, a Riemann surface, if it admits a covering by open sets 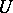 together with a homeomorphism
together with a homeomorphism 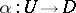 corresponding to each set
corresponding to each set  , where
, where 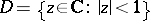 is the unit disc in the complex
is the unit disc in the complex  -plane
-plane 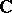 ; moreover, if a point
; moreover, if a point  belongs to
belongs to 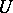 and
and  , then the one-to-one correspondence
, then the one-to-one correspondence 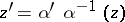 should be a conformal mapping of the first kind in a neighbourhood of the point
should be a conformal mapping of the first kind in a neighbourhood of the point 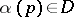 , that is,
, that is, 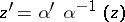 is a univalent analytic function in a neighbourhood of the point
is a univalent analytic function in a neighbourhood of the point 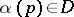 . In other words, an abstract Riemann surface is a two-dimensional complex-analytic manifold.
. In other words, an abstract Riemann surface is a two-dimensional complex-analytic manifold.
The definition of a Riemann surface with boundary 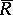 differs from definition
differs from definition 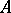 by the fact that together with the homeomorphisms
by the fact that together with the homeomorphisms 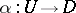 , homeomorphisms
, homeomorphisms 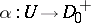 are admitted, where
are admitted, where 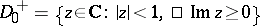 is the unit upper half-disc in
is the unit upper half-disc in 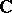 ; moreover, it is usually assumed that
; moreover, it is usually assumed that  is not already a Riemann surface in the sense of definition
is not already a Riemann surface in the sense of definition 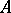 . The points of a Riemann surface with boundary
. The points of a Riemann surface with boundary 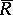 that have neighbourhoods homeomorphic to
that have neighbourhoods homeomorphic to 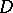 are called interior and the other points, that are mapped to the points of the segment
are called interior and the other points, that are mapped to the points of the segment
 |
form the boundary 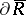 . The set of interior points of
. The set of interior points of 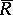 (the interior of
(the interior of 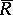 ) is a Riemann surface in the sense of definition
) is a Riemann surface in the sense of definition  . Thus, in the case of a Riemann surface with boundary, the boundary is usually considered to be a non-empty set.
. Thus, in the case of a Riemann surface with boundary, the boundary is usually considered to be a non-empty set.
A Riemann surface (with boundary) is a triangulable and orientable manifold with a countable base and, hence, it is separable and metrizable. A compact Riemann surface (without boundary) is called a closed Riemann surface; the wider class of finite Riemann surfaces includes the closed Riemann surfaces and the compact Riemann surfaces with a boundary consisting of a finite number of connected components. Non-compact Riemann surfaces with boundary or without it are called open Riemann surfaces. In certain cases it is more convenient to admit in definition 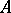 not only conformal mappings of the first kind but also conformal mappings of the second kind. A Riemann surface with boundary
not only conformal mappings of the first kind but also conformal mappings of the second kind. A Riemann surface with boundary 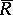 (or without it) obtained using such an approach is, generally speaking, not orientable any more, but under the assumption that it be finite it can be conformally imbedded in an orientable closed Riemann surface: the double of
(or without it) obtained using such an approach is, generally speaking, not orientable any more, but under the assumption that it be finite it can be conformally imbedded in an orientable closed Riemann surface: the double of 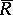 (see [8], cf. Double of a Riemann surface).
(see [8], cf. Double of a Riemann surface).
Let an analytic function 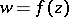 be given by one of its regular elements
be given by one of its regular elements 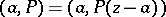 , i.e. by a pair consisting of a point
, i.e. by a pair consisting of a point 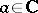 and a power series
and a power series
 |
with centre  and radius of convergence
and radius of convergence  ,
, 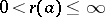 . Analytic continuation of the element
. Analytic continuation of the element 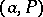 along all possible paths in the extended plane
along all possible paths in the extended plane 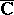 allows one to obtain all regular elements
allows one to obtain all regular elements  of the same type; in their totality they form the complete analytic function, which is also denoted by
of the same type; in their totality they form the complete analytic function, which is also denoted by 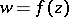 . Moreover, under analytic continuation elements of a more general nature arise:
. Moreover, under analytic continuation elements of a more general nature arise:
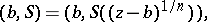 |
i.e. pairs consisting of a point  and a generalized power series (a Puiseux series):
and a generalized power series (a Puiseux series):
 |
or (in the case when 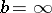 is the point at infinity):
is the point at infinity):
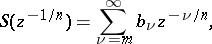 |
where 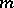 is an integer and
is an integer and  is a positive integer. Moreover, these series converge when
is a positive integer. Moreover, these series converge when 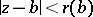 or
or 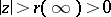 , respectively. The generalized elements
, respectively. The generalized elements 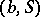 or, more precisely, their equivalence classes, form in their totality the analytic image
or, more precisely, their equivalence classes, form in their totality the analytic image 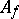 corresponding to the given analytic function
corresponding to the given analytic function 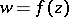 . Among the equivalence classes of elements
. Among the equivalence classes of elements 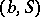 that form the analytic image one can distinguish regular ones, when
that form the analytic image one can distinguish regular ones, when 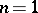 , and ramified ones, when
, and ramified ones, when 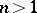 . The introduction of an appropriate topology on the analytic image
. The introduction of an appropriate topology on the analytic image 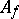 will turn it into the Riemann surface
will turn it into the Riemann surface 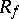 of the analytic function
of the analytic function 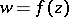 . This can be achieved, for example, by defining the neighbourhood of an element
. This can be achieved, for example, by defining the neighbourhood of an element 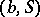 ,
,  , as the set consisting of the element
, as the set consisting of the element 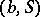 itself and all the regular elements
itself and all the regular elements  of
of 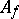 for which
for which 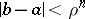 ,
, 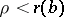 , and the series
, and the series 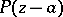 converges to one of the
converges to one of the  determinations of the series
determinations of the series 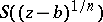 in their common domain of definition, i.e.
in their common domain of definition, i.e.
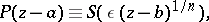 |
where  is one of the
is one of the  -th order roots of unity,
-th order roots of unity, 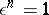 . The neighbourhood of the element
. The neighbourhood of the element 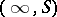 consists of the element
consists of the element 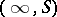 itself and all those regular elements
itself and all those regular elements 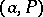 of
of  for which
for which 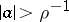 ,
, 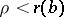 , and the series
, and the series 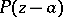 converges to one of the
converges to one of the  determinations of the series
determinations of the series 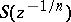 . The space
. The space 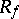 satisfies all the conditions of definition
satisfies all the conditions of definition 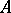 .
.
Thus, to any analytic function 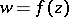 corresponds a Riemann surface
corresponds a Riemann surface  on which this function is a single-valued analytic function
on which this function is a single-valued analytic function 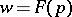 of a point
of a point 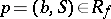 . This means that in a neighbourhood of any point
. This means that in a neighbourhood of any point 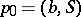 there exists a local uniformizing parameter
there exists a local uniformizing parameter 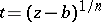 in which
in which 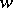 is represented as a single-valued analytic function
is represented as a single-valued analytic function  . In other words, the Riemann surface
. In other words, the Riemann surface 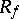 of an analytic function is a geometric construct that is used for the global uniformization of a, generally speaking, multiple-valued relation
of an analytic function is a geometric construct that is used for the global uniformization of a, generally speaking, multiple-valued relation 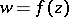 . In a neighbourhood of each point
. In a neighbourhood of each point 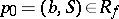 this relation is uniformized by the two single-valued analytic functions
this relation is uniformized by the two single-valued analytic functions 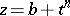 and
and 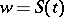 . On the other hand, the projection
. On the other hand, the projection 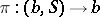 that takes each element
that takes each element 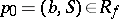 to its centre
to its centre 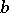 shows that the Riemann surface
shows that the Riemann surface 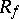 of an analytic function is a (ramified) covering surface over the extended complex plane
of an analytic function is a (ramified) covering surface over the extended complex plane 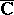 or, which is the same, over the Riemann sphere. The projections of the ramified elements
or, which is the same, over the Riemann sphere. The projections of the ramified elements 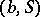 with
with 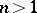 are branch points of this covering.
are branch points of this covering.
At the same time, to each a priori given Riemann surface 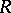 correspond infinitely-many analytic functions
correspond infinitely-many analytic functions 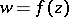 with precisely
with precisely 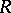 as Riemann surface,
as Riemann surface, 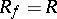 . For the case of closed Riemann surfaces this statement was formulated and proved already by Riemann in 1851. The central point of the corresponding proof is the construction on
. For the case of closed Riemann surfaces this statement was formulated and proved already by Riemann in 1851. The central point of the corresponding proof is the construction on 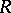 of harmonic functions with given singularities. The proof given by Riemann was based on an uncritical application of the so-called Dirichlet principle; Koebe (1909) was the first to give a rigorous proof; later there appeared simpler proofs of this fundamental statement, among them those based on the properly applied Dirichlet principle (see, e.g. [3], [4], [17], [18]).
of harmonic functions with given singularities. The proof given by Riemann was based on an uncritical application of the so-called Dirichlet principle; Koebe (1909) was the first to give a rigorous proof; later there appeared simpler proofs of this fundamental statement, among them those based on the properly applied Dirichlet principle (see, e.g. [3], [4], [17], [18]).
Whatever the orientable topological surface 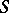 may be, one can construct a Riemann surface
may be, one can construct a Riemann surface 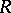 homeomorphic to
homeomorphic to 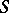 , i.e. a Riemann surface of the same topological type as
, i.e. a Riemann surface of the same topological type as 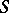 . Closed Riemann surfaces are topologically completely determined by one number — the genus
. Closed Riemann surfaces are topologically completely determined by one number — the genus 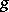 ,
, 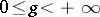 (cf. Genus of a surface). The topological type of such a Riemann surface
(cf. Genus of a surface). The topological type of such a Riemann surface  for
for 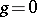 is a sphere, for
is a sphere, for 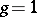 — a torus, for
— a torus, for  — a generalized torus, or a sphere with
— a generalized torus, or a sphere with 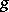 handles. By cutting a Riemann surface
handles. By cutting a Riemann surface 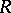 of genus
of genus  along some arc one obtains a digon with the symbol
along some arc one obtains a digon with the symbol  as its topological model or normal form, indicating that the points of the sides
as its topological model or normal form, indicating that the points of the sides  and
and 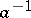 are identified; when
are identified; when 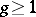 one has to carry out
one has to carry out 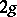 canonical sections
canonical sections 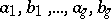 , after which one obtains the normal form of a closed Riemann surface
, after which one obtains the normal form of a closed Riemann surface 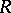 — a polygon with
— a polygon with  pairwise-identified sides; the symbol
pairwise-identified sides; the symbol 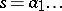 should indicate the order of appearance of the sides. For instance, in Fig. athe normal forms of a sphere for
should indicate the order of appearance of the sides. For instance, in Fig. athe normal forms of a sphere for  and a torus for
and a torus for 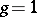 are shown together with their symbols.
are shown together with their symbols.
Figure: r082040a
From the analytic point of view a closed Riemann surface 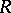 is characterized by the fact that it is the Riemann surface of some algebraic function
is characterized by the fact that it is the Riemann surface of some algebraic function 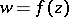 defined by an algebraic equation (1) of degree
defined by an algebraic equation (1) of degree 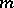 . This Riemann surface
. This Riemann surface 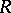 can be imagined as
can be imagined as  sheets extending over the Riemann sphere and mutually connected in a certain manner at the branch points and along some lines connecting these points (the manner of connecting is determined by the specific form of equation (1)). The genus
sheets extending over the Riemann sphere and mutually connected in a certain manner at the branch points and along some lines connecting these points (the manner of connecting is determined by the specific form of equation (1)). The genus 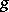 of the Riemann surface
of the Riemann surface 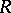 can in this case be expressed as a function of the number of sheets
can in this case be expressed as a function of the number of sheets 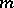 and the orders
and the orders 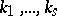 of the branch points by the Riemann–Hurwitz formula
of the branch points by the Riemann–Hurwitz formula
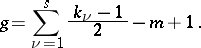 |
Finite Riemann surfaces 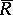 are topologically completely characterized by the genus
are topologically completely characterized by the genus 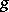 ,
, 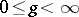 , and the number
, and the number  of connected components of the boundary; their topological type is a sphere with
of connected components of the boundary; their topological type is a sphere with 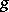 handles and
handles and 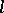 holes. In the normal form of a finite Riemann surface, the number of sides is not necessarily even, some sides corresponding to components of the boundary that remain free are not identified. The notion of the genus can also be generalized to open Riemann surfaces
holes. In the normal form of a finite Riemann surface, the number of sides is not necessarily even, some sides corresponding to components of the boundary that remain free are not identified. The notion of the genus can also be generalized to open Riemann surfaces 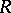 , for example by exhausting
, for example by exhausting 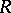 by a sequence
by a sequence 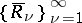 of compact Riemann surfaces with boundary
of compact Riemann surfaces with boundary 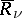 , belonging to
, belonging to 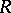 , and such that
, and such that 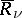 is contained in
is contained in 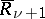 ,
, 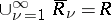 . The genus
. The genus 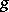 of
of 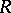 is set equal to
is set equal to 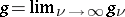 , where
, where 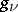 is the genus of
is the genus of 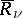 . This limit exists and is independent of the choice of the exhaustion
. This limit exists and is independent of the choice of the exhaustion 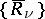 ,
, 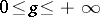 . However, the genus does not completely define the topological type of an open Riemann surface; the topological types of open Riemann surfaces can be rather diverse. Thus, in Fig.2a, Fig.2b two models with
. However, the genus does not completely define the topological type of an open Riemann surface; the topological types of open Riemann surfaces can be rather diverse. Thus, in Fig.2a, Fig.2b two models with 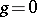 and
and 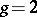 , respectively, are shown.
, respectively, are shown.
An important topological characteristic of a Riemann surface 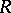 is the order of connectivity:
is the order of connectivity: 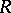 is called simply connected if any simple closed curve in
is called simply connected if any simple closed curve in  can be deformed continuously into a point without getting out of
can be deformed continuously into a point without getting out of 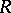 , i.e. in other words, if the fundamental group of
, i.e. in other words, if the fundamental group of 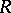 is trivial. Otherwise the Riemann surface
is trivial. Otherwise the Riemann surface 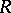 is called multiply connected. The schlichtartig Riemann surfaces form an important class of Riemann surfaces; they are the Riemann surfaces (with boundary or without) that are split by any simple closed curve into non-intersecting parts. For example, in Fig.2a a topological model of a multiply-connected schlichtartig Riemann surface is shown. A schlichtartig Riemann surface necessarily has genus zero. A schlichtartig Riemann surface
is called multiply connected. The schlichtartig Riemann surfaces form an important class of Riemann surfaces; they are the Riemann surfaces (with boundary or without) that are split by any simple closed curve into non-intersecting parts. For example, in Fig.2a a topological model of a multiply-connected schlichtartig Riemann surface is shown. A schlichtartig Riemann surface necessarily has genus zero. A schlichtartig Riemann surface 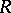 is called
is called  -connected if the minimum number of sections necessary to convert
-connected if the minimum number of sections necessary to convert  to a simply-connected Riemann surface is equal to
to a simply-connected Riemann surface is equal to 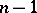 ,
, 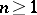 (see Fig.2b).
(see Fig.2b).
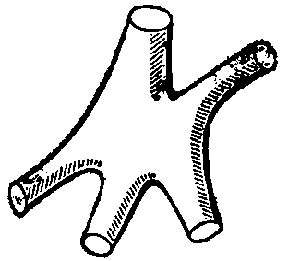
Figure: r082040b

Figure: r082040c
The topological properties of a Riemann surface 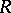 do not completely characterize the analytic properties of
do not completely characterize the analytic properties of 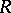 , i.e. the topological properties of
, i.e. the topological properties of 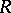 do not completely characterize the behaviour of functions of different classes on
do not completely characterize the behaviour of functions of different classes on 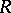 . In particular, let
. In particular, let 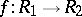 be a function on the Riemann surface
be a function on the Riemann surface 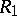 with values on another Riemann surface
with values on another Riemann surface 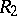 . The function
. The function 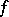 is called analytic on
is called analytic on 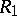 if for any point
if for any point 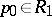 ,
, 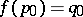 , one can find local uniformizing parameters
, one can find local uniformizing parameters 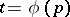 in a neighbourhood of
in a neighbourhood of 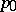 on
on 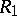 and
and 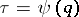 in a neighbourhood of
in a neighbourhood of  on
on 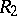 , respectively, such that the composite function
, respectively, such that the composite function
 |
is an analytic function of the complex variable 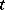 in a neighbourhood of the value
in a neighbourhood of the value 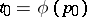 . Two Riemann surfaces
. Two Riemann surfaces 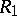 and
and 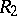 are called conformally equivalent, or are said to belong to the same conformal class (cf. Riemann surfaces, conformal classes of), if there exists an analytic function
are called conformally equivalent, or are said to belong to the same conformal class (cf. Riemann surfaces, conformal classes of), if there exists an analytic function 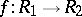 that gives a one-to-one mapping from
that gives a one-to-one mapping from 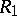 onto
onto 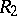 . From the point of view of the behaviour of analytic functions on Riemann surfaces, conformally-equivalent Riemann surfaces are considered as one and the same Riemann surface, but topologically-equivalent Riemann surfaces are not always conformally equivalent.
. From the point of view of the behaviour of analytic functions on Riemann surfaces, conformally-equivalent Riemann surfaces are considered as one and the same Riemann surface, but topologically-equivalent Riemann surfaces are not always conformally equivalent.
In terms of Riemann surfaces, the Riemann mapping theorem can be formulated as follows: Any simply-connected Riemann surface is conformally equivalent to one of the following three domains: 1) the extended complex plane 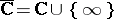 , i.e. the Riemann sphere (the elliptic case); 2) the finite complex plane
, i.e. the Riemann sphere (the elliptic case); 2) the finite complex plane 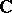 , i.e. the punctured Riemann sphere (the parabolic case); or 3) the unit disc
, i.e. the punctured Riemann sphere (the parabolic case); or 3) the unit disc 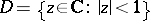 in
in 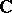 , i.e. the Riemann sphere with a section of positive length (the hyperbolic case). An important result is that any schlichtartig Riemann surface is conformally equivalent to some canonical domain in the extended complex plane. As such a canonical domain one may take the entire extended plane with a finite or infinite number of sections parallel to the real axis; moreover, some of these sections may degenerate into points. As mentioned above, in the case of a simply-connected Riemann surface the canonical domain either has no sections (elliptic type), or the section degenerates into a point (parabolic type), or the section has a positive length (hyperbolic type). All three types of simply-connected Riemann surfaces are conformally different, although the last two of them are topologically equivalent. The problem of types, which has not yet (1991) been solved completely, consists in finding additional conditions under which a simply-connected Riemann surface will be of hyperbolic or parabolic type (see [6], [7], [10], [11], and Riemann surfaces, classification of).
, i.e. the Riemann sphere with a section of positive length (the hyperbolic case). An important result is that any schlichtartig Riemann surface is conformally equivalent to some canonical domain in the extended complex plane. As such a canonical domain one may take the entire extended plane with a finite or infinite number of sections parallel to the real axis; moreover, some of these sections may degenerate into points. As mentioned above, in the case of a simply-connected Riemann surface the canonical domain either has no sections (elliptic type), or the section degenerates into a point (parabolic type), or the section has a positive length (hyperbolic type). All three types of simply-connected Riemann surfaces are conformally different, although the last two of them are topologically equivalent. The problem of types, which has not yet (1991) been solved completely, consists in finding additional conditions under which a simply-connected Riemann surface will be of hyperbolic or parabolic type (see [6], [7], [10], [11], and Riemann surfaces, classification of).
In the case of an arbitrary Riemann surface 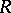 , its universal covering surface
, its universal covering surface 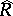 will always be a simply-connected Riemann surface, and thus belongs to one of the three types above. The Riemann surface
will always be a simply-connected Riemann surface, and thus belongs to one of the three types above. The Riemann surface 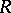 itself is considered to be of elliptic, parabolic or hyperbolic type, according to the type of its universal covering
itself is considered to be of elliptic, parabolic or hyperbolic type, according to the type of its universal covering 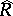 . This classification of Riemann surfaces is justified by the following considerations. Let
. This classification of Riemann surfaces is justified by the following considerations. Let  be one of three domains: the extended complex plane, the finite complex plane or the open unit disc, and let
be one of three domains: the extended complex plane, the finite complex plane or the open unit disc, and let  be some group of Möbius transformations of
be some group of Möbius transformations of 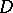 onto itself (automorphisms) without fixed points in
onto itself (automorphisms) without fixed points in 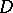 . A conformal mapping
. A conformal mapping 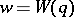 of the universal covering
of the universal covering 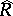 onto
onto  carries the group
carries the group 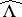 of transformations of the covering
of transformations of the covering 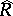 , which is isomorphic to the fundamental group
, which is isomorphic to the fundamental group 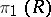 , onto some group
, onto some group  of automorphisms of
of automorphisms of 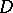 . Moreover,
. Moreover, 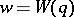 can be considered as a conformal mapping from the quotient space
can be considered as a conformal mapping from the quotient space 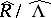 onto the quotient space
onto the quotient space 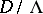 , and
, and  can be identified with
can be identified with 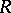 . Thus,
. Thus, 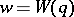 can be considered as a conformal mapping from the Riemann surface
can be considered as a conformal mapping from the Riemann surface  onto the quotient space
onto the quotient space 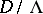 with some group of automorphisms
with some group of automorphisms 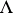 isomorphic to the fundamental group
isomorphic to the fundamental group 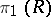 .
.
Since a Riemann surface 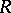 of elliptic type is necessarily simply-connected, the group
of elliptic type is necessarily simply-connected, the group 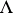 is trivial and thus such a Riemann surface is necessarily the Riemann surface of the function inverse to a rational function. A simply-connected Riemann surface of parabolic type is necessarily the Riemann surface of the function inverse to a meromorphic function in the finite plane. A compact Riemann surface of genus
is trivial and thus such a Riemann surface is necessarily the Riemann surface of the function inverse to a rational function. A simply-connected Riemann surface of parabolic type is necessarily the Riemann surface of the function inverse to a meromorphic function in the finite plane. A compact Riemann surface of genus 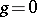 ,
, 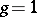 or
or 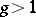 is a Riemann surface of elliptic, parabolic or hyperbolic type, respectively.
is a Riemann surface of elliptic, parabolic or hyperbolic type, respectively.
In connection with the conformal equivalence of Riemann surfaces there arises the question of the structure of the group 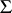 of conformal automorphisms of a Riemann surface
of conformal automorphisms of a Riemann surface 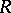 . Except for certain simple cases, this group
. Except for certain simple cases, this group 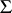 is discrete and for compact Riemann surfaces of genus
is discrete and for compact Riemann surfaces of genus 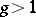 it is finite (Schwarz' theorem). There are only seven exceptional cases in which the group
it is finite (Schwarz' theorem). There are only seven exceptional cases in which the group 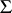 is continuous, namely (representatives of the corresponding conformal classes are given): the sphere in the elliptic case; the sphere with one or two punctures and the torus in the parabolic case; the open disc, the punctured open disc and an annulus in the hyperbolic case.
is continuous, namely (representatives of the corresponding conformal classes are given): the sphere in the elliptic case; the sphere with one or two punctures and the torus in the parabolic case; the open disc, the punctured open disc and an annulus in the hyperbolic case.
Of great importance also is the moduli problem for Riemann surfaces in its different versions (cf. Moduli of a Riemann surface; Moduli problem). It is the problem of the possible description of the diversity of conformally-inequivalent Riemann surfaces of different types. For example, it is easy to establish the following facts. The set of types of conformally-inequivalent doubly-connected schlichtartig Riemann surfaces (annuli) depends on one real parameter (the modulus) 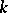 ,
, 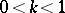 ; i.e. two annuli
; i.e. two annuli 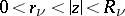 ,
, 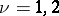 , are conformally equivalent if and only if the ratios of their radii
, are conformally equivalent if and only if the ratios of their radii 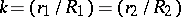 coincide. The set of types of conformally-inequivalent
coincide. The set of types of conformally-inequivalent  -connected schlichtartig Riemann surfaces for
-connected schlichtartig Riemann surfaces for 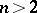 depends on
depends on  real parameters. The set of types of conformally-inequivalent closed Riemann surfaces of genus
real parameters. The set of types of conformally-inequivalent closed Riemann surfaces of genus 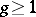 for
for 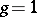 depends on two real parameters and for
depends on two real parameters and for 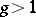 on
on 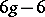 real parameters (see Riemann surfaces, conformal classes of, and also [3], [12], [13], , ; concerning the behaviour of functions of other classes on Riemann surfaces see Riemann surfaces, classification of).
real parameters (see Riemann surfaces, conformal classes of, and also [3], [12], [13], , ; concerning the behaviour of functions of other classes on Riemann surfaces see Riemann surfaces, classification of).
An important aspect of the theory of Riemann surfaces is its connection with the concept of uniformization. In general, for a multiple-valued analytic function
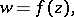 | (2) |
its Riemann surface 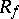 provides a geometrical means of uniformization: The multiple-valued relation (2) is replaced by two single-valued relations
provides a geometrical means of uniformization: The multiple-valued relation (2) is replaced by two single-valued relations
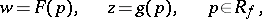 | (3) |
which give a single-valued expression of  and
and 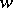 in the entire domain of definition of the function (2) as a complete analytic function. On the other hand, the approach of K. Weierstrass to the construction of the notion of the complete analytic function of (2) is based on the use of a local uniformizing parameter
in the entire domain of definition of the function (2) as a complete analytic function. On the other hand, the approach of K. Weierstrass to the construction of the notion of the complete analytic function of (2) is based on the use of a local uniformizing parameter  that allows one to express the variables
that allows one to express the variables  and
and 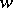 analytically as single-valued analytic functions
analytically as single-valued analytic functions 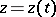 and
and 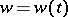 , locally in a neighbourhood of some point
, locally in a neighbourhood of some point 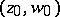 ,
, 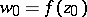 . The uniformization problem in its simplest classical form is the problem of synthesis of these two ideas. One has to replace the relation (2) in its entire domain of definition by two analytic representations
. The uniformization problem in its simplest classical form is the problem of synthesis of these two ideas. One has to replace the relation (2) in its entire domain of definition by two analytic representations 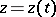 ,
, 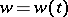 , where
, where 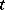 is a uniformizing complex variable with values in some domain of the plane.
is a uniformizing complex variable with values in some domain of the plane.
The above-mentioned statement of the possibility of uniformization was established by Koebe and, independently, by Poincaré almost simultaneously in 1907. If the Riemann surface 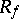 of the function (2) is simply connected or schlichtartig, then the uniformization problem is reduced to constructing a conformal mapping
of the function (2) is simply connected or schlichtartig, then the uniformization problem is reduced to constructing a conformal mapping 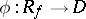 from
from  onto the planar domain
onto the planar domain 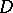 . The representations (3) then provide the sought uniformization:
. The representations (3) then provide the sought uniformization:
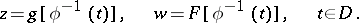 |
A conformal mapping 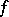 onto a planar domain exists only for the schlichtartig Riemann surfaces
onto a planar domain exists only for the schlichtartig Riemann surfaces 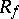 (the general uniformization theorem).
(the general uniformization theorem).
In the general case of an arbitrary analytic relation (2), the Riemann surface 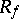 is not schlichtartig but its universal covering surface
is not schlichtartig but its universal covering surface 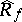 is simply connected and, hence, there exists a conformal mapping
is simply connected and, hence, there exists a conformal mapping
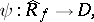 |
where 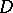 is one of the already-mentioned domains:
is one of the already-mentioned domains: 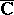 ,
, 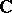 or the open unit disc. The function
or the open unit disc. The function 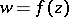 is meromorphic on the Riemann surface
is meromorphic on the Riemann surface 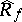 and, hence, it is also meromorphic on
and, hence, it is also meromorphic on 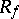 ; moreover, it depends only on the projection
; moreover, it depends only on the projection 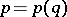 ,
,  , of a point
, of a point 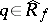 . Thus one obtains a geometrical uniformization in the form
. Thus one obtains a geometrical uniformization in the form
 |
and from it the analytic uniformization
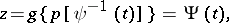 |
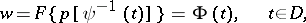 |
where  and
and 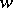 are expressed as meromorphic functions
are expressed as meromorphic functions 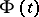 and
and 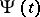 of a variable
of a variable 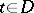 . These functions
. These functions 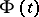 and
and 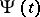 are automorphic functions (cf. Automorphic function) in
are automorphic functions (cf. Automorphic function) in 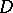 relative to the group of automorphisms
relative to the group of automorphisms 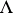 isomorphic to the fundamental group
isomorphic to the fundamental group 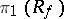 of the Riemann surface
of the Riemann surface 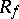 of the uniformizing function (see [3], [7], , ).
of the uniformizing function (see [3], [7], , ).
References
| [1] | B. Riemann, "Collected works" , Dover, reprint (1953) |
| [2] | A.I. Markushevich, "Theory of functions of a complex variable" , 1–2 , Chelsea (1977) (Translated from Russian) |
| [3] | A. Hurwitz, R. Courant, "Vorlesungen über allgemeine Funktionentheorie und elliptische Funktionen" , Springer (1964) |
| [4] | S. Stoilov, "The theory of functions of a complex variable" , 1–2 , Moscow (1962) (In Russian; translated from Rumanian) |
| [5] | S. [S. Stoilov] Stoilow, "Leçons sur les principes topologiques de la théorie des fonctions analytiques" , Gauthier-Villars (1938) |
| [6] | G. Springer, "Introduction to Riemann surfaces" , Chelsea, reprint (1981) |
| [7] | R. Nevanlinna, "Uniformisierung" , Springer (1967) |
| [8] | M. Schiffer, D.C. Spencer, "Functionals of finite Riemann surfaces" , Princeton Univ. Press (1954) |
| [9] | N.G. Chebotarev, "The theory of algebraic functions" , Moscow-Leningrad (1948) (In Russian) |
| [10] | L.I. Volkovyskii, "Investigation of the type problem for a simply-connected Riemann surface" Trudy Mat. Inst. Steklov. , 34 (1950) pp. 3–171 (In Russian) |
| [11] | L.I. Volkovyskii, "Contempory studies on Riemann surfaces" Uspekhi Mat. Nauk , 11 : 5 (1956) pp. 77–84 (In Russian) |
| [12] | S.L. Krushkal', "Quasi-conformal mappings and Riemann surfaces" , Winston & Wiley (1979) (Translated from Russian) |
| [13] | S.L. Krushkal', B.N. Apanasov, N.A. Gusevskii, "Kleinian groups and uniformization in examples and problems" , Amer. Math. Soc. (1986) (Translated from Russian) |
| [14] | E.B. Vinberg, O.V. Shvartsman, "Riemann surfaces" J. Soviet Math. , 14 : 1 (1980) pp. 985–1020 Itogi Nauk. i Tekhn. Algebra. Topol. Geom. , 16 (1978) pp. 191–245 |
| [15a] | L. Bers, "Quasi-conformal mappings and Teichmüller's theorem" R. Nevanlinna (ed.) et al. (ed.) , Analytic functions , Princeton Univ. Press (1960) pp. 89–119 |
| [15b] | L.V. Ahlfors, "The complex analytic structure of the space of closed Riemann surfaces" R. Nevanlinna (ed.) et al. (ed.) , Analytic functions , Princeton Univ. Press (1960) pp. 45–66 |
| [15c] | L. Bers, "Spaces of Riemann surfaces" J.A. Todd (ed.) , Proc. Internat. Congress Mathematicians (Edinburgh, 1958) , Cambridge Univ. Press (1960) pp. 349–361 |
| [15d] | L. Bers, "Simultaneous uniformization" Bull. Amer. Math. Soc. , 66 (1960) pp. 94–97 |
| [15e] | L. Bers, "Holomorphic differentials as functions of moduli" Bull. Amer. Math. Soc. , 67 (1961) pp. 206–210 |
| [15f] | L. Ahlfors, "On quasiconformal mappings" J. d'Anal. Math. , 3 (1954) pp. 1–58; 207–208 |
| [16a] | L. Bers, "Uniformization, moduli, and Kleinian groups" Bull. London Math. Soc. , 4 (1972) pp. 257–300 |
| [16b] | L. Bers, "The moduli of Kleinian groups" Russian Math. Surveys , 29 : 2 (1974) pp. 88–102 Uspekhi Mat. Nauk , 29 : 2 (1974) pp. 86–102 |
| [17] | F. Klein, "Riemannschen Flächen" , Springer, reprint (1986) |
| [18] | H. Weyl, "The concept of a Riemann surface" , Addison-Wesley (1955) (Translated from German) |
| [19] | L.V. Ahlfors, L. Sario, "Riemann surfaces" , Princeton Univ. Press (1974) |
| [20] | A. Pfluger, "Theorie der Riemannschen Flächen" , Springer (1957) |
| [21] | L. Sario, M. Nakai, "Classification theory of Riemann surfaces" , Springer (1970) |
| [22] | M. Heins, "Hardy classes on Riemann surfaces" , Springer (1969) |
| [23] | R.C. Gunning, "Lectures on Riemann surfaces" , Princeton Univ. Press (1966) |
| [24] | R.C. Gunning, "Lectures on Riemann surfaces: Jacobi varieties" , Princeton Univ. Press (1972) |
| [25] | O. Forster, "Lectures on Riemann surfaces" , Springer (1981) (Translated from German) |
Comments
For the generalization to "Riemann surfaces over Cn" see, e.g., [a6] and Riemannian domain.
References
| [a1] | P.A. Griffiths, J.E. Harris, "Principles of algebraic geometry" , Wiley (Interscience) (1978) |
| [a2] | P. Griffiths, "Introduction to algebraic curves" , Amer. Math. Soc. (1989) |
| [a3] | H.M. Farkas, I. Kra, "Riemann surfaces" , Springer (1980) pp. Sect. III.6 |
| [a4] | H. Behnke, F. Sommer, "Theorie der analytischen Funktionen einer komplexen Veränderlichen" , Springer (1955) |
| [a5] | H. Cohn, "Conformal mapping on Riemann surfaces" , Dover, reprint (1980) |
| [a6] | H. Behnke, P. Thullen, "Theorie der Funktionen meherer komplexer Veränderlichen" , Springer (1970) pp. Chapt. VI (Elraged & Revised Edition. Original: 1934) |
| [a7] | W. Osgood, "Lehrbuch der Funktionentheorie" , 1–2 , Chelsea, reprint (1965) |
Riemann surface. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemann_surface&oldid=18777