Rees semi-group of matrix type

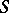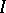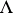A semi-group theoretical construction defined as follows. Let be an arbitrary semi-group, let and be (index) sets and let 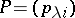be a -matrix over 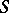, i.e. a mapping from the Cartesian product 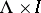into 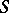. The following formula defines an operation on the set :

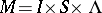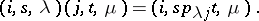Then 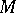is a semi-group, called a Rees semi-group of matrix type over 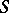and denoted by 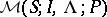; the matrix 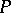is called the sandwich matrix of . If 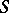is a semi-group with zero 0, then 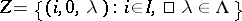is an ideal in 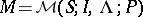and the Rees quotient semi-group 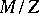(see Semi-group) is denoted by 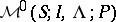; in the case when is a group 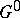with an adjoined zero, instead of 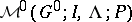one writes 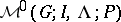and calls it a Rees semi-group of matrix type over the group 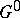with an adjoined zero. The group 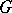is called the structure group for the semi-groups 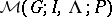and .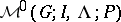

Another representation of the Rees semi-group of matrix type over a semi-group 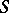with zero and 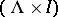-sandwich matrix 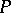is realized in the following way. An 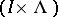-matrix over 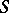is called a Rees matrix if it does not contain more than one non-zero element. Let 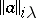be the Rees matrix over 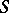that has in the -th row and 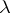-th column, and zeros in all other places. On the set of all 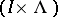-Rees matrices over 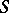one can define an operation

(1)

where on the right-hand side is the "ordinary" matrix product. This set becomes a semi-group with respect to this operation. The mapping 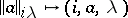is an isomorphism between this semi-group and the semi-group 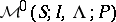; the notation 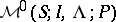is used for both of these semi-groups. Formula (1) provides an explanation of the term "sandwich matrix" for 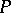. If 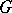is a group, then the semi-group 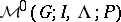is regular if and only if each row and each column of the matrix 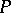contains a non-zero element; any semi-group 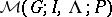is completely simple (cf. Completely-simple semi-group), any regular semi-group 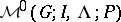is completely -simple. The converse of the last two statements gives the main content of Rees's theorem [1]: Any completely-simple (completely -simple) semi-group can be isomorphically represented as a Rees semi-group of matrix type over a group (as a regular Rees semi-group of matrix type over a group with an adjoined zero). 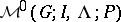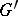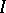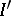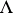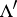If and are isomorphic, then the groups and are isomorphic, and have the same cardinality, and and have the same cardinality. Necessary and sufficient conditions for isomorphy of the semi-groups 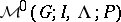and 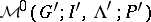are known, and together with the just-mentioned conditions they include a quite definite relation between the sandwich matrices 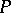and 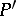(see [1]–[3]). In particular, any completely -simple semi-group can be isomorphically represented as a Rees semi-group of matrix type in whose sandwich matrix each element in a given row and a given column is either 0 or the identity element of the structure group; such a sandwich matrix is called normalized. Similar properties are valid for completely-simple semi-groups.

References

[1]	D. Rees, "On semi-groups" Proc. Cambridge Philos. Soc. , 36 (1940) pp. 387–400
[2]	A.H. Clifford, G.B. Preston, "Algebraic theory of semi-groups" , 1–2 , Amer. Math. Soc. (1961–1967)
[3]	E.S. Lyapin, "Semigroups" , Amer. Math. Soc. (1974) (Translated from Russian)