Difference between revisions of "Probability integral"
(Importing text file) |
(OldImage template added) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | p0749201.png | ||
| + | $#A+1 = 28 n = 0 | ||
| + | $#C+1 = 28 : ~/encyclopedia/old_files/data/P074/P.0704920 Probability integral, | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
''error integral'' | ''error integral'' | ||
The function | The function | ||
| − | + | $$ | |
| + | \mathop{\rm erf} ( x) = \ | ||
| + | |||
| + | \frac{2}{\sqrt \pi } | ||
| + | |||
| + | \int\limits _ { 0 } ^ { x } e ^ {- t ^ {2} } d t ,\ \ | ||
| + | | x | < \infty . | ||
| + | $$ | ||
In probability theory one mostly encounters not the probability integral, but the [[Normal distribution|normal distribution]] function | In probability theory one mostly encounters not the probability integral, but the [[Normal distribution|normal distribution]] function | ||
| − | + | $$ | |
| + | \Phi ( x) = \ | ||
| − | + | \frac{1}{\sqrt {2 \pi } } | |
| − | + | \int\limits _ {- \infty } ^ { x } e ^ {- t ^ {2} / 2 } d t = | |
| + | \frac{1}{2} | ||
| + | |||
| + | \left [ 1 + \mathop{\rm erf} \left ( | ||
| + | \frac{x}{\sqrt 2 } | ||
| + | \right ) \right ] , | ||
| + | $$ | ||
| + | |||
| + | which is the so-called Gaussian probability integral. For a random variable $ X $ | ||
| + | having the normal distribution with mathematical expectation 0 and variance $ \sigma ^ {2} $, | ||
| + | the probability that $ | X | \leq t $ | ||
| + | is equal to $ \mathop{\rm erf} ( t / \sqrt 2 ) $. | ||
| + | For real $ x $, | ||
| + | the probability integral takes real values; in particular, | ||
| + | |||
| + | $$ | ||
| + | \mathop{\rm erf} ( 0) = 0 ,\ \ | ||
| + | \lim\limits _ {x \rightarrow + \infty } \mathop{\rm erf} ( x) = 1 . | ||
| + | $$ | ||
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/p074920a.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/p074920a.gif" /> | ||
| Line 17: | Line 55: | ||
Figure: p074920a | Figure: p074920a | ||
| − | The graph of the probability integral and its derivatives are illustrated in the figure. Regarded as a function of the complex variable | + | The graph of the probability integral and its derivatives are illustrated in the figure. Regarded as a function of the complex variable $ z $, |
| + | the probability integral $ \mathop{\rm erf} ( z) $ | ||
| + | is an entire function of $ z $. | ||
| − | The asymptotic representation for large | + | The asymptotic representation for large $ z $, |
| + | $ \mathop{\rm Re} z > 0 $, | ||
| + | is given by: | ||
| − | + | $$ | |
| + | 1 - \mathop{\rm erf} ( z) \sim \ | ||
| − | + | \frac{e ^ {- z ^ {2} } }{\sqrt {\pi z } } | |
| − | + | \left ( | |
| + | 1 + \sum_{k=1} ^ \infty ( - 1 ) ^ {k} | ||
| − | + | \frac{1 \cdot 3 \dots ( 2 k - 1 ) }{2 ^ {k} } | |
| − | + | \frac{1}{z ^ {2k} } | |
| − | + | \right ) . | |
| + | $$ | ||
| + | |||
| + | In a neighbourhood of $ z = 0 $ | ||
| + | the probability integral can be represented by the series | ||
| + | |||
| + | $$ | ||
| + | \mathop{\rm erf} ( z) = \ | ||
| + | |||
| + | \frac{2}{\sqrt \pi } | ||
| + | |||
| + | \left ( | ||
| + | z - | ||
| + | \frac{z ^ {3} }{1!3} | ||
| + | |||
| + | + \dots + | ||
| + | \frac{( - 1 ) ^ {k} }{k ! ( 2 k + 1 ) } | ||
| + | |||
| + | z ^ {2k+1} + \dots | ||
| + | \right ) . | ||
| + | $$ | ||
| + | |||
| + | The probability integral is related to the [[Fresnel integrals|Fresnel integrals]] $ C ( z) $ | ||
| + | and $ S ( z) $ | ||
| + | by the formulas | ||
| + | |||
| + | $$ | ||
| + | 1+ | ||
| + | \frac{i}{2} | ||
| + | \ | ||
| + | \mathop{\rm erf} \left ( 1- | ||
| + | \frac{i}{\sqrt 2} | ||
| + | z \right ) = \ | ||
| + | C ( z) + i S ( z) , | ||
| + | $$ | ||
| + | |||
| + | $$ | ||
| + | 1- | ||
| + | \frac{i}{2} | ||
| + | \mathop{\rm erf} \left ( 1+ | ||
| + | \frac{i}{\sqrt 2} | ||
| + | z \right ) = C ( z) - i S ( z) . | ||
| + | $$ | ||
The derivative of the probability integral is given by: | The derivative of the probability integral is given by: | ||
| − | + | $$ | |
| + | [ \mathop{\rm erf} ( z) ] ^ \prime = \ | ||
| + | |||
| + | \frac{2}{\sqrt \pi} | ||
| + | |||
| + | e ^ {- z ^ {2} } . | ||
| + | $$ | ||
The following notations are sometimes used: | The following notations are sometimes used: | ||
| − | + | $$ | |
| + | \Theta ( x) = H ( x) = \ | ||
| + | \Phi ( x) = \mathop{\rm erf} ( x) , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \mathop{\rm Erf} ( x) = | ||
| + | \frac{\sqrt \pi }{2} | ||
| + | \mathop{\rm erf} ( x) , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \mathop{\rm Erfi} ( x) = - i | ||
| + | \frac{\sqrt \pi }{2} | ||
| + | \mathop{\rm erf} ( i x | ||
| + | ) = \int\limits _ { 0 } ^ { x } e ^ {t ^ {2} } d t , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \mathop{\rm Erfc} ( x) = | ||
| + | \frac{\sqrt \pi }{2} | ||
| + | - \mathop{\rm Erf} x = \int\limits | ||
| + | _ { x } ^ \infty e ^ {- t ^ {2} } d t , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \alpha ( x) = | ||
| + | \frac{2}{\sqrt \pi} | ||
| + | \int\limits _ {- \infty } ^ { x } e ^ | ||
| + | {- t ^ {2} } d t - 1 = | ||
| + | \frac{2} \pi | ||
| + | \mathop{\rm Erf} \left ( | ||
| + | \frac{x}{\sqrt 2} | ||
| + | \right ) . | ||
| + | $$ | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , ''Higher transcendental functions'' , '''2. Bessel functions, parabolic cylinder functions, orthogonal polynomials''' , McGraw-Hill (1953)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , ''Higher transcendental functions'' , '''2. Bessel functions, parabolic cylinder functions, orthogonal polynomials''' , McGraw-Hill (1953)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian)</TD></TR></table> | ||
| + | ====Comments==== | ||
| + | The series representation of the probability integral around $ z= 0 $ | ||
| + | takes the form of a [[confluent hypergeometric function]]: | ||
| + | $$ | ||
| + | \mathop{\rm erf} ( z)= | ||
| + | \frac{2}{\sqrt \pi } | ||
| + | z \Phi ( 1/2; 3/2; - z ^ {2} ) . | ||
| + | $$ | ||
| − | + | {{OldImage}} | |
| − | |||
| − | |||
| − | |||
Latest revision as of 19:23, 11 January 2024
error integral
The function
$$ \mathop{\rm erf} ( x) = \ \frac{2}{\sqrt \pi } \int\limits _ { 0 } ^ { x } e ^ {- t ^ {2} } d t ,\ \ | x | < \infty . $$
In probability theory one mostly encounters not the probability integral, but the normal distribution function
$$ \Phi ( x) = \ \frac{1}{\sqrt {2 \pi } } \int\limits _ {- \infty } ^ { x } e ^ {- t ^ {2} / 2 } d t = \frac{1}{2} \left [ 1 + \mathop{\rm erf} \left ( \frac{x}{\sqrt 2 } \right ) \right ] , $$
which is the so-called Gaussian probability integral. For a random variable $ X $ having the normal distribution with mathematical expectation 0 and variance $ \sigma ^ {2} $, the probability that $ | X | \leq t $ is equal to $ \mathop{\rm erf} ( t / \sqrt 2 ) $. For real $ x $, the probability integral takes real values; in particular,
$$ \mathop{\rm erf} ( 0) = 0 ,\ \ \lim\limits _ {x \rightarrow + \infty } \mathop{\rm erf} ( x) = 1 . $$
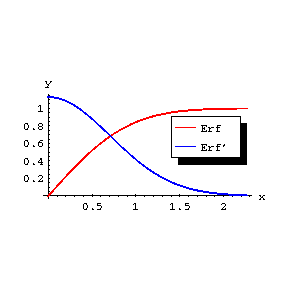
Figure: p074920a
The graph of the probability integral and its derivatives are illustrated in the figure. Regarded as a function of the complex variable $ z $, the probability integral $ \mathop{\rm erf} ( z) $ is an entire function of $ z $.
The asymptotic representation for large $ z $, $ \mathop{\rm Re} z > 0 $, is given by:
$$ 1 - \mathop{\rm erf} ( z) \sim \ \frac{e ^ {- z ^ {2} } }{\sqrt {\pi z } } \left ( 1 + \sum_{k=1} ^ \infty ( - 1 ) ^ {k} \frac{1 \cdot 3 \dots ( 2 k - 1 ) }{2 ^ {k} } \frac{1}{z ^ {2k} } \right ) . $$
In a neighbourhood of $ z = 0 $ the probability integral can be represented by the series
$$ \mathop{\rm erf} ( z) = \ \frac{2}{\sqrt \pi } \left ( z - \frac{z ^ {3} }{1!3} + \dots + \frac{( - 1 ) ^ {k} }{k ! ( 2 k + 1 ) } z ^ {2k+1} + \dots \right ) . $$
The probability integral is related to the Fresnel integrals $ C ( z) $ and $ S ( z) $ by the formulas
$$ 1+ \frac{i}{2} \ \mathop{\rm erf} \left ( 1- \frac{i}{\sqrt 2} z \right ) = \ C ( z) + i S ( z) , $$
$$ 1- \frac{i}{2} \mathop{\rm erf} \left ( 1+ \frac{i}{\sqrt 2} z \right ) = C ( z) - i S ( z) . $$
The derivative of the probability integral is given by:
$$ [ \mathop{\rm erf} ( z) ] ^ \prime = \ \frac{2}{\sqrt \pi} e ^ {- z ^ {2} } . $$
The following notations are sometimes used:
$$ \Theta ( x) = H ( x) = \ \Phi ( x) = \mathop{\rm erf} ( x) , $$
$$ \mathop{\rm Erf} ( x) = \frac{\sqrt \pi }{2} \mathop{\rm erf} ( x) , $$
$$ \mathop{\rm Erfi} ( x) = - i \frac{\sqrt \pi }{2} \mathop{\rm erf} ( i x ) = \int\limits _ { 0 } ^ { x } e ^ {t ^ {2} } d t , $$
$$ \mathop{\rm Erfc} ( x) = \frac{\sqrt \pi }{2} - \mathop{\rm Erf} x = \int\limits _ { x } ^ \infty e ^ {- t ^ {2} } d t , $$
$$ \alpha ( x) = \frac{2}{\sqrt \pi} \int\limits _ {- \infty } ^ { x } e ^ {- t ^ {2} } d t - 1 = \frac{2} \pi \mathop{\rm Erf} \left ( \frac{x}{\sqrt 2} \right ) . $$
References
| [1] | H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , Higher transcendental functions , 2. Bessel functions, parabolic cylinder functions, orthogonal polynomials , McGraw-Hill (1953) |
| [2] | E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German) |
| [3] | A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960) |
| [4] | N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian) |
Comments
The series representation of the probability integral around $ z= 0 $ takes the form of a confluent hypergeometric function:
$$ \mathop{\rm erf} ( z)= \frac{2}{\sqrt \pi } z \Phi ( 1/2; 3/2; - z ^ {2} ) . $$
Probability integral. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Probability_integral&oldid=18624