Difference between revisions of "Plane trigonometry"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
Ulf Rehmann (talk | contribs) m (Undo revision 48186 by Ulf Rehmann (talk)) Tag: Undo |
||
| Line 1: | Line 1: | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
''trigonometry in the Euclidean plane.'' | ''trigonometry in the Euclidean plane.'' | ||
| − | The elements of a triangle, its sides | + | The elements of a triangle, its sides <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728101.png" /> and its angles <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728102.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728103.png" /> opposite to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728104.png" />, etc.), are related in various ways. In the Euclidean plane the most important relations are the angle sum formula |
| − | and its angles | ||
| − | |||
| − | opposite to | ||
| − | etc.), are related in various ways. In the Euclidean plane the most important relations are the angle sum formula | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728105.png" /></td> </tr></table> | |
| − | |||
| − | |||
(angles in radians), and the triangle inequalities | (angles in radians), and the triangle inequalities | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728106.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | These inequalities are necessary and sufficient for three segments of positive length | + | These inequalities are necessary and sufficient for three segments of positive length <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728107.png" /> to form the sides of a triangle. |
| − | to form the sides of a triangle. | ||
Another relation is the [[Cosine theorem|cosine theorem]]: | Another relation is the [[Cosine theorem|cosine theorem]]: | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728108.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | In particular, when | + | In particular, when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p0728109.png" />, the triangle is right-angled, and the cosine theorem becomes Pythagoras' theorem (cf. [[Pythagoras theorem|Pythagoras theorem]]) |
| − | the triangle is right-angled, and the cosine theorem becomes Pythagoras' theorem (cf. [[Pythagoras theorem|Pythagoras theorem]]) | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281010.png" /></td> </tr></table> | |
| − | |||
| − | |||
In such a right-angled triangle, | In such a right-angled triangle, | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281011.png" /></td> </tr></table> | |
In a general triangle, further relations are provided by the [[Sine theorem|sine theorem]]: | In a general triangle, further relations are provided by the [[Sine theorem|sine theorem]]: | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281012.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281013.png" /> is the radius of the circumcircle of the triangle (cf. [[Inscribed and circumscribed figures|Inscribed and circumscribed figures]]). A corollary of the sine theorem is the tangent formula | |
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281014.png" /></td> </tr></table> | |
| − | |||
| − | + | With the notation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281015.png" /> for the semi-perimeter of the triangle, the following half-angle formulas follow from the cosine theorem: | |
| − | |||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281016.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281017.png" /></td> </tr></table> | |
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281018.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==Geometry of the triangle.== | ==Geometry of the triangle.== | ||
| − | Among the many remarkable lines, points and circles connected with any triangle are the circumcircle with centre 0 and radius | + | Among the many remarkable lines, points and circles connected with any triangle are the circumcircle with centre 0 and radius <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281019.png" />, the incircle and the three excircles with centres <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281020.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281021.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281022.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281023.png" /> and radii <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281024.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281025.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281026.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281027.png" /> (cf. [[Inscribed and circumscribed figures|Inscribed and circumscribed figures]]), the medians (cf. [[Median (of a triangle)|Median (of a triangle)]]) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281028.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281029.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281030.png" />, with the centroid <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281031.png" /> as their common point, the inner bisectors <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281032.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281033.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281034.png" />, and the outer bisectors <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281035.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281036.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281037.png" />, the altitude lines <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281038.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281039.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281040.png" /> with the [[Orthocentre|orthocentre]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281041.png" /> as their common point, the Euler line (cf. [[Euler straight line|Euler straight line]]) through <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281042.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281043.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281044.png" />, and the [[Nine-point circle|nine-point circle]] through the midpoints of the sides, the feet of the altitude lines, and the midpoints of the segments connecting the vertices of the triangle to its orthocentre. The nine-point circle has radius <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281045.png" />, its centre <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281046.png" /> is on the Euler line between <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281047.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281048.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281049.png" />, and the nine-point circle touches the incircle and the three excircles (Feuerbach's theorem). |
| − | the incircle and the three excircles with centres | ||
| − | |||
| − | |||
| − | |||
| − | and radii | ||
| − | |||
| − | |||
| − | |||
| − | cf. [[Inscribed and circumscribed figures|Inscribed and circumscribed figures]]), the medians (cf. [[Median (of a triangle)|Median (of a triangle)]]) | ||
| − | |||
| − | |||
| − | with the centroid | ||
| − | as their common point, the inner bisectors | ||
| − | |||
| − | |||
| − | and the outer bisectors | ||
| − | |||
| − | |||
| − | the altitude lines | ||
| − | |||
| − | |||
| − | with the [[Orthocentre|orthocentre]] | ||
| − | as their common point, the Euler line (cf. [[Euler straight line|Euler straight line]]) through | ||
| − | |||
| − | and | ||
| − | and the [[Nine-point circle|nine-point circle]] through the midpoints of the sides, the feet of the altitude lines, and the midpoints of the segments connecting the vertices of the triangle to its orthocentre. The nine-point circle has radius | ||
| − | its centre | ||
| − | is on the Euler line between | ||
| − | and | ||
| − | such that | ||
| − | and the nine-point circle touches the incircle and the three excircles (Feuerbach's theorem). | ||
| − | With the notation | + | With the notation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281050.png" /> for the area of the triangle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281051.png" />, the following relations are valid: |
| − | for the area of the triangle | ||
| − | the following relations are valid: | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281052.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281053.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | = | ||
| − | |||
| − | |||
It follows that, among others, | It follows that, among others, | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281054.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Very remarkable is Morley's theorem: The points of intersection of the adjacent trisectors of the angles of any triangle form the vertices of an equilateral triangle. Indeed, a direct calculation shows that the sides of Morley's triangle have length | Very remarkable is Morley's theorem: The points of intersection of the adjacent trisectors of the angles of any triangle form the vertices of an equilateral triangle. Indeed, a direct calculation shows that the sides of Morley's triangle have length | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281055.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | which is symmetric in | + | which is symmetric in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281056.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281057.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281058.png" />. |
| − | |||
| − | and | ||
==The theorems of Ceva and Menelaus.== | ==The theorems of Ceva and Menelaus.== | ||
| − | Let | + | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281059.png" /> be points on the (possibly extended) sides <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281060.png" /> of a triangle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281061.png" />. Then, by the [[Ceva theorem|Ceva theorem]], <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281062.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281063.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281064.png" /> are congruent if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281065.png" /> (signed distances) and by the [[Menelaus theorem|Menelaus theorem]], <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281066.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281067.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281068.png" /> are collinear if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281069.png" />. |
| − | be points on the (possibly extended) sides | ||
| − | of a triangle | ||
| − | Then, by the [[Ceva theorem|Ceva theorem]], | ||
| − | |||
| − | and | ||
| − | are congruent if and only if | ||
| − | signed distances) and by the [[Menelaus theorem|Menelaus theorem]], | ||
| − | |||
| − | and | ||
| − | are collinear if and only if | ||
==Convex quadrangles.== | ==Convex quadrangles.== | ||
| − | Ptolemy's theorem (cf. [[Ptolemeus theorem|Ptolemeus theorem]]): For any point | + | Ptolemy's theorem (cf. [[Ptolemeus theorem|Ptolemeus theorem]]): For any point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281070.png" /> in the plane of a triangle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281071.png" /> the inequality |
| − | in the plane of a triangle | ||
| − | the inequality | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281072.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | holds, with equality if and only if | + | holds, with equality if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281073.png" /> is on the arc <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281074.png" /> of the circumcircle of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281075.png" /> (in this last case, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281076.png" /> is a circle quadrangle). |
| − | is on the arc | ||
| − | of the circumcircle of | ||
| − | in this last case, | ||
| − | is a circle quadrangle). | ||
| − | Brahmagupta's formula states that for any convex cyclic quadrangle | + | Brahmagupta's formula states that for any convex cyclic quadrangle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281077.png" /> with area <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281078.png" />, sides <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281079.png" /> and semi-perimeter <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281080.png" />, the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281081.png" /> holds. In general, for any quadrangle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281082.png" />, the area <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281083.png" /> satisfies |
| − | with area | ||
| − | sides | ||
| − | and semi-perimeter | ||
| − | the relation | ||
| − | holds. In general, for any quadrangle | ||
| − | the area | ||
| − | satisfies | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281084.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
It follows that among all quadrangles with given side lengths the inscribed quadrangles have maximum area (the cyclic order of the sides is immaterial). | It follows that among all quadrangles with given side lengths the inscribed quadrangles have maximum area (the cyclic order of the sides is immaterial). | ||
| − | ==Regular | + | ==Regular <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281085.png" />-gons.== |
| − | A regular | + | A regular <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281086.png" />-gon inscribed in a circle with radius <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281087.png" /> has perimeter <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281088.png" /> and area <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281089.png" />; a regular <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281090.png" />-gon circumscribed about a circle with radius <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281091.png" /> has perimeter <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281092.png" /> and area <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/p/p072/p072810/p07281093.png" />. See also [[Regular polyhedra|Regular polyhedra]]. |
| − | gon inscribed in a circle with radius | ||
| − | has perimeter | ||
| − | and area | ||
| − | a regular | ||
| − | gon circumscribed about a circle with radius | ||
| − | has perimeter | ||
| − | and area | ||
| − | See also [[Regular polyhedra|Regular polyhedra]]. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> H.S.M. Coxeter, "Introduction to geometry" , Wiley (1969) pp. 3–23</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> H.S.M. Coxeter, S.L. Greitzer, "Geometry revisited" , Random House (1967)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> M. Berger, "Geometry" , '''II''' , Springer (1987) pp. Chapt. 10</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> H.S.M. Coxeter, "Introduction to geometry" , Wiley (1969) pp. 3–23</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> H.S.M. Coxeter, S.L. Greitzer, "Geometry revisited" , Random House (1967)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> M. Berger, "Geometry" , '''II''' , Springer (1987) pp. Chapt. 10</TD></TR></table> | ||
Revision as of 14:52, 7 June 2020
trigonometry in the Euclidean plane.
The elements of a triangle, its sides 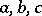 and its angles
and its angles  (
(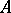 opposite to
opposite to  , etc.), are related in various ways. In the Euclidean plane the most important relations are the angle sum formula
, etc.), are related in various ways. In the Euclidean plane the most important relations are the angle sum formula
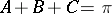 |
(angles in radians), and the triangle inequalities
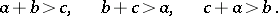 |
These inequalities are necessary and sufficient for three segments of positive length 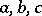 to form the sides of a triangle.
to form the sides of a triangle.
Another relation is the cosine theorem:
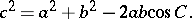 |
In particular, when  , the triangle is right-angled, and the cosine theorem becomes Pythagoras' theorem (cf. Pythagoras theorem)
, the triangle is right-angled, and the cosine theorem becomes Pythagoras' theorem (cf. Pythagoras theorem)
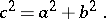 |
In such a right-angled triangle,
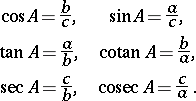 |
In a general triangle, further relations are provided by the sine theorem:
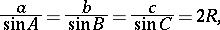 |
where 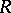 is the radius of the circumcircle of the triangle (cf. Inscribed and circumscribed figures). A corollary of the sine theorem is the tangent formula
is the radius of the circumcircle of the triangle (cf. Inscribed and circumscribed figures). A corollary of the sine theorem is the tangent formula
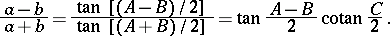 |
With the notation 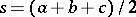 for the semi-perimeter of the triangle, the following half-angle formulas follow from the cosine theorem:
for the semi-perimeter of the triangle, the following half-angle formulas follow from the cosine theorem:
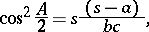 |
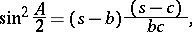 |
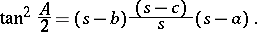 |
Geometry of the triangle.
Among the many remarkable lines, points and circles connected with any triangle are the circumcircle with centre 0 and radius 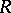 , the incircle and the three excircles with centres
, the incircle and the three excircles with centres  ,
, 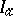 ,
,  ,
, 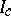 and radii
and radii  ,
, 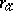 ,
, 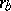 ,
, 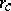 (cf. Inscribed and circumscribed figures), the medians (cf. Median (of a triangle))
(cf. Inscribed and circumscribed figures), the medians (cf. Median (of a triangle))  ,
, 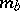 ,
, 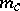 , with the centroid
, with the centroid 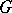 as their common point, the inner bisectors
as their common point, the inner bisectors 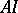 ,
, 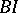 ,
, 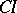 , and the outer bisectors
, and the outer bisectors 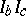 ,
, 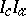 ,
, 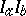 , the altitude lines
, the altitude lines  ,
, 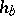 ,
,  with the orthocentre
with the orthocentre  as their common point, the Euler line (cf. Euler straight line) through
as their common point, the Euler line (cf. Euler straight line) through 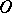 ,
, 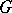 and
and 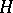 , and the nine-point circle through the midpoints of the sides, the feet of the altitude lines, and the midpoints of the segments connecting the vertices of the triangle to its orthocentre. The nine-point circle has radius
, and the nine-point circle through the midpoints of the sides, the feet of the altitude lines, and the midpoints of the segments connecting the vertices of the triangle to its orthocentre. The nine-point circle has radius 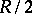 , its centre
, its centre 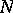 is on the Euler line between
is on the Euler line between 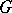 and
and 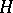 such that
such that  , and the nine-point circle touches the incircle and the three excircles (Feuerbach's theorem).
, and the nine-point circle touches the incircle and the three excircles (Feuerbach's theorem).
With the notation  for the area of the triangle
for the area of the triangle 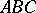 , the following relations are valid:
, the following relations are valid:
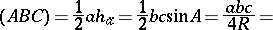 |
 |
It follows that, among others,
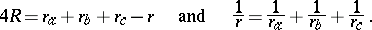 |
Very remarkable is Morley's theorem: The points of intersection of the adjacent trisectors of the angles of any triangle form the vertices of an equilateral triangle. Indeed, a direct calculation shows that the sides of Morley's triangle have length
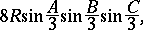 |
which is symmetric in 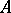 ,
, 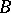 and
and 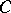 .
.
The theorems of Ceva and Menelaus.
Let 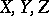 be points on the (possibly extended) sides
be points on the (possibly extended) sides 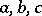 of a triangle
of a triangle 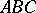 . Then, by the Ceva theorem,
. Then, by the Ceva theorem, 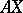 ,
, 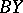 and
and 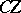 are congruent if and only if
are congruent if and only if 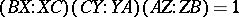 (signed distances) and by the Menelaus theorem,
(signed distances) and by the Menelaus theorem, 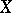 ,
, 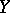 and
and  are collinear if and only if
are collinear if and only if 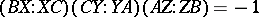 .
.
Convex quadrangles.
Ptolemy's theorem (cf. Ptolemeus theorem): For any point 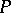 in the plane of a triangle
in the plane of a triangle 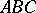 the inequality
the inequality
 |
holds, with equality if and only if 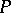 is on the arc
is on the arc 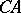 of the circumcircle of
of the circumcircle of 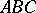 (in this last case,
(in this last case, 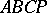 is a circle quadrangle).
is a circle quadrangle).
Brahmagupta's formula states that for any convex cyclic quadrangle 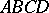 with area
with area 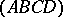 , sides
, sides  and semi-perimeter
and semi-perimeter  , the relation
, the relation  holds. In general, for any quadrangle
holds. In general, for any quadrangle 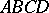 , the area
, the area 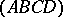 satisfies
satisfies
 |
It follows that among all quadrangles with given side lengths the inscribed quadrangles have maximum area (the cyclic order of the sides is immaterial).
Regular  -gons.
-gons.
A regular  -gon inscribed in a circle with radius
-gon inscribed in a circle with radius 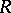 has perimeter
has perimeter 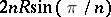 and area
and area 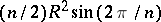 ; a regular
; a regular  -gon circumscribed about a circle with radius
-gon circumscribed about a circle with radius 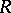 has perimeter
has perimeter 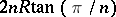 and area
and area 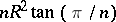 . See also Regular polyhedra.
. See also Regular polyhedra.
References
| [a1] | H.S.M. Coxeter, "Introduction to geometry" , Wiley (1969) pp. 3–23 |
| [a2] | H.S.M. Coxeter, S.L. Greitzer, "Geometry revisited" , Random House (1967) |
| [a3] | M. Berger, "Geometry" , II , Springer (1987) pp. Chapt. 10 |
Plane trigonometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Plane_trigonometry&oldid=49366