Moduli theory
A theory studying continuous families of objects in algebraic geometry.
Let 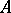 be a class of objects in algebraic geometry (varieties, schemes, vector bundles, etc.) on which an equivalence relation
be a class of objects in algebraic geometry (varieties, schemes, vector bundles, etc.) on which an equivalence relation 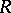 has been given. The fundamental classification problem (the description of the set of classes
has been given. The fundamental classification problem (the description of the set of classes 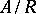 ) has the following two parts: 1) the description of discrete invariants, which usually allow a partition of
) has the following two parts: 1) the description of discrete invariants, which usually allow a partition of 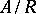 into a countable number of subsets, the objects of which already continuously depend on parameters; 2) the assignment and study of algebro-geometric structures on the parameter sets. The second part forms the matter of moduli theory.
into a countable number of subsets, the objects of which already continuously depend on parameters; 2) the assignment and study of algebro-geometric structures on the parameter sets. The second part forms the matter of moduli theory.
Moduli theory arose in the study of elliptic functions: There is a continuous family of different fields of elliptic functions (or of their models — isomorphic elliptic curves over  ), parametrized by the complex numbers. B. Riemann, who introduced the term "moduli" , showed that an algebraic function field over
), parametrized by the complex numbers. B. Riemann, who introduced the term "moduli" , showed that an algebraic function field over 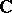 (or their models, compact Riemann surfaces) of genus
(or their models, compact Riemann surfaces) of genus 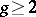 depends on
depends on 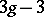 continuous complex parameters — the moduli.
continuous complex parameters — the moduli.
Basic concepts in moduli theory.
Let 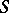 be a scheme (a complex or algebraic space). A family of objects parametrized by the scheme
be a scheme (a complex or algebraic space). A family of objects parametrized by the scheme 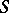 (or, as is often said, "scheme over Sover S" or "scheme with basis Swith basis S" ) is a set of objects
(or, as is often said, "scheme over Sover S" or "scheme with basis Swith basis S" ) is a set of objects
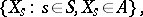 |
equipped with an additional structure compatible with the structure of the base  . This structure, in each concrete case, is given explicitly. A functor of families is a contravariant functor
. This structure, in each concrete case, is given explicitly. A functor of families is a contravariant functor 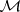 from the category of the schemes (or spaces) into the category of sets defined as follows:
from the category of the schemes (or spaces) into the category of sets defined as follows: 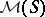 is the set of classes of isomorphic families over
is the set of classes of isomorphic families over 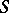 . To every morphism
. To every morphism 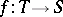 is associated a mapping
is associated a mapping 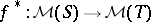 which assigns to a family over
which assigns to a family over 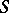 the pullback, or induced, family over
the pullback, or induced, family over  .
.
Let 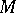 be an object in the category of schemes (complex or algebraic spaces) and let
be an object in the category of schemes (complex or algebraic spaces) and let 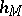 be a functor of the points in this category, that is,
be a functor of the points in this category, that is,  . If the functor of families
. If the functor of families 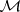 is representable, that is,
is representable, that is,  for some
for some 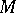 , then there exists a universal family with base
, then there exists a universal family with base 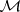 , and
, and 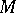 is called a fine moduli scheme (respectively, fine complex moduli space or fine algebraic moduli space). The functor
is called a fine moduli scheme (respectively, fine complex moduli space or fine algebraic moduli space). The functor 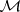 is representable in very few cases. Therefore the notion of a coarse moduli scheme was introduced.
is representable in very few cases. Therefore the notion of a coarse moduli scheme was introduced. 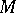 is called a coarse moduli scheme if there is a morphism of functors
is called a coarse moduli scheme if there is a morphism of functors  with the properties: a) if
with the properties: a) if  is one point (where
is one point (where 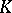 is an algebraically closed field), then the mapping
is an algebraically closed field), then the mapping 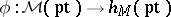 is bijective; in other words, the set of geometric points of the scheme
is bijective; in other words, the set of geometric points of the scheme 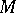 is in a natural one-to-one correspondence with the set of equivalence classes of parametrized objects; and b) for each scheme
is in a natural one-to-one correspondence with the set of equivalence classes of parametrized objects; and b) for each scheme  and morphism of functors
and morphism of functors  there is a unique morphism
there is a unique morphism 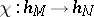 such that
such that 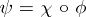 . Coarse schemes of complex and algebraic moduli spaces are similarly defined.
. Coarse schemes of complex and algebraic moduli spaces are similarly defined.
Although a coarse moduli scheme uniquely parametrizes the class of objects defined by given discrete invariants, the natural family over it (in contrast to the family over a fine moduli scheme) does not have the strong universality property. A coarse moduli scheme (space) already exists in a fairly large number of cases.
Examples. 1) Moduli of algebraic curves. Let 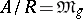 (respectively
(respectively 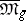 ) be the set of classes of isomorphic projective non-singular curves (respectively, stable curves) of genus
) be the set of classes of isomorphic projective non-singular curves (respectively, stable curves) of genus 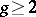 over an algebraically closed field
over an algebraically closed field 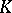 . A family over
. A family over 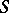 is a smooth (flat) proper morphism of schemes
is a smooth (flat) proper morphism of schemes  whose fibres are smooth (stable) curves of genus
whose fibres are smooth (stable) curves of genus 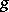 . Then there is a coarse (but not a fine) moduli scheme
. Then there is a coarse (but not a fine) moduli scheme  (respectively
(respectively  ), which is a quasi-projective (projective), irreducible, normal variety over
), which is a quasi-projective (projective), irreducible, normal variety over 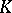 (see [3], [5], [6]).
(see [3], [5], [6]).
2) Moduli of algebraic curves with level  structure (with Jacobian rigidity). Let
structure (with Jacobian rigidity). Let  be a smooth family of projective curves (respectively, a flat family of stable curves) of genus
be a smooth family of projective curves (respectively, a flat family of stable curves) of genus 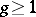 , let
, let  be an integer invertible on
be an integer invertible on 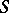 , and let
, and let 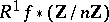 be the first direct image of the constant sheaf
be the first direct image of the constant sheaf 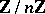 in the étale topology. Then
in the étale topology. Then 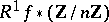 is locally free, has rank
is locally free, has rank 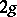 and is equipped with a locally non-degenerate symplectic form with values in
and is equipped with a locally non-degenerate symplectic form with values in 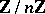 , up to an invertible element in
, up to an invertible element in 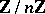 . A Jacobian structure of level
. A Jacobian structure of level  on
on 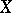 is an assignment of a symplectic isomorphism
is an assignment of a symplectic isomorphism
 |
Let 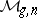 (respectively,
(respectively, 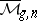 ) be the functor of families of smooth (stable) curves of genus
) be the functor of families of smooth (stable) curves of genus 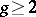 with a Jacobian rigidity of level
with a Jacobian rigidity of level  . Then for
. Then for  the functor
the functor 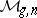 (respectively,
(respectively, 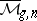 ) is represented by a quasi-projective (projective) scheme
) is represented by a quasi-projective (projective) scheme 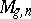 (respectively,
(respectively, 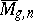 ) over
) over  , where
, where  is the inverse image of an
is the inverse image of an  -th root of unity, that is, there is a fine moduli scheme
-th root of unity, that is, there is a fine moduli scheme 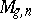 (respectively,
(respectively, 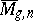 ) for the smooth (stable) curves of genus
) for the smooth (stable) curves of genus 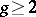 over a field of characteristic coprime with
over a field of characteristic coprime with  , equipped with a Jacobian rigidity of level
, equipped with a Jacobian rigidity of level  . For sufficiently large
. For sufficiently large  the scheme
the scheme 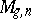 is smooth [5].
is smooth [5].
3) Polarized algebraic varieties. A polarized family is a pair 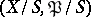 , where
, where 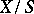 is a smooth family of varieties, i.e. a smooth proper morphism
is a smooth family of varieties, i.e. a smooth proper morphism 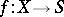 , and
, and 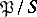 is the class of the relatively ample invertible sheaf
is the class of the relatively ample invertible sheaf 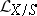 in
in 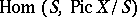 modulo
modulo 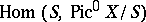 , where
, where  is the relative Picard scheme and
is the relative Picard scheme and 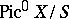 is the connected component of its zero section. In this case a functor of the polarized families
is the connected component of its zero section. In this case a functor of the polarized families 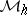 , with a given Hilbert polynomial
, with a given Hilbert polynomial 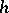 , is constructed. Without additional restrictions this functor is not representable. The existence of a coarse moduli space is known (1989) only in individual cases.
, is constructed. Without additional restrictions this functor is not representable. The existence of a coarse moduli space is known (1989) only in individual cases.
For polarized algebraic varieties the idea of rigidity of level  also exists.
also exists.
4) Vector bundles. There are also results on moduli spaces for vector bundles of rank  over an algebraic variety
over an algebraic variety 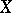 . In this case a family over
. In this case a family over 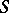 is a vector bundle over
is a vector bundle over 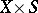 . Cf. [7], [10]–[14] for a description of results and more detail.
. Cf. [7], [10]–[14] for a description of results and more detail.
Local and global theory.
The local theory arose as the theory of deformations of complex structures (see Deformation 1) and 2)). The fundamental methods of the global theory are those of the theory of representable functors and geometric invariant theory, the theory of algebraic stacks, and the algebraization of formal moduli.
The method of construction of a global moduli space goes back to the classical theory of invariants (cf. Invariants, theory of). It is as follows. A sufficiently large family 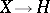 is constructed which contains representatives of all equivalence classes of the objects in questions, and so that the equivalence relation on
is constructed which contains representatives of all equivalence classes of the objects in questions, and so that the equivalence relation on 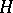 reduces to the action of an algebraic group
reduces to the action of an algebraic group 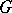 . Then the theory of actions of algebraic groups on algebraic varieties (schemes, spaces) is exploited with the aim of clarifying conditions for the existence of the quotient
. Then the theory of actions of algebraic groups on algebraic varieties (schemes, spaces) is exploited with the aim of clarifying conditions for the existence of the quotient 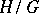 in the corresponding category. The basic tool in the construction of the family
in the corresponding category. The basic tool in the construction of the family  is the theory of Hilbert schemes (cf. Hilbert scheme). In such an approach the difficulty in constructing the family
is the theory of Hilbert schemes (cf. Hilbert scheme). In such an approach the difficulty in constructing the family 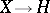 is reduced to the problem on a simultaneous immersion of the objects in question into a projective space. An important result on the possibility of such a simultaneous immersion is Matsusaka's theorem. Then the difficult problem remains of the existence of the quotient
is reduced to the problem on a simultaneous immersion of the objects in question into a projective space. An important result on the possibility of such a simultaneous immersion is Matsusaka's theorem. Then the difficult problem remains of the existence of the quotient 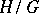 . Here one has the notions of categorical and geometric quotients. The construction of a coarse moduli space reduces to the problem of the existence of geometric quotients; here the idea of stability of points, corresponding to the idea of orbits in general position, is used. Results concerning actions of reductive groups on algebraic varieties over fields of characteristic
. Here one has the notions of categorical and geometric quotients. The construction of a coarse moduli space reduces to the problem of the existence of geometric quotients; here the idea of stability of points, corresponding to the idea of orbits in general position, is used. Results concerning actions of reductive groups on algebraic varieties over fields of characteristic  have been extended to the case of fields of characteristic
have been extended to the case of fields of characteristic 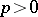 .
.
Another approach to global moduli theory is the method of algebraic stacks, that is, a method of globalization of local deformation theory. The first step in the investigation of the representability of a global functor of families in this approach is the establishment of the algebraizability of a formal versal deformation for each object  . The difficulty in the construction of a global moduli space is that not every factorization of the base of the family with respect to an equivalence relation is a separated space. In such cases the object representing the functor
. The difficulty in the construction of a global moduli space is that not every factorization of the base of the family with respect to an equivalence relation is a separated space. In such cases the object representing the functor 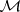 is replaced by an algebraic stack, the study of the properties of which gives some information on the moduli space.
is replaced by an algebraic stack, the study of the properties of which gives some information on the moduli space.
One of the approaches to global moduli theory over 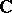 is the theory of period mappings (cf. Period mapping). The fundamental object here is the classifying space
is the theory of period mappings (cf. Period mapping). The fundamental object here is the classifying space 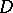 of polarized Hodge structures (cf. Hodge structure) of weight
of polarized Hodge structures (cf. Hodge structure) of weight 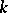 for given Hodge numbers. For a family
for given Hodge numbers. For a family  of polarized algebraic varieties over
of polarized algebraic varieties over 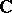 the periods define a mapping of
the periods define a mapping of 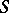 onto the corresponding classifying space
onto the corresponding classifying space 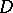 of Hodge structures. The moduli problem reduces to the study of conditions for the period mapping to be bijective. The presence of (global) injectivity for the period mapping is the so-called local-global Torelli problem. Along this route the existence of coarse moduli spaces has been proved for curves, Abelian varieties and
of Hodge structures. The moduli problem reduces to the study of conditions for the period mapping to be bijective. The presence of (global) injectivity for the period mapping is the so-called local-global Torelli problem. Along this route the existence of coarse moduli spaces has been proved for curves, Abelian varieties and 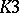 -surfaces.
-surfaces.
The compactification problem for a moduli variety  is that of finding a natural and complete (projective or compact, in the theory over the field
is that of finding a natural and complete (projective or compact, in the theory over the field 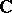 ) variety
) variety 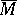 containing
containing  as a dense open subset, and also the description and geometric interpretation of the boundary
as a dense open subset, and also the description and geometric interpretation of the boundary 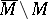 . In example 1) the natural compactification of the coarse moduli variety
. In example 1) the natural compactification of the coarse moduli variety  of curves of genus
of curves of genus 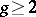 is the projective moduli variety
is the projective moduli variety 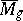 of stable curves. For polarized Abelian varieties over
of stable curves. For polarized Abelian varieties over  several means for compactifying moduli varieties are known.
several means for compactifying moduli varieties are known.
References
| [1] | M. Artin, "Algebraization of formal moduli 1" , Global analysis , Univ. Tokyo Press (1969) pp. 21–71 |
| [2] | M. Artin, "Versal deformations and algebraic stacks" Invent. Math. , 27 (1974) pp. 165–189 |
| [3] | P. Deligne, D. Mumford, "The irreducibility of the space of curves of given genus" Publ. Math. IHES , 36 (1969) pp. 75–109 |
| [4] | J. Dieudonné, J.B. Carrell, "Invariant theory, old and new" , Acad. Press (1971) |
| [5] | D. Mumford, "Geometric invariant theory" , Springer (1965) |
| [6] | D. Mumford, "Stability of projective varieties" l'Enseign. Math. (2) , 23 : 1–2 (1977) pp. 39–110 |
| [7] | D. Gieseker, "Global moduli for varieties of general type" Invent. Math. , 43 (1977) pp. 233–282 |
| [8] | D. Gieseker, "On the moduli of vector bundles on an algebraic surface" Ann. of Math. , 106 (1977) pp. 45–60 |
| [9] | T. Matsusaka, "Polarized varieties with a given Hilbert polynomial" Amer. J. Math. , 94 : 4 (1972) pp. 1027–1077 |
| [10] | P.E. Newstead, "Lectures on introduction to moduli problems and orbit spaces" , Springer (1978) |
| [11] | C. Okonek, M. Schneider, H. Spindler, "Vector bundles on complex projective spaces" , Birkhäuser (1980) |
| [12] | H. Popp, "Moduli theory and classification theory of algebraic varieties" , Springer (1977) |
| [13] | C.S. Seshadri, "Spaces of unitary vector bundles on a compact Riemann surface" Ann. of Math. , 85 (1967) pp. 302–336 |
| [14] | A.N. Tyurin, "The geometry of moduli of vector bundles" Russian Math. Surveys , 29 : 6 (1974) pp. 57–88 Uspekhi Mat. Nauk , 29 : 6 (1974) pp. 59–88 |
Comments
Much progress has been made recently in the study of the moduli spaces of algebraic curves and Abelian varieties; see the appendices of [a2]. Among the most important ones are the question of the compactification of 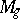 (see the appendix to Chapt. 5 in [a1], which is an enlarged edition of [5]) and the proof that
(see the appendix to Chapt. 5 in [a1], which is an enlarged edition of [5]) and the proof that 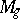 is of general type for
is of general type for 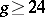 [a2].
[a2].
G. Faltings has constructed a compactification of the moduli space 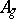 of principally-polarized Abelian varieties over
of principally-polarized Abelian varieties over 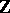 , see [a3].
, see [a3].
References
| [a1] | D. Mumford, J. Fogarty, "Geometric invariant theory" , Springer (1982) |
| [a2] | J. Harris, "Curves and their moduli" S.J. Bloch (ed.) , Algebraic geometry , Proc. Symp. Pure Math. , 46.1 , Amer. Math. Soc. (1985) pp. 99–143 |
| [a3] | G. Faltings, "Arithmetische Kompaktifizierung des Modulraums der abelschen Varietäten" F. Hirzebruch (ed.) J. Schwermer (ed.) S. Suter (ed.) , Arbeitstagung Bonn 1984 , Lect. notes in math. , 1111 , Springer (1985) pp. 321–383 |
Moduli theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Moduli_theory&oldid=13904