Difference between revisions of "Lebesgue theorem"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 7: | Line 7: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Engelking, "Dimension theory" , North-Holland & PWN (1978) pp. 19; 50</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) ((Appendix by L.S. Pontryagin and L.G. Shnirel'man in Russian edition.))</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> C. Kuratowski, "Introduction to set theory and topology" , Pergamon (1972) (Translated from Polish)</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Engelking, "Dimension theory" , North-Holland & PWN (1978) pp. 19; 50 {{MR|0482696}} {{MR|0482697}} {{ZBL|0401.54029}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) ((Appendix by L.S. Pontryagin and L.G. Shnirel'man in Russian edition.)) {{MR|}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> C. Kuratowski, "Introduction to set theory and topology" , Pergamon (1972) (Translated from Polish) {{MR|0346724}} {{ZBL|0267.54002}} {{ZBL|0247.54001}} </TD></TR></table> |
Lebesgue's theorem on the passage to the limit under the integral sign: Suppose that on a measurable set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795013.png" /> there is specified a sequence of measurable functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795014.png" /> that converges almost-everywhere (or in measure) on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795015.png" /> to a function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795016.png" />. If there is a summable function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795017.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795018.png" /> such that for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795019.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795020.png" />, | Lebesgue's theorem on the passage to the limit under the integral sign: Suppose that on a measurable set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795013.png" /> there is specified a sequence of measurable functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795014.png" /> that converges almost-everywhere (or in measure) on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795015.png" /> to a function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795016.png" />. If there is a summable function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795017.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795018.png" /> such that for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795019.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057950/l05795020.png" />, | ||
| Line 28: | Line 28: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Lebesgue, "Sur les intégrales singuliéres" ''Ann. Fac. Sci. Univ. Toulouse Sci. Math. Sci. Phys.'' , '''1''' (1909) pp. 25–117</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> H. Lebesgue, "Intégrale, longueur, aire" , Univ. Paris (1902) (Thesis)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> B. Levi, "Sopra l'integrazione delle serie" ''Rend. Ist. Lombardo sue Lett. (2)'' , '''39''' (1906) pp. 775–780</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> I.P. Natanson, "Theory of functions of a real variable" , '''1–2''' , F. Ungar (1955–1961) (Translated from Russian)</TD></TR></table> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Lebesgue, "Sur les intégrales singuliéres" ''Ann. Fac. Sci. Univ. Toulouse Sci. Math. Sci. Phys.'' , '''1''' (1909) pp. 25–117 {{MR|1508308}} {{ZBL|41.0329.01}} {{ZBL|41.0327.02}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> H. Lebesgue, "Intégrale, longueur, aire" , Univ. Paris (1902) (Thesis) {{MR|}} {{ZBL|33.0307.02}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> B. Levi, "Sopra l'integrazione delle serie" ''Rend. Ist. Lombardo sue Lett. (2)'' , '''39''' (1906) pp. 775–780 {{MR|}} {{ZBL|37.0424.03}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French) {{MR|0167578}} {{ZBL|1196.28001}} {{ZBL|0017.30004}} {{ZBL|63.0183.05}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> I.P. Natanson, "Theory of functions of a real variable" , '''1–2''' , F. Ungar (1955–1961) (Translated from Russian) {{MR|0640867}} {{MR|0354979}} {{MR|0148805}} {{MR|0067952}} {{MR|0039790}} {{ZBL|}} </TD></TR></table> |
''T.P. Lukashenko'' | ''T.P. Lukashenko'' | ||
| Line 36: | Line 36: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N. Dunford, J.T. Schwartz, "Linear operators" , '''1–3''' , Interscience (1958–1971)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> P.R. Halmos, "Measure theory" , v. Nostrand (1950)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965)</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N. Dunford, J.T. Schwartz, "Linear operators" , '''1–3''' , Interscience (1958–1971) {{MR|1009164}} {{MR|1009163}} {{MR|1009162}} {{MR|0412888}} {{MR|0216304}} {{MR|0188745}} {{MR|0216303}} {{MR|1530651}} {{MR|0117523}} {{ZBL|0635.47003}} {{ZBL|0635.47002}} {{ZBL|0635.47001}} {{ZBL|0283.47002}} {{ZBL|0243.47001}} {{ZBL|0146.12601}} {{ZBL|0128.34803}} {{ZBL|0084.10402}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> P.R. Halmos, "Measure theory" , v. Nostrand (1950) {{MR|0033869}} {{ZBL|0040.16802}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965) {{MR|0188387}} {{ZBL|0137.03202}} </TD></TR></table> |
Revision as of 12:11, 27 September 2012
Lebesgue's theorem in dimension theory: For any 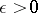 the
the  -dimensional cube has a finite closed
-dimensional cube has a finite closed  -covering of multiplicity
-covering of multiplicity 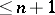 , and at the same there is an
, and at the same there is an 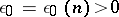 such that any finite closed
such that any finite closed  -covering of the
-covering of the  -dimensional cube has multiplicity
-dimensional cube has multiplicity 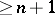 (cf. also Covering (of a set)). This assertion led later to a definition of a fundamental dimension invariant, the Lebesgue dimension
(cf. also Covering (of a set)). This assertion led later to a definition of a fundamental dimension invariant, the Lebesgue dimension 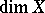 of a normal topological space
of a normal topological space  .
.
Comments
This theorem is also called the Lebesgue covering theorem or "PflastersatzPflastersatz" (see Dimension). In the language of dimension theory it says that 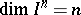 for every
for every  .
.
References
| [a1] | R. Engelking, "Dimension theory" , North-Holland & PWN (1978) pp. 19; 50 MR0482696 MR0482697 Zbl 0401.54029 |
| [a2] | W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) ((Appendix by L.S. Pontryagin and L.G. Shnirel'man in Russian edition.)) |
| [a3] | C. Kuratowski, "Introduction to set theory and topology" , Pergamon (1972) (Translated from Polish) MR0346724 Zbl 0267.54002 Zbl 0247.54001 |
Lebesgue's theorem on the passage to the limit under the integral sign: Suppose that on a measurable set 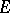 there is specified a sequence of measurable functions
there is specified a sequence of measurable functions 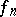 that converges almost-everywhere (or in measure) on
that converges almost-everywhere (or in measure) on 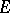 to a function
to a function 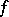 . If there is a summable function
. If there is a summable function 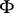 on
on 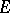 such that for all
such that for all  and
and  ,
,
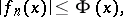 |
then 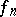 and
and  are summable on
are summable on  and
and
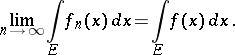 |
This was first proved by H. Lebesgue [1]. The important special case when 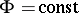 and
and  has finite measure is also called the Lebesgue theorem; he obtained it earlier [2].
has finite measure is also called the Lebesgue theorem; he obtained it earlier [2].
A theorem first proved by B. Levi [3] is sometimes called the Lebesgue theorem: Suppose that on a measurable set 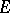 there is specified a non-decreasing sequence of measurable non-negative functions
there is specified a non-decreasing sequence of measurable non-negative functions 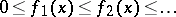 (
(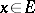 ) and that
) and that
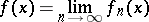 |
almost-everywhere; then
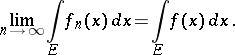 |
References
| [1] | H. Lebesgue, "Sur les intégrales singuliéres" Ann. Fac. Sci. Univ. Toulouse Sci. Math. Sci. Phys. , 1 (1909) pp. 25–117 MR1508308 Zbl 41.0329.01 Zbl 41.0327.02 |
| [2] | H. Lebesgue, "Intégrale, longueur, aire" , Univ. Paris (1902) (Thesis) Zbl 33.0307.02 |
| [3] | B. Levi, "Sopra l'integrazione delle serie" Rend. Ist. Lombardo sue Lett. (2) , 39 (1906) pp. 775–780 Zbl 37.0424.03 |
| [4] | S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French) MR0167578 Zbl 1196.28001 Zbl 0017.30004 Zbl 63.0183.05 |
| [5] | I.P. Natanson, "Theory of functions of a real variable" , 1–2 , F. Ungar (1955–1961) (Translated from Russian) MR0640867 MR0354979 MR0148805 MR0067952 MR0039790 |
T.P. Lukashenko
Comments
This Lebesgue theorem is also called the dominated convergence theorem, while Levi's theorem is also known as the monotone convergence theorem.
References
| [a1] | N. Dunford, J.T. Schwartz, "Linear operators" , 1–3 , Interscience (1958–1971) MR1009164 MR1009163 MR1009162 MR0412888 MR0216304 MR0188745 MR0216303 MR1530651 MR0117523 Zbl 0635.47003 Zbl 0635.47002 Zbl 0635.47001 Zbl 0283.47002 Zbl 0243.47001 Zbl 0146.12601 Zbl 0128.34803 Zbl 0084.10402 |
| [a2] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) MR0033869 Zbl 0040.16802 |
| [a3] | E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965) MR0188387 Zbl 0137.03202 |
Lebesgue theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lebesgue_theorem&oldid=12122