|
|
| Line 1: |
Line 1: |
| | + | <!-- |
| | + | i0525201.png |
| | + | $#A+1 = 134 n = 1 |
| | + | $#C+1 = 134 : ~/encyclopedia/old_files/data/I052/I.0502520 Involution algebra, |
| | + | Automatically converted into TeX, above some diagnostics. |
| | + | Please remove this comment and the {{TEX|auto}} line below, |
| | + | if TeX found to be correct. |
| | + | --> |
| | + | |
| | + | {{TEX|auto}} |
| | + | {{TEX|done}} |
| | + | |
| | ''algebra with involution'' | | ''algebra with involution'' |
| | | | |
| − | An algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525201.png" /> over the field of complex numbers endowed with an [[Involution|involution]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525202.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525203.png" />. Some examples are: the algebra of continuous functions on a compact set, in which the involution sends any function to its complex conjugate; the algebra of bounded linear operators on a Hilbert space, in which the involution sends any operator to its adjoint; the [[Group algebra of a locally compact group|group algebra of a locally compact group]]; and the algebra of measures on a locally compact group. The element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525204.png" /> is called the conjugate, or adjoint, of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525205.png" />. An element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525206.png" /> is called self-adjoint, or Hermitian, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525207.png" />, and normal if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525208.png" />. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i0525209.png" /> contains a unit element 1, then an element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252010.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252011.png" /> is called unitary. The set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252012.png" /> of Hermitian elements of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252013.png" /> is a real vector subspace of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252014.png" />, and any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252015.png" /> can be uniquely written in the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252016.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252017.png" />. In this case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252018.png" /> is normal if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252019.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252020.png" /> commute. Every element of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252021.png" /> is Hermitian, and so is the unit element. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252022.png" /> is invertible, then so is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252023.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252024.png" />. The spectrum of any Hermitian element (cf. [[Spectrum of an element|Spectrum of an element]]) is symmetric about the real axis. An involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252025.png" /> is called a total involution algebra if the spectrum of any element of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252026.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252027.png" />, is contained in the set of non-negative real numbers. Examples of total involution algebras are: the involution algebra of continuous functions on a compact set; the involution algebra of bounded linear operators on a Hilbert space; and group algebras of compact and commutative locally compact groups. The group algebras of non-compact semi-simple Lie groups are not total involution algebras. A commutative involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252028.png" /> is a total involution algebra if and only if all its maximal ideals are symmetric, or if and only if all characters of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252029.png" /> are Hermitian. Every [[C*-algebra|<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252030.png" />-algebra]] is a total involution algebra. | + | An algebra $ E $ |
| | + | over the field of complex numbers endowed with an [[Involution|involution]] $ x \mapsto x ^ {*} $, |
| | + | $ x \in E $. |
| | + | Some examples are: the algebra of continuous functions on a compact set, in which the involution sends any function to its complex conjugate; the algebra of bounded linear operators on a Hilbert space, in which the involution sends any operator to its adjoint; the [[Group algebra of a locally compact group|group algebra of a locally compact group]]; and the algebra of measures on a locally compact group. The element $ x ^ {*} \in E $ |
| | + | is called the conjugate, or adjoint, of $ x $. |
| | + | An element $ x \in E $ |
| | + | is called self-adjoint, or Hermitian, if $ x ^ {*} = x $, |
| | + | and normal if $ x ^ {*} x = x x ^ {*} $. |
| | + | If $ E $ |
| | + | contains a unit element 1, then an element $ x \in E $ |
| | + | such that $ x ^ {*} x = x x ^ {*} = 1 $ |
| | + | is called unitary. The set $ E _ {h} $ |
| | + | of Hermitian elements of $ E $ |
| | + | is a real vector subspace of $ E $, |
| | + | and any $ x \in E $ |
| | + | can be uniquely written in the form $ x = x _ {1} + i x _ {2} $, |
| | + | where $ x _ {1} , x _ {2} \in E _ {h} $. |
| | + | In this case $ x \in E $ |
| | + | is normal if and only if $ x _ {1} $ |
| | + | and $ x _ {2} $ |
| | + | commute. Every element of the form $ x ^ {*} x $ |
| | + | is Hermitian, and so is the unit element. If $ x $ |
| | + | is invertible, then so is $ x ^ {*} $, |
| | + | and $ ( x ^ {*} ) ^ {-} 1 = ( x ^ {-} 1 ) ^ {*} $. |
| | + | The spectrum of any Hermitian element (cf. [[Spectrum of an element|Spectrum of an element]]) is symmetric about the real axis. An involution algebra $ E $ |
| | + | is called a total involution algebra if the spectrum of any element of the form $ x ^ {*} x $, |
| | + | $ x \in E $, |
| | + | is contained in the set of non-negative real numbers. Examples of total involution algebras are: the involution algebra of continuous functions on a compact set; the involution algebra of bounded linear operators on a Hilbert space; and group algebras of compact and commutative locally compact groups. The group algebras of non-compact semi-simple Lie groups are not total involution algebras. A commutative involution algebra $ E $ |
| | + | is a total involution algebra if and only if all its maximal ideals are symmetric, or if and only if all characters of $ E $ |
| | + | are Hermitian. Every [[C*-algebra| $ C ^ {*} $- |
| | + | algebra]] is a total involution algebra. |
| | | | |
| − | A subset <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252031.png" /> of an involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252032.png" /> is called an involution set if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252033.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252034.png" />. A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252035.png" /> of involution algebras is called an involution mapping if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252036.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252037.png" />. The kernel of an involution homomorphism of involution algebras is a symmetric two-sided ideal. Every symmetric one-sided ideal is two-sided and the quotient algebra of an involution algebra by a symmetric ideal admits the structure of an involution algebra in a natural way. The radical (cf. [[Radical of rings and algebras|Radical of rings and algebras]]) of an involution algebra is a symmetric ideal. An involution subalgebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252038.png" /> of an involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252039.png" /> is an involution algebra. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252040.png" /> be the direct sum of an involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252041.png" /> and the field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252042.png" />, in which the linear operations and the involution are defined componentwise and the multiplication is given by | + | A subset $ M $ |
| | + | of an involution algebra $ E $ |
| | + | is called an involution set if $ x ^ {*} \in M $ |
| | + | for all $ x \in M $. |
| | + | A mapping $ \phi : E \rightarrow F $ |
| | + | of involution algebras is called an involution mapping if $ \phi ( x) ^ {*} = \phi ( x ^ {*} ) $ |
| | + | for all $ x \in E $. |
| | + | The kernel of an involution homomorphism of involution algebras is a symmetric two-sided ideal. Every symmetric one-sided ideal is two-sided and the quotient algebra of an involution algebra by a symmetric ideal admits the structure of an involution algebra in a natural way. The radical (cf. [[Radical of rings and algebras|Radical of rings and algebras]]) of an involution algebra is a symmetric ideal. An involution subalgebra $ F $ |
| | + | of an involution algebra $ E $ |
| | + | is an involution algebra. Let $ \widetilde{E} $ |
| | + | be the direct sum of an involution algebra $ E $ |
| | + | and the field $ \mathbf C $, |
| | + | in which the linear operations and the involution are defined componentwise and the multiplication is given by |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252043.png" /></td> </tr></table>
| + | $$ |
| | + | \{ x , \lambda \} \{ y , \mu \} = \ |
| | + | \{ x y + \lambda y + \mu x , \lambda \mu \} |
| | + | $$ |
| | | | |
| − | for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252044.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252045.png" />. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252046.png" /> is an involution algebra with a unit element. | + | for all $ \lambda , \mu \in \mathbf C $, |
| | + | $ x , y \in E $. |
| | + | Then $ \widetilde{E} $ |
| | + | is an involution algebra with a unit element. |
| | | | |
| − | A linear functional <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252047.png" /> on an involution algebra is called Hermitian if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252048.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252049.png" />, and positive if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252050.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252051.png" />. The set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252052.png" /> of Hermitian linear functionals on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252053.png" /> is a real vector subspace of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252054.png" />, the dual of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252055.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252056.png" /> is the direct sum of the subspaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252057.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252058.png" />. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252059.png" /> has a unit 1, then every positive functional <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252060.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252061.png" /> is Hermitian and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252062.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252063.png" />. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252064.png" /> is a positive functional on an involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252065.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252066.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252067.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252068.png" />. | + | A linear functional $ f $ |
| | + | on an involution algebra is called Hermitian if $ f ( x ^ {*} ) = \overline{ {f ( x) }}\; $ |
| | + | for all $ x \in E $, |
| | + | and positive if $ f ( x ^ {*} x ) \geq 0 $ |
| | + | for all $ x \in E $. |
| | + | The set $ E _ {h} ^ \prime $ |
| | + | of Hermitian linear functionals on $ E $ |
| | + | is a real vector subspace of $ E ^ \prime $, |
| | + | the dual of $ E $, |
| | + | and $ E ^ \prime $ |
| | + | is the direct sum of the subspaces $ E _ {h} ^ \prime $ |
| | + | and $ i E _ {h} ^ \prime $. |
| | + | If $ E $ |
| | + | has a unit 1, then every positive functional $ f $ |
| | + | on $ E $ |
| | + | is Hermitian and $ | f ( x) | ^ {2} \leq f ( 1) f ( x ^ {*} x ) $ |
| | + | for all $ x \in E $. |
| | + | If $ f $ |
| | + | is a positive functional on an involution algebra $ E $, |
| | + | then $ f ( y ^ {*} x ) = f ( x ^ {*} y ) $ |
| | + | and $ | f ( y ^ {*} x ) | ^ {2} \leq f ( y ^ {*} y) f( x ^ {*} x ) $ |
| | + | for all $ x , y \in E $. |
| | | | |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252069.png" /> be an involution algebra equipped with a norm making <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252070.png" /> into a [[Normed algebra|normed algebra]] and satisfying the condition <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252071.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252072.png" />. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252073.png" /> is called a normed algebra with involution. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252074.png" /> is complete with respect to this norm, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252075.png" /> is called a Banach algebra with involution. Every normed algebra with involution <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252076.png" /> can be imbedded in a Banach algebra with involution <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252077.png" /> containing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252078.png" /> as a dense involution subalgebra. <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252079.png" /> is uniquely defined up to an isometric involution isomorphism. <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252080.png" /> is called the completion of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252081.png" />. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252082.png" /> is a Banach algebra with involution having an approximate identity, then every positive linear functional <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252083.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252084.png" /> is continuous and can be extended to a positive linear functional on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252085.png" />. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252086.png" /> has a unit 1 and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252087.png" />, then for any positive linear functional <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252088.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252089.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252090.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252091.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252092.png" /> is the [[Spectral radius|spectral radius]] of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252093.png" /> (see [[Banach algebra|Banach algebra]]). | + | Let $ E $ |
| | + | be an involution algebra equipped with a norm making $ E $ |
| | + | into a [[Normed algebra|normed algebra]] and satisfying the condition $ \| x ^ {*} \| = \| x \| $ |
| | + | for all $ x \in E $. |
| | + | Then $ E $ |
| | + | is called a normed algebra with involution. If $ E $ |
| | + | is complete with respect to this norm, then $ E $ |
| | + | is called a Banach algebra with involution. Every normed algebra with involution $ E $ |
| | + | can be imbedded in a Banach algebra with involution $ \overline{E}\; $ |
| | + | containing $ E $ |
| | + | as a dense involution subalgebra. $ \overline{E}\; $ |
| | + | is uniquely defined up to an isometric involution isomorphism. $ \overline{E}\; $ |
| | + | is called the completion of $ E $. |
| | + | If $ E $ |
| | + | is a Banach algebra with involution having an approximate identity, then every positive linear functional $ f $ |
| | + | on $ E $ |
| | + | is continuous and can be extended to a positive linear functional on $ \overline{E}\; $. |
| | + | If $ E $ |
| | + | has a unit 1 and $ \| 1 \| = 1 $, |
| | + | then for any positive linear functional $ f $ |
| | + | on $ E $, |
| | + | $ \| f \| = f ( 1) $ |
| | + | and $ f ( x ^ {*} x ) \leq f ( 1) r ( x ^ {*} x ) $, |
| | + | where $ r ( x ^ {*} x ) $ |
| | + | is the [[Spectral radius|spectral radius]] of $ x ^ {*} x $( |
| | + | see [[Banach algebra|Banach algebra]]). |
| | | | |
| − | A Hermitian element of a total involution algebra has a real spectrum. For any maximal closed left ideal <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252094.png" /> in a total involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252095.png" /> with a unit there is a positive linear functional on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252096.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252097.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252098.png" />. An element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i05252099.png" /> in a total involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520100.png" /> is left-invertible in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520101.png" /> if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520102.png" /> for all non-zero positive functionals <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520103.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520104.png" />. The radical of a total involution algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520105.png" /> coincides with the set of elements <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520106.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520107.png" /> for all positive linear functionals <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520108.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520109.png" />. A Banach algebra with involution <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520110.png" /> with a unit 1 is a total involution algebra if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520111.png" />, where the supremum is taken over the set of positive linear functionals <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520112.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520113.png" /> for which <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520114.png" />. | + | A Hermitian element of a total involution algebra has a real spectrum. For any maximal closed left ideal $ I $ |
| | + | in a total involution algebra $ E $ |
| | + | with a unit there is a positive linear functional on $ f $ |
| | + | on $ E $ |
| | + | such that $ I = \{ {x \in E } : {f ( x ^ {*} x ) = 0 } \} $. |
| | + | An element $ x $ |
| | + | in a total involution algebra $ E $ |
| | + | is left-invertible in $ E $ |
| | + | if and only if $ f ( x ^ {*} x ) > 0 $ |
| | + | for all non-zero positive functionals $ f $ |
| | + | on $ E $. |
| | + | The radical of a total involution algebra $ E $ |
| | + | coincides with the set of elements $ x \in E $ |
| | + | such that $ f ( x ^ {*} x ) = 0 $ |
| | + | for all positive linear functionals $ f $ |
| | + | on $ E $. |
| | + | A Banach algebra with involution $ E $ |
| | + | with a unit 1 is a total involution algebra if and only if $ r ( x ^ {*} x ) = \sup f ( x ^ {*} x ) $, |
| | + | where the supremum is taken over the set of positive linear functionals $ f $ |
| | + | on $ E $ |
| | + | for which $ f ( 1) = 1 $. |
| | | | |
| | ====References==== | | ====References==== |
| | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> M.A. Naimark, "Normed rings" , Reidel (1984) (Translated from Russian)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J. Dixmier, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520115.png" /> algebras" , North-Holland (1977) (Translated from French)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> V. Pták, "Banach algebras with involution" ''Manuscripta Math.'' , '''6''' : 3 (1972) pp. 245–290</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , '''2''' , Springer (1979)</TD></TR></table> | | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> M.A. Naimark, "Normed rings" , Reidel (1984) (Translated from Russian)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J. Dixmier, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520115.png" /> algebras" , North-Holland (1977) (Translated from French)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> V. Pták, "Banach algebras with involution" ''Manuscripta Math.'' , '''6''' : 3 (1972) pp. 245–290</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , '''2''' , Springer (1979)</TD></TR></table> |
| − |
| |
| − |
| |
| | | | |
| | ====Comments==== | | ====Comments==== |
| − | A (Banach, normed) algebra with involution is also called an involutive (Banach, normed) algebra. If in an involutive Banach algebra <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520116.png" /> one has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520117.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520118.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520119.png" /> is called a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520121.png" />-algebra. | + | A (Banach, normed) algebra with involution is also called an involutive (Banach, normed) algebra. If in an involutive Banach algebra $ A $ |
| | + | one has $ \| x x ^ {*} \| = \| x \| ^ {2} $ |
| | + | for all $ x \in A $, |
| | + | then $ A $ |
| | + | is called a $ B ^ {*} $- |
| | + | algebra. |
| | | | |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520122.png" /> be a Banach algebra. A left-approximate identity in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520123.png" /> is a net <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520124.png" /> of elements of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520125.png" /> (cf. [[Net (directed set)|Net (directed set)]]) such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520126.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520127.png" />. A right-approximate identity is similarly defined, using <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520128.png" />. A left- and right-approximate identity is simply called an approximate identity. Every <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520129.png" />-algebra has an approximate identity. | + | Let $ A $ |
| | + | be a Banach algebra. A left-approximate identity in $ A $ |
| | + | is a net $ \{ e _ {i} \} _ {i \in I } $ |
| | + | of elements of $ A $( |
| | + | cf. [[Net (directed set)|Net (directed set)]]) such that $ \lim\limits \| e _ {i} x - x \| = 0 $ |
| | + | for all $ x \in A $. |
| | + | A right-approximate identity is similarly defined, using $ \| x e _ {i} - x \| $. |
| | + | A left- and right-approximate identity is simply called an approximate identity. Every $ B ^ {*} $- |
| | + | algebra has an approximate identity. |
| | | | |
| − | An algebra with involution <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520130.png" /> is also termed a symmetric algebra, and a total involution algebra is also called a completely symmetric algebra. Correspondingly, a homomorphism of algebras with involution <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520131.png" /> is called a symmetric homomorphism if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520132.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520133.png" />. Unfortunately, the term symmetric algebra is also sometimes used to mean a total involution algebra. | + | An algebra with involution $ E $ |
| | + | is also termed a symmetric algebra, and a total involution algebra is also called a completely symmetric algebra. Correspondingly, a homomorphism of algebras with involution $ \phi : E \rightarrow F $ |
| | + | is called a symmetric homomorphism if $ \phi ( x ^ {*} ) = \phi ( x ) ^ {*} $ |
| | + | for all $ x \in E $. |
| | + | Unfortunately, the term symmetric algebra is also sometimes used to mean a total involution algebra. |
| | | | |
| − | A symmetric ideal of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520134.png" /> is an ideal <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520135.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/i/i052/i052520/i052520136.png" />. | + | A symmetric ideal of $ E $ |
| | + | is an ideal $ M $ |
| | + | such that $ M ^ {*} = \{ {m ^ {*} } : {m \in M } \} = M $. |
| | | | |
| | ====References==== | | ====References==== |
| | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> W. Rudin, "Functional analysis" , McGraw-Hill (1979)</TD></TR></table> | | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> W. Rudin, "Functional analysis" , McGraw-Hill (1979)</TD></TR></table> |
algebra with involution
An algebra $ E $
over the field of complex numbers endowed with an involution $ x \mapsto x ^ {*} $,
$ x \in E $.
Some examples are: the algebra of continuous functions on a compact set, in which the involution sends any function to its complex conjugate; the algebra of bounded linear operators on a Hilbert space, in which the involution sends any operator to its adjoint; the group algebra of a locally compact group; and the algebra of measures on a locally compact group. The element $ x ^ {*} \in E $
is called the conjugate, or adjoint, of $ x $.
An element $ x \in E $
is called self-adjoint, or Hermitian, if $ x ^ {*} = x $,
and normal if $ x ^ {*} x = x x ^ {*} $.
If $ E $
contains a unit element 1, then an element $ x \in E $
such that $ x ^ {*} x = x x ^ {*} = 1 $
is called unitary. The set $ E _ {h} $
of Hermitian elements of $ E $
is a real vector subspace of $ E $,
and any $ x \in E $
can be uniquely written in the form $ x = x _ {1} + i x _ {2} $,
where $ x _ {1} , x _ {2} \in E _ {h} $.
In this case $ x \in E $
is normal if and only if $ x _ {1} $
and $ x _ {2} $
commute. Every element of the form $ x ^ {*} x $
is Hermitian, and so is the unit element. If $ x $
is invertible, then so is $ x ^ {*} $,
and $ ( x ^ {*} ) ^ {-} 1 = ( x ^ {-} 1 ) ^ {*} $.
The spectrum of any Hermitian element (cf. Spectrum of an element) is symmetric about the real axis. An involution algebra $ E $
is called a total involution algebra if the spectrum of any element of the form $ x ^ {*} x $,
$ x \in E $,
is contained in the set of non-negative real numbers. Examples of total involution algebras are: the involution algebra of continuous functions on a compact set; the involution algebra of bounded linear operators on a Hilbert space; and group algebras of compact and commutative locally compact groups. The group algebras of non-compact semi-simple Lie groups are not total involution algebras. A commutative involution algebra $ E $
is a total involution algebra if and only if all its maximal ideals are symmetric, or if and only if all characters of $ E $
are Hermitian. Every $ C ^ {*} $-
algebra is a total involution algebra.
A subset $ M $
of an involution algebra $ E $
is called an involution set if $ x ^ {*} \in M $
for all $ x \in M $.
A mapping $ \phi : E \rightarrow F $
of involution algebras is called an involution mapping if $ \phi ( x) ^ {*} = \phi ( x ^ {*} ) $
for all $ x \in E $.
The kernel of an involution homomorphism of involution algebras is a symmetric two-sided ideal. Every symmetric one-sided ideal is two-sided and the quotient algebra of an involution algebra by a symmetric ideal admits the structure of an involution algebra in a natural way. The radical (cf. Radical of rings and algebras) of an involution algebra is a symmetric ideal. An involution subalgebra $ F $
of an involution algebra $ E $
is an involution algebra. Let $ \widetilde{E} $
be the direct sum of an involution algebra $ E $
and the field $ \mathbf C $,
in which the linear operations and the involution are defined componentwise and the multiplication is given by
$$
\{ x , \lambda \} \{ y , \mu \} = \
\{ x y + \lambda y + \mu x , \lambda \mu \}
$$
for all $ \lambda , \mu \in \mathbf C $,
$ x , y \in E $.
Then $ \widetilde{E} $
is an involution algebra with a unit element.
A linear functional $ f $
on an involution algebra is called Hermitian if $ f ( x ^ {*} ) = \overline{ {f ( x) }}\; $
for all $ x \in E $,
and positive if $ f ( x ^ {*} x ) \geq 0 $
for all $ x \in E $.
The set $ E _ {h} ^ \prime $
of Hermitian linear functionals on $ E $
is a real vector subspace of $ E ^ \prime $,
the dual of $ E $,
and $ E ^ \prime $
is the direct sum of the subspaces $ E _ {h} ^ \prime $
and $ i E _ {h} ^ \prime $.
If $ E $
has a unit 1, then every positive functional $ f $
on $ E $
is Hermitian and $ | f ( x) | ^ {2} \leq f ( 1) f ( x ^ {*} x ) $
for all $ x \in E $.
If $ f $
is a positive functional on an involution algebra $ E $,
then $ f ( y ^ {*} x ) = f ( x ^ {*} y ) $
and $ | f ( y ^ {*} x ) | ^ {2} \leq f ( y ^ {*} y) f( x ^ {*} x ) $
for all $ x , y \in E $.
Let $ E $
be an involution algebra equipped with a norm making $ E $
into a normed algebra and satisfying the condition $ \| x ^ {*} \| = \| x \| $
for all $ x \in E $.
Then $ E $
is called a normed algebra with involution. If $ E $
is complete with respect to this norm, then $ E $
is called a Banach algebra with involution. Every normed algebra with involution $ E $
can be imbedded in a Banach algebra with involution $ \overline{E}\; $
containing $ E $
as a dense involution subalgebra. $ \overline{E}\; $
is uniquely defined up to an isometric involution isomorphism. $ \overline{E}\; $
is called the completion of $ E $.
If $ E $
is a Banach algebra with involution having an approximate identity, then every positive linear functional $ f $
on $ E $
is continuous and can be extended to a positive linear functional on $ \overline{E}\; $.
If $ E $
has a unit 1 and $ \| 1 \| = 1 $,
then for any positive linear functional $ f $
on $ E $,
$ \| f \| = f ( 1) $
and $ f ( x ^ {*} x ) \leq f ( 1) r ( x ^ {*} x ) $,
where $ r ( x ^ {*} x ) $
is the spectral radius of $ x ^ {*} x $(
see Banach algebra).
A Hermitian element of a total involution algebra has a real spectrum. For any maximal closed left ideal $ I $
in a total involution algebra $ E $
with a unit there is a positive linear functional on $ f $
on $ E $
such that $ I = \{ {x \in E } : {f ( x ^ {*} x ) = 0 } \} $.
An element $ x $
in a total involution algebra $ E $
is left-invertible in $ E $
if and only if $ f ( x ^ {*} x ) > 0 $
for all non-zero positive functionals $ f $
on $ E $.
The radical of a total involution algebra $ E $
coincides with the set of elements $ x \in E $
such that $ f ( x ^ {*} x ) = 0 $
for all positive linear functionals $ f $
on $ E $.
A Banach algebra with involution $ E $
with a unit 1 is a total involution algebra if and only if $ r ( x ^ {*} x ) = \sup f ( x ^ {*} x ) $,
where the supremum is taken over the set of positive linear functionals $ f $
on $ E $
for which $ f ( 1) = 1 $.
References
| [1] | M.A. Naimark, "Normed rings" , Reidel (1984) (Translated from Russian) |
| [2] | J. Dixmier, "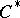 algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
| [3] | V. Pták, "Banach algebras with involution" Manuscripta Math. , 6 : 3 (1972) pp. 245–290 |
| [4] | E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , 2 , Springer (1979) |
A (Banach, normed) algebra with involution is also called an involutive (Banach, normed) algebra. If in an involutive Banach algebra $ A $
one has $ \| x x ^ {*} \| = \| x \| ^ {2} $
for all $ x \in A $,
then $ A $
is called a $ B ^ {*} $-
algebra.
Let $ A $
be a Banach algebra. A left-approximate identity in $ A $
is a net $ \{ e _ {i} \} _ {i \in I } $
of elements of $ A $(
cf. Net (directed set)) such that $ \lim\limits \| e _ {i} x - x \| = 0 $
for all $ x \in A $.
A right-approximate identity is similarly defined, using $ \| x e _ {i} - x \| $.
A left- and right-approximate identity is simply called an approximate identity. Every $ B ^ {*} $-
algebra has an approximate identity.
An algebra with involution $ E $
is also termed a symmetric algebra, and a total involution algebra is also called a completely symmetric algebra. Correspondingly, a homomorphism of algebras with involution $ \phi : E \rightarrow F $
is called a symmetric homomorphism if $ \phi ( x ^ {*} ) = \phi ( x ) ^ {*} $
for all $ x \in E $.
Unfortunately, the term symmetric algebra is also sometimes used to mean a total involution algebra.
A symmetric ideal of $ E $
is an ideal $ M $
such that $ M ^ {*} = \{ {m ^ {*} } : {m \in M } \} = M $.
References
| [a1] | W. Rudin, "Functional analysis" , McGraw-Hill (1979) |
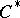 algebras" , North-Holland (1977) (Translated from French)
algebras" , North-Holland (1977) (Translated from French)