Hyperbolic metric, principle of the
Suppose that domains  and
and  lie in the
lie in the  -plane and
-plane and  -plane, respectively, and suppose they have at least three boundary points each; let
-plane, respectively, and suppose they have at least three boundary points each; let  be a holomorphic function in
be a holomorphic function in  taking values in
taking values in  , and let
, and let  and
and  be the line elements of the hyperbolic metric of
be the line elements of the hyperbolic metric of  and
and 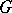 at points
at points  and
and 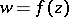 , respectively. The following inequality will then be true:
, respectively. The following inequality will then be true:
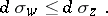 |
At any point 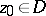 equality holds if
equality holds if  in
in 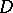 , where the function
, where the function  maps
maps 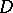 conformally onto the disc
conformally onto the disc  , while the function
, while the function  maps
maps 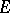 conformally onto
conformally onto 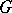 . The principle of the hyperbolic metric generalizes the Schwarz lemma to multiply-connected domains in which a hyperbolic metric can be defined.
. The principle of the hyperbolic metric generalizes the Schwarz lemma to multiply-connected domains in which a hyperbolic metric can be defined.
In formulating the principle of the hyperbolic metric it is permissible to replace the assumption on the analyticity of the function  in
in 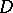 by a more general assumption, i.e. that
by a more general assumption, i.e. that 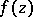 is an analytic function which is defined in
is an analytic function which is defined in 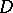 by any one of its elements and which can be analytically continued in
by any one of its elements and which can be analytically continued in 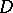 along any path.
along any path.
The same principle can also be formulated about the behaviour of the hyperbolic length of curves, the hyperbolic distance or the hyperbolic area for a given mapping. In fact, if  is a rectifiable curve in
is a rectifiable curve in  , then (for the meaning of the symbols see Hyperbolic metric)
, then (for the meaning of the symbols see Hyperbolic metric)
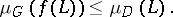 |
If 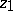 and
and 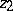 are two points in
are two points in 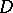 , then
, then
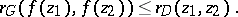 |
If 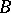 is a domain in
is a domain in 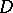 , then
, then
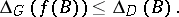 |
Equality in these inequalities holds only in the above-mentioned case.
The above result as applied to the hyperbolic distance shows that under the mapping 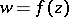 the image of the hyperbolic disc with centre at the point
the image of the hyperbolic disc with centre at the point  is contained in the hyperbolic disc with its centre at the point
is contained in the hyperbolic disc with its centre at the point 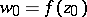 of the same hyperbolic radius.
of the same hyperbolic radius.
This result is a generalization to the case of multiply-connected domains of the following fact in the theory of conformal mapping (the invariant form of Schwarz' lemma): Under the mapping of the disc 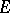 by a regular function
by a regular function
 |
in  , the hyperbolic distance between the images of the points
, the hyperbolic distance between the images of the points  and
and  of
of 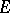 does not exceed the hyperbolic distance between
does not exceed the hyperbolic distance between 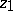 and
and 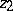 , and is equal to that distance only for a bilinear transformation of
, and is equal to that distance only for a bilinear transformation of 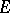 onto itself.
onto itself.
The principle of the hyperbolic metric is connected with the Lindelöf principle as follows. If the domains  and
and 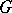 have a Green function and are simply connected, both these principles are identical. If
have a Green function and are simply connected, both these principles are identical. If 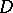 is simply connected, while
is simply connected, while 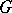 is multiply connected, the principle of the hyperbolic metric yields a more precise estimate of the domain containing the image of a hyperbolic disc in
is multiply connected, the principle of the hyperbolic metric yields a more precise estimate of the domain containing the image of a hyperbolic disc in  , defined by an inequality
, defined by an inequality  under the mapping
under the mapping 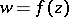 , where
, where 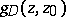 denotes the Green function of
denotes the Green function of  with logarithmic pole at
with logarithmic pole at  . The principle of the hyperbolic metric is also applicable to cases in which Lindelöf's principle does not apply — e.g. to domains having at least three boundary points but not having a Green function.
. The principle of the hyperbolic metric is also applicable to cases in which Lindelöf's principle does not apply — e.g. to domains having at least three boundary points but not having a Green function.
Comments
References
| [a1] | L.V. Ahlfors, "Conformal invariants. Topics in geometric function theory" , McGraw-Hill (1973) |
Hyperbolic metric, principle of the. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hyperbolic_metric,_principle_of_the&oldid=11504