Hardy spaces
real-variable theory of, real-variable 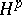 theory
theory
The real-variable Hardy spaces  (
( ) are spaces of distributions on
) are spaces of distributions on  (cf. Generalized functions, space of), originally defined as boundary values of holomorphic or harmonic functions, which have assumed an important role in modern harmonic analysis. They may be defined in terms of certain maximal functions.
(cf. Generalized functions, space of), originally defined as boundary values of holomorphic or harmonic functions, which have assumed an important role in modern harmonic analysis. They may be defined in terms of certain maximal functions.
Specifically, suppose  belongs to the Schwartz class
belongs to the Schwartz class  of rapidly decreasing smooth functions, and let
of rapidly decreasing smooth functions, and let  for
for  . If
. If 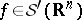 , the space of tempered distributions, define the radial maximal function
, the space of tempered distributions, define the radial maximal function  and the non-tangential maximal function
and the non-tangential maximal function 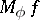 by
by
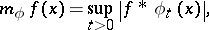 |
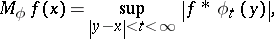 |
where  denotes convolution of functions. C. Fefferman and E.M. Stein [a2] (see also [a4]) proved that for
denotes convolution of functions. C. Fefferman and E.M. Stein [a2] (see also [a4]) proved that for 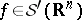 and
and  , the following conditions are equivalent (the Fefferman–Stein theorem):
, the following conditions are equivalent (the Fefferman–Stein theorem):
1)  for some
for some  with
with 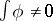 ;
;
2) 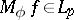 for some
for some 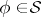 with
with 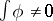 ;
;
3)  for every
for every 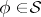 , and in fact
, and in fact 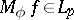 uniformly for
uniformly for  in a suitable bounded subset of
in a suitable bounded subset of 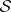 .
.  is the space of all
is the space of all  that satisfy these conditions.
that satisfy these conditions.
For  ,
,  coincides with
coincides with 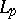 , and
, and  is a proper subspace of
is a proper subspace of  . For
. For 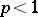 ,
,  contains distributions that are not functions. A distribution
contains distributions that are not functions. A distribution  on
on  is in
is in  if and only if
if and only if  is the boundary value of a harmonic function
is the boundary value of a harmonic function  on the upper half-plane such that
on the upper half-plane such that 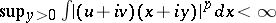 , where
, where  is the harmonic conjugate of
is the harmonic conjugate of  ; this is the connection with the complex-variable Hardy classes. There is a similar characterization of
; this is the connection with the complex-variable Hardy classes. There is a similar characterization of  for
for 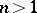 in terms of systems of harmonic functions satisfying generalized Cauchy–Riemann equations; see [a2].
in terms of systems of harmonic functions satisfying generalized Cauchy–Riemann equations; see [a2].
Another characterization of 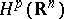 for
for 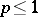 is of great importance. A measurable function
is of great importance. A measurable function 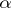 is called a
is called a  -atom (
-atom ( ) if
) if
i)  vanishes outside some ball of radius
vanishes outside some ball of radius 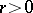 and
and 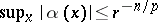 ;
;
ii) 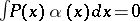 for all polynomials
for all polynomials  of degree
of degree 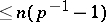 . The atomic decomposition theorem (see [a4]) states that
. The atomic decomposition theorem (see [a4]) states that  if and only if
if and only if 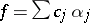 , where each
, where each 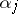 is a
is a  -atom and
-atom and  .
.
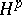 is a complete topological vector space for
is a complete topological vector space for 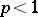 , and a Banach space for
, and a Banach space for  , with topology defined by any of the quasi-norms
, with topology defined by any of the quasi-norms  ,
, 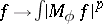 , or
, or
 |
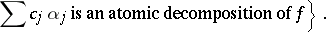 |
By the celebrated Fefferman theorem [a2] (see also [a4]), the dual of  is the space
is the space 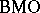 of functions of bounded mean oscillation (cf. also
of functions of bounded mean oscillation (cf. also  -space). For
-space). For 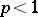 , the dual of
, the dual of 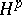 is the homogeneous Lipschitz space of order
is the homogeneous Lipschitz space of order  ; see [a3].
; see [a3].
The spaces 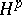 (
( ) and
) and  provide an extension of the scale of
provide an extension of the scale of 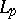 -spaces (
-spaces (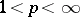 ) that is in many respects more natural and useful than the corresponding
) that is in many respects more natural and useful than the corresponding 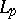 -spaces. Most importantly, many of the essential operations of harmonic analysis, e.g., singular integrals of Calderón–Zygmund type (cf. also Calderón–Zygmund operator; Singular integral), maximal operators and Littlewood–Paley functionals, that are well-behaved on
-spaces. Most importantly, many of the essential operations of harmonic analysis, e.g., singular integrals of Calderón–Zygmund type (cf. also Calderón–Zygmund operator; Singular integral), maximal operators and Littlewood–Paley functionals, that are well-behaved on 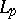 only for
only for 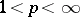 are also well-behaved on
are also well-behaved on 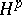 and
and 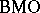 . In addition, many important classes of singular distributions belong to
. In addition, many important classes of singular distributions belong to  , or are closely related to elements of
, or are closely related to elements of  , for suitable
, for suitable  . See [a3], [a4].
. See [a3], [a4].
The real-variable  theory can be extended to spaces other than
theory can be extended to spaces other than  . A rather complete extension is available in the setting of homogeneous groups, i.e., simply-connected nilpotent Lie groups (cf. also Lie group, nilpotent) with a one-parameter family of dilations; see [a3]. (These groups include, in particular,
. A rather complete extension is available in the setting of homogeneous groups, i.e., simply-connected nilpotent Lie groups (cf. also Lie group, nilpotent) with a one-parameter family of dilations; see [a3]. (These groups include, in particular, 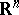 with non-isotropic dilations.) Parts of the theory have also been developed in the much more general setting of the Coifman–Weiss spaces of homogeneous type; see [a1].
with non-isotropic dilations.) Parts of the theory have also been developed in the much more general setting of the Coifman–Weiss spaces of homogeneous type; see [a1].
References
| [a1] | R.R. Coifman, G. Weiss, "Extensions of Hardy spaces and their use in analysis" Bull. Amer. Math. Soc. , 83 (1977) pp. 569–645 |
| [a2] | C. Fefferman, E.M. Stein, "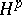 spaces of several variables" Acta Math. , 129 (1972) pp. 137–193 spaces of several variables" Acta Math. , 129 (1972) pp. 137–193 |
| [a3] | G.B. Folland, E.M. Stein, "Hardy spaces on homogeneous groups" , Princeton Univ. Press (1982) |
| [a4] | E.M. Stein, "Harmonic analysis" , Princeton Univ. Press (1993) |
Hardy spaces. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hardy_spaces&oldid=13621