Gromov hyperbolic space
hyperbolic space in the sense of Gromov
This notion provides a uniform "global" approach to such objects as the hyperbolic plane, simply-connected Riemannian manifolds with pinched negative sectional curvature,  -spaces, and metric trees. Various "hyperbolic properties" introduced earlier (mostly in the context of group theory) [a1], [a2], [a3] were summed up and further developed by M. Gromov in his seminal paper [a4]. More detailed expositions of (some parts of) Gromov's work can be found in [a5] and [a6].
-spaces, and metric trees. Various "hyperbolic properties" introduced earlier (mostly in the context of group theory) [a1], [a2], [a3] were summed up and further developed by M. Gromov in his seminal paper [a4]. More detailed expositions of (some parts of) Gromov's work can be found in [a5] and [a6].
If  is a metric space, then the Gromov product of two points
is a metric space, then the Gromov product of two points  with respect to a reference point
with respect to a reference point  is defined as
is defined as
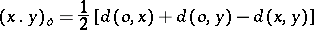 |
(when  is a tree, this product coincides with the confluent of
is a tree, this product coincides with the confluent of  and
and  , i.e., the length of the common part of the geodesic segments
, i.e., the length of the common part of the geodesic segments  and
and  ). The space
). The space 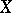 is called
is called  -hyperbolic (with a constant
-hyperbolic (with a constant  ) if the Gromov product satisfies the
) if the Gromov product satisfies the  -ultrametric inequality
-ultrametric inequality
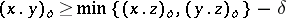 |
for any  points
points  . If
. If  is
is  -hyperbolic for some
-hyperbolic for some  , it is called Gromov hyperbolic. Any metric tree is
, it is called Gromov hyperbolic. Any metric tree is  -hyperbolic, and, conversely, any
-hyperbolic, and, conversely, any  -hyperbolic space isometrically imbeds into a tree. For Cartan–Hadamard manifolds, hyperbolicity is equivalent to the strong visibility property [a7].
-hyperbolic space isometrically imbeds into a tree. For Cartan–Hadamard manifolds, hyperbolicity is equivalent to the strong visibility property [a7].
Often one adds to the definition of Gromov hyperbolicity the following two requirements: 1) 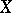 is geodesic (cf. also Geodesic manifold), i.e., any two points in
is geodesic (cf. also Geodesic manifold), i.e., any two points in  can be joined by a path (a geodesic) whose length coincides with the distance between these points; and 2)
can be joined by a path (a geodesic) whose length coincides with the distance between these points; and 2)  is proper, i.e., all metric balls in
is proper, i.e., all metric balls in  are compact. Then hyperbolicity is equivalent to the following thin triangles condition: there exists a constant
are compact. Then hyperbolicity is equivalent to the following thin triangles condition: there exists a constant  such that for any geodesic triangle in
such that for any geodesic triangle in 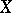 one can choose a point on each of the sides of the triangle in such a way that the pairwise distances between these
one can choose a point on each of the sides of the triangle in such a way that the pairwise distances between these  points are all not greater than
points are all not greater than 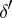 .
.
Yet another definition of hyperbolicity can be given in terms of exponential divergence of geodesic rays in  , cf. [a8].
, cf. [a8].
A sequence  in
in 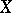 is called convergent if
is called convergent if  . The hyperbolic boundary
. The hyperbolic boundary  of a hyperbolic space
of a hyperbolic space  is defined as the set of equivalence classes of convergent sequences with respect to the equivalence relation
is defined as the set of equivalence classes of convergent sequences with respect to the equivalence relation  . Any geodesic ray in
. Any geodesic ray in  is convergent, and, conversely, for any class
is convergent, and, conversely, for any class  and any point
and any point  there exists a geodesic ray (not necessarily unique!) joining
there exists a geodesic ray (not necessarily unique!) joining  and
and  , i.e., starting from
, i.e., starting from  and belonging to the class
and belonging to the class  . Equivalently,
. Equivalently, 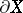 can be defined as the set of asymptotic classes of geodesic rays, with two rays being asymptotic if they are within bounded distance from each other.
can be defined as the set of asymptotic classes of geodesic rays, with two rays being asymptotic if they are within bounded distance from each other.
The hyperbolic boundary is the boundary of the hyperbolic compactification of  . For trees the hyperbolic compactification coincides with the end compactification, and for hyperbolic Cartan–Hadamard manifolds with the visibility compactification. The Gromov product naturally extends to
. For trees the hyperbolic compactification coincides with the end compactification, and for hyperbolic Cartan–Hadamard manifolds with the visibility compactification. The Gromov product naturally extends to  , and
, and  , for sufficiently small
, for sufficiently small  , is uniformly equivalent to a metric on
, is uniformly equivalent to a metric on  .
.
Hyperbolicity of a metric space 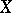 is determined by its "global" structure only. If
is determined by its "global" structure only. If  and
and  are two metric spaces such that there exist mappings
are two metric spaces such that there exist mappings  and
and  and a constant
and a constant 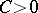 with the property that
with the property that  and
and  for all
for all  , and
, and  and
and  for all
for all  (such metric spaces are called roughly isometric), then
(such metric spaces are called roughly isometric), then  and
and  are hyperbolic simultaneously, and the hyperbolic boundaries
are hyperbolic simultaneously, and the hyperbolic boundaries 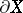 and
and  are homeomorphic.
are homeomorphic.
Isometries of hyperbolic spaces can be classified into elliptic, parabolic and hyperbolic ones in the same way as isometries of the usual hyperbolic plane. An important class of hyperbolic spaces is provided by finitely generated groups whose Cayley graphs are Gromov hyperbolic spaces (such groups are called word hyperbolic).
See also Hyperbolic group.
References
| [a1] | E. Rips, "Subgroups of small cancellation groups" Bull. London Math. Soc. , 14 (1982) pp. 45–47 |
| [a2] | M. Gromov, "Infinite groups as geometric objects" , Proc. Int. Congress Math. Warszawa, 1983 , 1 (1984) pp. 385–391 |
| [a3] | J. Cannon, "The combinatorial structure of cocompact discrete hyperbolic groups" Geom. Dedicata , 16 (1984) pp. 123–148 |
| [a4] | M. Gromov, "Hyperbolic groups" S.M. Gersten (ed.) , Essays in Group Theory , MSRI Publ. , 8 , Springer (1987) pp. 75–263 |
| [a5] | "Sur les groupes hyperboliques d'après Mikhael Gromov" E. Ghys (ed.) P. de la Harpe (ed.) , Progress in Maths. , 83 , Birkhäuser (1990) |
| [a6] | M. Coornaert, T. Delzant, A. Papadopoulos, "Géométrie et théorie des groupes" , Lecture Notes in Mathematics , 1441 , Springer (1990) |
| [a7] | V.A. Kaimanovich, "Ergodicity of harmonic invariant measures for the geodesic flow on hyperbolic spaces" J. Reine Angew. Math. , 455 (1994) pp. 57–103 |
| [a8] | J. Cannon, "The theory of negatively curved spaces and groups" T. Bedford (ed.) M. Keane (ed.) C. Ser. (ed.) , Ergodic Theory, Symbolic Dynamics and Hyperbolic Spaces , Oxford Univ. Press (1991) pp. 315–369 |
Gromov hyperbolic space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gromov_hyperbolic_space&oldid=17648