Gorenstein ring
A commutative local Noetherian ring of finite injective dimension (cf. Homological dimension). A local ring  with a maximal ideal
with a maximal ideal  and residue field
and residue field  of dimension
of dimension  is a Gorenstein ring if and only if one of the following equivalent conditions is satisfied:
is a Gorenstein ring if and only if one of the following equivalent conditions is satisfied:
1)  for
for  and
and  .
.
2) For any maximal  -sequence
-sequence  (cf. Depth of a module) the ideal
(cf. Depth of a module) the ideal 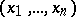 is irreducible.
is irreducible.
3) The functor 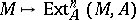 , defined on the category of
, defined on the category of 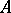 -modules of finite length, is isomorphic to the functor
-modules of finite length, is isomorphic to the functor  , where
, where 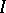 is the injective envelope of
is the injective envelope of  .
.
4) The ring 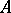 is a Cohen–Macaulay ring (in particular, all local cohomology groups
is a Cohen–Macaulay ring (in particular, all local cohomology groups  for
for  ), and
), and  coincides with the injective envelope of
coincides with the injective envelope of  .
.
5) For any  -module
-module 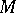 of finite type there exists a canonical isomorphism
of finite type there exists a canonical isomorphism
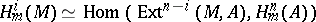 |
(local duality).
Examples of Gorenstein rings include regular rings and also their quotient rings by an ideal generated by a regular sequence of elements (complete intersections).
If a Gorenstein ring  is a one-dimensional integral domain, then this ring has the following numerical characterization. Let
is a one-dimensional integral domain, then this ring has the following numerical characterization. Let 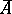 be the integral closure of
be the integral closure of  in its field of fractions, let
in its field of fractions, let  be the conductor (cf. Conductor of an integral closure) of
be the conductor (cf. Conductor of an integral closure) of  in
in 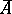 , let
, let  , and let
, and let  . The ring
. The ring 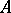 is then a Gorenstein ring if and only if
is then a Gorenstein ring if and only if  . This equality was first demonstrated by D. Gorenstein [1] for the local ring of an irreducible plane algebraic curve. A localization of a Gorenstein ring is a Gorenstein ring. In this connection an extension of the concept of a Gorenstein ring arose: A Noetherian ring (or scheme) is said to be a Gorenstein ring (scheme) if all the localizations of this ring by prime ideals (or, correspondingly, all local rings of the scheme) are local Gorenstein rings (in the former definition).
. This equality was first demonstrated by D. Gorenstein [1] for the local ring of an irreducible plane algebraic curve. A localization of a Gorenstein ring is a Gorenstein ring. In this connection an extension of the concept of a Gorenstein ring arose: A Noetherian ring (or scheme) is said to be a Gorenstein ring (scheme) if all the localizations of this ring by prime ideals (or, correspondingly, all local rings of the scheme) are local Gorenstein rings (in the former definition).
References
| [1] | D. Gorenstein, "An arithmetic theory of adjoint plane curves" Trans. Amer. Math. Soc. , 72 (1952) pp. 414–436 |
| [2] | J.-P. Serre, "Groupes algébrique et corps des classes" , Hermann (1959) |
| [3] | L.L. Abramov, E.S. Golod, "Homology algebra of the Koszul complex of a local Gorenstein ring" Math. Notes , 9 : 1 (1971) pp. 30–32 Mat. Zametki , 9 : 1 (1971) pp. 53–58 |
| [4] | A. Grothendieck, "Géométrie formelle et géométrie algébrique" Sem. Bourbaki , 11 (1958–1959) |
| [5] | R. Hartshorne, "Local cohomology, a seminar given by A. Grothendieck" , Springer (1967) |
| [6] | R. Hartshorne, "Residues and duality" , Springer (1966) |
| [7] | H. Bass, "On the ubiquity of Gorenstein rings" Math. Z. , 82 (1963) pp. 8–28 |
Gorenstein ring. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gorenstein_ring&oldid=18502