Gell-Mann formula
A prescription for constructing anti-Hermitian representations of a symmetric Lie algebra (over the real numbers)  from representations of an Inönü–Wigner contraction
from representations of an Inönü–Wigner contraction 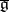 . One assumes that
. One assumes that 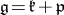 is a direct sum of vector spaces and
is a direct sum of vector spaces and
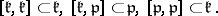 |
Then 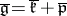 and there exists an isomorphism of vector spaces
and there exists an isomorphism of vector spaces 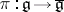 such that
such that  ,
, 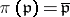 and
and 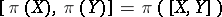 for all
for all 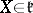 ,
,  . In addition, one has
. In addition, one has 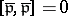 .
.
The best studied examples concern the (pseudo-) orthogonal algebras, when 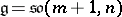 or
or 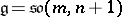 and
and  [a1], [a2]. Then
[a1], [a2]. Then  is an inhomogeneous Lie algebra with
is an inhomogeneous Lie algebra with 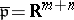 . Let
. Let 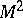 be the quadratic
be the quadratic 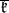 -invariant element from the symmetric algebra of
-invariant element from the symmetric algebra of 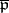 . If
. If 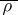 is an anti-Hermitian representation of
is an anti-Hermitian representation of  such that
such that 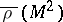 is a multiple of the unit operator, then the formula for the representation
is a multiple of the unit operator, then the formula for the representation  of
of 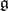 reads:
reads:  for all
for all  , and, for all
, and, for all 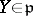 ,
,
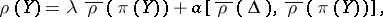 |
where 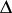 is the second-degree Casimir element from the universal enveloping algebra of
is the second-degree Casimir element from the universal enveloping algebra of 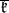 while
while 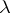 and
and  are parameters. Here,
are parameters. Here, 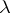 is real and arbitrary and
is real and arbitrary and  is pure imaginary and depends on
is pure imaginary and depends on 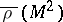 .
.
References
| [a1] | E. Weimar, "The range of validity of the Gell-Mann formula" Nuovo Cim. Lett. , 4 (1972) pp. 43–50 |
| [a2] | R. Hermann, "Lie groups for physicists" , Benjamin (1966) |
Gell-Mann formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gell-Mann_formula&oldid=11628