Differential equation, partial, of the second order

An equation containing at least one derivative of the second order of the unknown function and not containing derivatives of higher orders. For instance, a linear equation of the second order has the form

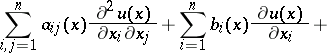(1)

where the point 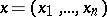belongs to some domain 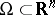in which the real-valued functions , and 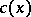are defined, and at each point 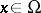at least one of the coefficients 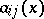is non-zero. For any point there exists a non-singular transformation of the independent variables 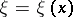such that equation (1) assumes the following form in the new coordinates :

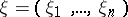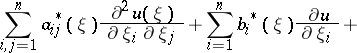(2)

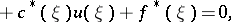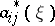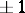where the coefficients at the point are equal to zero if and are equal to or to zero if . Equation (2) is known as the canonical form of equation (1) at the point .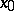

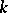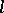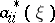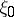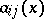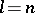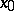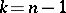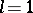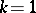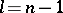The number and the number of coefficients in equation (2) which are, respectively, positive and negative at the point depend only on the coefficients of equation (1). As a consequence, differential equations (1) can be classified as follows. If or , equation (1) is called elliptic at the point ; if and , or if and , it is called hyperbolic; if 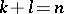and 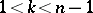, it is called ultra-hyperbolic. The equation is called parabolic in the wide sense at the point 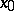if at least one of the coefficients 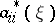is zero at the point 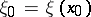and ; it is called parabolic at the point 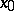if only one of the coefficients 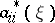is zero at the point 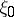(say 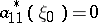), while all the remaining coefficients 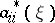have the same sign and the coefficient .

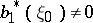In the case of two independent variables 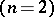it is more convenient to define the type of an equation by the function

Thus, equation (1) is elliptic at the point 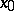if 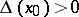; it is hyperbolic if 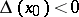and is parabolic in the wide sense if .

An equation is called elliptic, hyperbolic, etc., in a domain, if it is, respectively, elliptic, hyperbolic, etc., at each point of this domain. For instance, the Tricomi equation 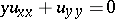is elliptic if 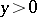; it is hyperbolic if 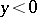; and it is parabolic in the wide sense if .

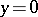The transformation of variables which converts equation (1) to canonical form at the point 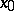depends on that point. If there are three or more independent variables, there is, in general, no non-singular transformation of equation (1) to canonical form at all points of some neighbourhood of the point 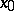at the same time, i.e. to the form

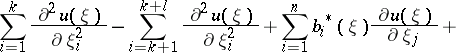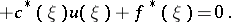In the case of two independent variables (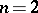), on the other hand, it is possible to bring equation (1) to canonical form by imposing certain conditions on the coefficients 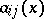; as an example, the functions 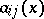must be continuously differentiable up to the second order inclusive, and equation (1) must be of one type in a certain neighbourhood of the point .

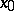Let

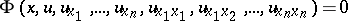(3)

be a non-linear equation of the second order, where 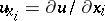, , 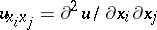and let the derivatives 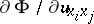exist at each point in the domain of definition of the real-valued function 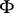; further, let the condition

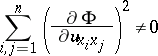be satisfied. In the classification of non-linear equations of the type (3) one determines a certain solution 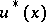of this equation and one considers the linear equation

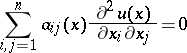(4)

with coefficients

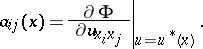For a given solution 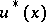, equation (3) is said to be elliptic, hyperbolic, etc., at a point 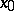(or in a domain) if equation (4) is elliptic, hyperbolic, etc., respectively, at this point (or in this domain).

A very wide class of physical problems is reduced to solving differential equations of the second order. See, for example, Wave equation; Telegraph equation; Thermal-conductance equation; Tricomi equation; Laplace equation; Poisson equation; Helmholtz equation.

Comments

See also Differential equation, partial.