Density of a set
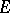 that is measurable on the real line
that is measurable on the real line 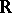 , at a point
, at a point 
The limit (if it exists) of the ratio
 | (1) |
where 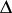 is any segment containing
is any segment containing  and
and 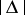 is its length. If one considers an outer measure instead of a measure, one obtains the definition of the outer density of
is its length. If one considers an outer measure instead of a measure, one obtains the definition of the outer density of 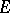 at
at  . Similarly one can introduce the density in
. Similarly one can introduce the density in  -dimensional space. Here the lengths of the segments in
-dimensional space. Here the lengths of the segments in 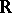 are replaced by the volumes of the corresponding
are replaced by the volumes of the corresponding  -dimensional parallelepipeds with faces parallel to the coordinate planes, while the limit is considered as the diameters of the parallelepipeds tend to zero. For sets from
-dimensional parallelepipeds with faces parallel to the coordinate planes, while the limit is considered as the diameters of the parallelepipeds tend to zero. For sets from  it is useful to employ the concept of the right (left) density of a set
it is useful to employ the concept of the right (left) density of a set 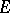 at a point
at a point  , which is obtained from the general definition if in it one considers only segments
, which is obtained from the general definition if in it one considers only segments  having left (right) ends at
having left (right) ends at  . Very often, the concept of density is used when the density of the set is equal to one (see Density point) or zero (see Thinness of a set).
. Very often, the concept of density is used when the density of the set is equal to one (see Density point) or zero (see Thinness of a set).
References
| [1] | I.P. Natanson, "Theorie der Funktionen einer reellen Veränderlichen" , H. Deutsch , Frankfurt a.M. (1961) (Translated from Russian) |
| [2] | S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French) |
Comments
See [a1] for a nice topological application of these notions.
References
| [a1] | F.D. Tall, "The density topology" Pacific J. Math , 62 (1976) pp. 275–284 |
Density of a set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Density_of_a_set&oldid=18377