Conjugate harmonic functions
harmonically-conjugate functions
A pair of real harmonic functions  and
and  which are the real and imaginary parts of some analytic function
which are the real and imaginary parts of some analytic function 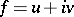 of a complex variable. In the case of one complex variable
of a complex variable. In the case of one complex variable  , two harmonic functions
, two harmonic functions 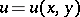 and
and 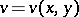 are conjugate in a domain
are conjugate in a domain 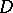 of the complex plane
of the complex plane 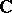 if and only if they satisfy the Cauchy–Riemann equations in
if and only if they satisfy the Cauchy–Riemann equations in 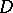 :
:
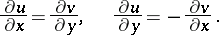 | (1) |
The roles of  and
and  in (1) are not symmetric:
in (1) are not symmetric:  is a conjugate for
is a conjugate for  but
but 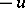 , and not
, and not  , is a conjugate for
, is a conjugate for  . Given a harmonic function
. Given a harmonic function  , a local conjugate
, a local conjugate  and a local complete analytic function
and a local complete analytic function 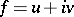 are easily determined up to a constant term. This can be done, for example, using the Goursat formula
are easily determined up to a constant term. This can be done, for example, using the Goursat formula
 | (2) |
in a neighbourhood of some point  in the domain of definition of
in the domain of definition of  .
.
In the case of several complex variables 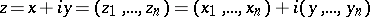 ,
,  , the Cauchy–Riemann system becomes overdetermined
, the Cauchy–Riemann system becomes overdetermined
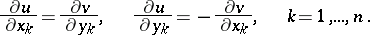 | (3) |
It follows from (3) that for 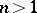 ,
,  can no longer be taken as an arbitrary harmonic function; it must belong to the subclass of pluriharmonic functions (cf. Pluriharmonic function). The conjugate pluriharmonic function
can no longer be taken as an arbitrary harmonic function; it must belong to the subclass of pluriharmonic functions (cf. Pluriharmonic function). The conjugate pluriharmonic function  can then be found using (2).
can then be found using (2).
There are various analogues of conjugate harmonic functions 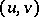 involving a vector function
involving a vector function 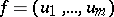 whose components
whose components  are real functions of real variables
are real functions of real variables  . An example is a gradient system
. An example is a gradient system 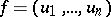 satisfying the generalized system of Cauchy–Riemann equations
satisfying the generalized system of Cauchy–Riemann equations
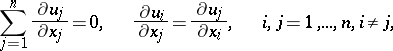 | (4) |
which can also be written in abbreviated form:
 |
If the conditions (4) hold in a domain  of a Euclidean space
of a Euclidean space 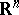 homeomorphic to a ball, then there is a harmonic function
homeomorphic to a ball, then there is a harmonic function 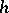 on
on 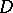 such that
such that  . When
. When 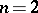 , it turns out that
, it turns out that 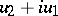 is an analytic function of the variable
is an analytic function of the variable 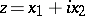 . The behaviour of the solutions of (4) is in some respects similar to that of the Cauchy–Riemann system (1), for example in the study of boundary properties (see [3]).
. The behaviour of the solutions of (4) is in some respects similar to that of the Cauchy–Riemann system (1), for example in the study of boundary properties (see [3]).
References
| [1] | A.V. Bitsadze, "Fundamentals of the theory of analytic functions of a complex variable" , Moscow (1972) (In Russian) |
| [2] | V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian) |
| [3] | E.M. Stein, G. Weiss, "Introduction to Fourier analysis on Euclidean spaces" , Princeton Univ. Press (1971) |
Conjugate harmonic functions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conjugate_harmonic_functions&oldid=17885