Cohomology of Banach algebras
The groups  ,
,  , where
, where  is a two-sided Banach module over a Banach algebra
is a two-sided Banach module over a Banach algebra  , defined as the cohomology groups of the cochain complex
, defined as the cohomology groups of the cochain complex
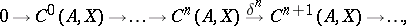 |
the  -dimensional chains of which are the continuous
-dimensional chains of which are the continuous  -linear operators from
-linear operators from  into
into  , and
, and
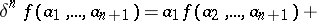 |
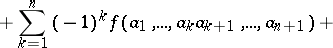 |
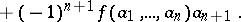 |
The cohomology of Banach algebras can also be introduced via a Banach analogue of the functor  , and there is also an axiomatic definition.
, and there is also an axiomatic definition.
Analogously to the cohomology of algebras, the elements of the one-dimensional cohomology group  of a Banach algebra can be interpreted as continuous derivations from
of a Banach algebra can be interpreted as continuous derivations from  into
into  modulo inner derivations, and the elements of the two-dimensional cohomology group can be interpreted as equivalence classes of extensions of
modulo inner derivations, and the elements of the two-dimensional cohomology group can be interpreted as equivalence classes of extensions of  by
by  in which
in which  is complemented. At the same time a number of specific analytic and topological concepts can be expressed in the language of cohomology of Banach algebras.
is complemented. At the same time a number of specific analytic and topological concepts can be expressed in the language of cohomology of Banach algebras.
An algebra  such that
such that  for all
for all 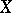 is said to be completely separable; these algebras are characterized by the fact that all their extensions split. The specific character of Banach structures is reflected by the fact that such a requirement is very rigid: A completely-separable commutative Banach algebra necessarily has finite spectrum (space of maximal ideals). In particular, a completely-separable function algebra is the direct sum of finitely many copies of
is said to be completely separable; these algebras are characterized by the fact that all their extensions split. The specific character of Banach structures is reflected by the fact that such a requirement is very rigid: A completely-separable commutative Banach algebra necessarily has finite spectrum (space of maximal ideals). In particular, a completely-separable function algebra is the direct sum of finitely many copies of 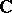 (the field of complex numbers).
(the field of complex numbers).
The class of Banach algebras with trivial cohomology in higher dimension  is not so restricted; it contains, e.g., the class of biprojective algebras, i.e. algebras
is not so restricted; it contains, e.g., the class of biprojective algebras, i.e. algebras 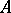 that are projective as two-sided Banach
that are projective as two-sided Banach  -modules. The
-modules. The  -algebra and the
-algebra and the  -algebra of a compact group are biprojective, as are the algebras of nuclear operators in all classical Banach spaces. Under certain conditions on the Banach structure, topologically-simple biprojective algebras can be characterized completely, and every semi-simple biprojective algebra is a topological direct sum of such algebras.
-algebra of a compact group are biprojective, as are the algebras of nuclear operators in all classical Banach spaces. Under certain conditions on the Banach structure, topologically-simple biprojective algebras can be characterized completely, and every semi-simple biprojective algebra is a topological direct sum of such algebras.
A commutative algebra is said to be weakly hereditary if its maximal ideals are projective. This property is equivalent to the triviality of  for those
for those  for which
for which 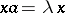 for all
for all 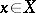 ,
,  . For an ideal in a commutative Banach algebra
. For an ideal in a commutative Banach algebra  to be projective it is necessary that its spectrum be paracompact. If
to be projective it is necessary that its spectrum be paracompact. If  this condition is also sufficient. In particular,
this condition is also sufficient. In particular,  is weakly hereditary if and only if all sets of the form
is weakly hereditary if and only if all sets of the form  ,
,  , are paracompact.
, are paracompact.
The space dual to a two-sided  -module
-module  is itself a two-sided
is itself a two-sided  -module. Algebras with
-module. Algebras with  for all
for all  and
and  are called amenable, since for
are called amenable, since for  this property is equivalent to the amenability (averageability) of
this property is equivalent to the amenability (averageability) of  . In general,
. In general,  is amenable if and only if the algebra
is amenable if and only if the algebra
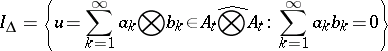 |
has a bounded approximate identity.
References
| [1] | B.E. Johnson, "Cohomology of Banach algebras" Mem. Amer. Math. Soc. , 127 (1972) |
| [2] | A.Ya. Khelemskii, "Lower values that admit the global homological dimension of Banach function algebras" Trudy Sem. Petrovsk. : 3 (1978) pp. 223–242 (In Russian) |
Comments
References
| [a1] | A.Ya. [A.Ya. Khelemskii] Helemsky, "Cohomology of Banach and topological spaces" , Reidel (Forthcoming) (Translated from Russian) |
Cohomology of Banach algebras. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cohomology_of_Banach_algebras&oldid=15856