Algebra of measures

measure algebra

The algebra 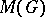of complex-valued regular Borel measures on a locally compact Abelian group that have bounded variation, with the ordinary linear operations and with convolution as multiplication (cf. Harmonic analysis, abstract). The convolution of the measures is completely defined by the condition that, for any continuous function on with compact support,

If the total variation of a measure is taken as norm, 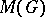becomes a commutative Banach algebra over the field of complex numbers. The algebra of measures has a unit which is the 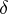-measure located at the zero of the group. The set of discrete measures contained in 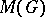forms a closed subalgebra.

To each function 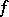which belongs to the group algebra 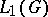may be assigned a corresponding measure 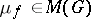in accordance with the rule

(integral with respect to the Haar measure). The result is an isometric isomorphic imbedding 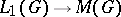. Under this imbedding the image is a closed ideal in .

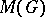The Fourier–Stieltjes transform of a measure 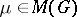is the function 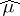on the dual group 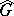defined by the formula

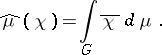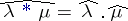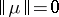Then and if 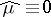. In particular, 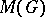is an algebra without a radical.

If the group 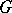is not discrete, then the structure of the measure algebra 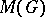is extremely complicated: It is not symmetric and its space of maximal ideals has a number of pathological properties. For instance, this space contains infinite-dimensional analytic sets, and the naturally imbedded group 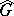in it is not dense even in the Shilov boundary. Nevertheless, the idempotent measures, i.e. measures for which , are known. Each idempotent measure is a finite integer combination 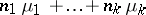, where 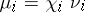, and where 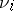is the Haar measure of a compact subgroup, and 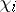is a character. In the case 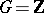this means that a sequence 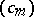of zeros and ones is the Fourier–Stieltjes transform of some measure on the circle if and only if 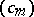differs from a periodic sequence by not more than a finite number of terms.

In the general case the theorem on idempotent measures can be naturally interpreted in terms of the cohomology spaces of dimension zero of the space of maximal ideals. A satisfactory description is also known for other cohomology groups of the space of maximal ideals of the measure algebra. This makes it possible to tell, in particular, if a logarithm of an invertible measure from 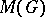can be taken (one-dimensional integral cohomology).

References

[1]	W. Rudin, "Fourier analysis on groups" , Interscience (1962)
[2]	J.L. Taylor, "The cohomology of the spectrum of a measure algebra" Acta Math. , 126 (1971) pp. 195–225

References

[a1]	C.C. Graham, O.C. McGehee, "Essays in commutative harmonic analysis" , Springer (1979) pp. Chapt. 5
[a2]	J.L. Taylor, "Measure algebras" , Amer. Math. Soc. (1972)