Putnam-Fuglede theorems
Fuglede–Putnam theorems, Berberian–Putnam–Fuglede theorems
Let $H$ denote a Hilbert space, $B ( H )$ the algebra of operators on $H$ (i.e., bounded linear transformations; cf. Linear transformation; Operator), $\delta _ { A , B } : B ( H ) \rightarrow B ( H )$ the derivation $\delta _ { A , B } ( X ) = A X - X B$ ($\delta _ { A , A } = \delta _ { A }$; cf. also Derivation in a ring) and let $\operatorname{ker} \delta _ { A , B } = \{ X \in B ( H ) : \delta _ { A , B } ( X ) = 0 \}$. If $\operatorname { ker }\delta _ { A } \subseteq \operatorname { ker } \delta _ { A ^*}$, then $A$ is normal (cf. Normal operator; simply choose $X = A$ in $\delta _ { A } ( X )$). The question whether the converse assertion, namely "Is kerdAkerdA* for normal A?" , also holds was raised by J. von Neumann in 1942, and answered in the affirmative in 1950 by B. Fuglede [a7], p. 349, $\#$45. C.R. Putnam extended the Fuglede theorem to $\operatorname { ker } \delta _ { A , B } \subseteq \operatorname { ker } \delta _ { A^* , B^* }$, for normal $A$ and $B$ [a7], p. 352, $\#$109, and a beautiful proof of the Putnam–Fuglede theorem was given by M. Rosenblum [a7], p. 352, $\#$118. Introducing the trick of considering the operators $\hat { A } = A \oplus B$ and $\hat { X } = ( A , B )$ on $\widehat { H } = H \oplus H$, S.K. Berberian [a7], p. 347, $\#$9, showed that the Putnam–Fuglede theorem indeed follows from the Fuglede theorem. For this reason, Putnam–Fuglede theorems are sometimes also referred to as Berberian–Putnam–Fuglede theorems.
The Putnam–Fuglede theorem, namely "kerdA,BkerdA*,B* for normal A and B" , has since been considered in a large number of papers, and various generalizations of it have appeared over the past four decades. Broadly speaking, these generalizations fall into the following four types:
i) where the normality is replaced by a weaker requirement, such as subnormality or $p$-hyponormality;
ii) asymptotic Putnam–Fuglede theorems;
iii) Putnam–Fuglede theorems modulo (proper, two-sided) ideals of $B ( H )$; and
iv) Putnam–Fuglede theorems in a $B$-space setting. Before briefly examining some of these, note that there exist subnormal operators $A$ and $B$ for which $\operatorname { ker } \delta _ { A , B } \nsubseteq \operatorname { ker } \delta _ { A ^ { * } , B ^ { * }}$ [a7], p. 107. This implies that in any generalization of the Putnam–Fuglede theorem to a wider class of operators, the hypotheses on $A$ and $B$ are not symmetric (and that it is more appropriate to think of $A$ and $B ^ { * }$ as being normal in the Putnam–Fuglede theorem).
Asymmetric Putnam–Fuglede theorems.
If $A$ and $B ^ { * }$ are subnormal operators with normal extensions 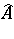 and $\widehat { B^* } $ on $\widehat { H } = H \oplus H$ (say) and $X \in \operatorname { ker } \delta _ { A , B }$, then $\hat { X } = X \oplus 0 \in \operatorname { ker } \delta _ { \hat{A} , B }$, and it follows that $\hat { X } \in \operatorname { ker } \delta _ { \hat { A } ^ { * } , B ^ { * }}$ and $X \in \operatorname{ker} \delta _ { A^ * , B ^*}$. This asymmetric extension of the Putnam–Fuglede theorem was proved by T. Furuta [a6] (though an avatar of this result had already appeared in [a10]). Following a lot of activity during the 1970s and the 1980s ([a2], [a5], [a6], [a9] list some of the references), it is now (1998) known that $\operatorname { ker } \delta _ { A , B } \subseteq \operatorname { ker } \delta _ { A^* , B^* }$ for $A$ and $B ^ { * }$ belonging to a large number of suitably paired classes of operators, amongst them $p$-hyponormal ($0 < p \leq 1$), $M$-hyponormal, dominant and $k$-quasi-hyponormal classes [a5].
and $\widehat { B^* } $ on $\widehat { H } = H \oplus H$ (say) and $X \in \operatorname { ker } \delta _ { A , B }$, then $\hat { X } = X \oplus 0 \in \operatorname { ker } \delta _ { \hat{A} , B }$, and it follows that $\hat { X } \in \operatorname { ker } \delta _ { \hat { A } ^ { * } , B ^ { * }}$ and $X \in \operatorname{ker} \delta _ { A^ * , B ^*}$. This asymmetric extension of the Putnam–Fuglede theorem was proved by T. Furuta [a6] (though an avatar of this result had already appeared in [a10]). Following a lot of activity during the 1970s and the 1980s ([a2], [a5], [a6], [a9] list some of the references), it is now (1998) known that $\operatorname { ker } \delta _ { A , B } \subseteq \operatorname { ker } \delta _ { A^* , B^* }$ for $A$ and $B ^ { * }$ belonging to a large number of suitably paired classes of operators, amongst them $p$-hyponormal ($0 < p \leq 1$), $M$-hyponormal, dominant and $k$-quasi-hyponormal classes [a5].
Asymptotic Putnam–Fuglede theorems.
Given normal $A$ and $B$, and a neighbourhood $\mathcal{N} _ { \epsilon}$ of $0$ in some topology (weak operator, strong operator or uniform), does there exist a neighbourhood $\mathcal N _ { \epsilon } ^ { \prime }$ of $0$ in the same topology such that $\delta _ { A , B } ( X ) \in \mathcal{N} _ { \epsilon } ^ { \prime } \Rightarrow \delta _ { A ^ { * } , B ^ { * } } ( X ) \in \mathcal{N}_ { \epsilon }$? The answer to this question is (in general) no, for there exists a normal $A$ and a (non-uniformly bounded) sequence $\{ X _ { n } \}$ of operators such that $\| \delta _ { A } ( X _ { n } ) \| \rightarrow 0$ but $\| \delta _ { A } * ( X _ { n } ) \| \geq 1$ for all $n$ [a8]. If, however, the sequence $\{ X _ { n } \}$ is uniformly bounded, then the answer is in the affirmative for normal (and subnormal) $A$ and $B ^ { * }$ [a2] (and indeed, if one limits oneself to the uniform topology, for a number of classes of operators [a5], [a9]).
Putnam–Fuglede theorems modulo ideals.
Say that the Putnam–Fuglede theorem holds modulo an ideal $I$ if, given normal operators $A$ and $B$, $\delta _ { A , B } ( X ) \in I$ implies $\delta _ { A ^ * , B^ *} ( X ) \in I$ for all $X \in B ( H )$. The Putnam–Fuglede theorem holds modulo the compacts (simply consider the Putnam–Fuglede theorem in the Calkin algebra), and does not hold modulo the ideal of finite-rank operators. In a remarkable extension of the Putnam–Fuglede theorem to Schatten-von Neumann ideals $\mathcal{C} _ { p }$, $1 \leq p < \infty$ (cf. also Calderón couples), G. Weiss proved in [a12] that $\delta _ { A , B } ( X ) \in \mathcal{C} _ { 2 }$ implies $\delta _ { A^{*} , B ^ { * } } ( X ) \in \mathcal{C} _ { 2 }$. It has since been proved that the Putnam–Fuglede theorem holds modulo $\mathcal{C} _ { p }$ for all $1 < p < \infty$ [a1], [a12], and also with normal $A$, $B ^ { * }$ replaced by subnormal $A$, $B ^ { * }$. It is not known if the Putnam–Fuglede theorem holds modulo $\mathcal{C} _ { 1 }$.
Banach space formulation of the Putnam–Fuglede theorem.
Letting $A = a + i b$ and $B = c + i d$, where $a$, $b$, $c$, $d$ are self-adjoint operators such that $a b = b a$ and $c d = d c$ (cf. also Self-adjoint operator), the Putnam–Fuglede theorem can be written as
\begin{equation*} ( a + i b ) x = x ( c + i d ) \Leftrightarrow ( a - i b ) x = x ( c - i d ), \end{equation*}
or, equivalently,
\begin{equation*} ( a x - x c ) + i ( b x - x d ) = 0 \end{equation*}
\begin{equation*} \Updownarrow a x - x c = 0 \text { and } b x - x d = 0, \end{equation*}
for all $x \in B ( H )$. Defining $\mathcal{A}$ and $\mathcal{B}$ by $\mathcal{A} x = a x - x c$ and $\mathcal{B} x = b x - x d$, it is seen that $\mathcal{A}$ and $\mathcal{B}$ are Hermitian (i. e., the one-parameter groups $e ^ { i t {\cal A}}$ and $e ^ { i t \mathcal{B} }$, $t$ a real number, are groups of isometries on the Banach space $B ( H )$) which commute. The Putnam–Fuglede theorem now says that if $x \in B ( H )$ and $( \mathcal{A} + i \mathcal{B} ) x = 0$, then $\mathcal{A} x = 0 = \mathcal{B} x$. This version of the Putnam–Fuglede theorem has been generalized to the Banach space setting as follows: if $\mathcal{A}$ and $\mathcal{B}$ are commuting Hermitian operators on a complex Banach space $V$, then, given $x \in V$,
\begin{equation*} ( \mathcal{A} + i \mathcal{B} ) x = 0 \Leftrightarrow \mathcal{A} x = 0 = \mathcal{B} x \end{equation*}
(see [a3], [a4] for more general results).
References
| [a1] | A. Abdessemed, E.B. Davies, "Some commutator estimates in the Schatten classes" J. London Math. Soc. , 39 (1989) pp. 299–308 |
| [a2] | S.T.M. Ackermans, S.J.L. Eijndhoven, F.J.L. Martens, "On almost commuting operators" Nederl. Akad. Wetensch. Proc. Ser. A , 86 (1983) pp. 389–391 |
| [a3] | K. Boydazhiev, "Commuting $C_o $ groups and the Fuglede–Putnam theorem" Studia Math. , 81 (1985) pp. 303–306 |
| [a4] | M.J. Crabb, P.G. Spain, "Commutators and normal operators" Glasgow Math. J. , 18 (1977) pp. 197–198 |
| [a5] | B.P. Duggal, "On generalised Putnam–Fuglede theorems" Monatsh. Math. , 107 (1989) pp. 309–332 (See also: On quasi-similar hyponormal operators, Integral Eq. Oper. Th. 26 (1996), 338-345) |
| [a6] | T. Furuta, "On relaxation of normality in the Fuglede–Putnam theorem" Proc. Amer. Math. Soc. , 77 (1979) pp. 324–328 |
| [a7] | P.R. Halmos, "A Hilbert space problem book" , Springer (1982) |
| [a8] | B.E. Johnson, J.P. Williams, "The range of a normal derivation" Pacific J. Math. , 58 (1975) pp. 105–122 |
| [a9] | M. Radjabalipour, "An extension of Putnam–Fuglede theorem for hyponormal operators" Math. Z. , 194 (1987) pp. 117–120 |
| [a10] | H. Radjavi, P. Rosenthal, "On roots of normal operators" J. Math. Anal. Appl. , 34 (1971) pp. 653–664 |
| [a11] | V. Shulman, "Some remarks on the Fuglede–Weiss Theorem" Bull. London Math. Soc. , 28 (1996) pp. 385–392 |
| [a12] | G. Weiss, "The Fuglede commutativity theorem modulo the Hilbert–Schmidt class and generating functions I" Trans. Amer. Math. Soc. , 246 (1978) pp. 193–209 (See also: II, J. Operator Th. 5 (1981), 3-16) |
Putnam-Fuglede theorems. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Putnam-Fuglede_theorems&oldid=55339