Fourier integral operator
An integral operator with a generalized kernel that is a rapidly-oscillating function or the integral of such a function. Operators of this type arose when investigating the asymptotic expansions of rapidly-oscillating solutions to partial differential equations (see [1], [2]) and in studying the singularities of the fundamental solutions of hyperbolic equations (see [1], [2], [3]).
The Maslov canonical operator.
Let $ \Lambda $ be an $ n $- dimensional Lagrangian manifold of class $ C ^ \infty $ in the phase space $ \mathbf R _ {x, p } ^ {2n} $, where $ x \in \mathbf R ^ {n} $, and let $ d \sigma $ be the volume element on $ \Lambda $. A canonical atlas is a locally finite countable covering of $ \Lambda $ by bounded simply-connected domains $ \Omega _ {j} $( the charts) in each of which one can take as coordinates either the variables $ x $ or $ p $ or a mixed collection
$$ ( p _ \alpha , x _ \beta ),\ \ \alpha = ( \alpha _ {1} \dots \alpha _ {s} ),\ \ \beta = ( \beta _ {1} \dots \beta _ {n - s } ), $$
not containing dual pairs $ ( p _ {j} , x _ {j} ) $. The Maslov canonical operator sends $ C _ {0} ^ \infty ( \Lambda ) $ into $ C ( \mathbf R _ {x} ^ {n} ) $. The canonical operators $ K ( \Omega _ {j} ) $ are introduced as follows.
1) Let the chart $ \Omega _ {j} $ be non-degenerate, that is, $ \Omega _ {j} $ is given by an equation $ p = p ( x) $ and
$$ ( K ( \Omega _ {j} ) \phi ) ( x) = \ \sqrt {\left | \frac{d \sigma }{dx } \right | } \ \mathop{\rm exp} \left [ i \lambda \int\limits _ {r ^ {0} } ^ { r } ( p, dx) \right ] \phi ( r), $$
$$ r = ( x, p ( x)). $$
Here $ \lambda \geq 1 $ is a parameter, $ r ^ {0} \in \Omega _ {j} $ is a fixed point, $ ( p, dx) = \sum _ {j = 1 } ^ {n} p _ {j} dx _ {j} $, and $ \phi \in C _ {0} ^ \infty ( \Omega ) $.
2) Let the local coordinates in the chart $ \Omega _ {j} $ be $ p $, that is, $ \Omega _ {j} $ is given by an equation $ x = x ( p) $, and let
$$ ( K ( \Omega _ {j} ) \phi ) ( x) = \ F _ {\lambda , p \rightarrow x } ^ { - 1 } \left \{ \sqrt {\left | \frac{d \sigma }{dp } \right | }\right . \times $$
$$ \times \left . \mathop{\rm exp} \left [ i \lambda \left ( \int\limits _ {r ^ {0} } ^ { r } ( p, dx) - ( x ( p), p) \right ) \right ] \phi ( r) \right \} , $$
$$ r = ( x ( p), p). $$
Here $ F ^ { - 1 } $ is the Fourier $ \lambda $- transform
$$ F _ {\lambda , p \rightarrow x } ^ { - 1 } \psi ( x) = \ \left ( { \frac \lambda {- 2 \pi i } } \right ) ^ {n/2} \int\limits _ {\mathbf R ^ {n} } \mathop{\rm exp} [ i \lambda ( x, p)] \psi ( p) dp. $$
$ K ( \Omega _ {j} ) $ is defined analogously in the case when the coordinates in $ \Omega _ {j} $ are some collection $ ( p _ \alpha , x _ \beta ) $. Let $ \oint _ {l} ( p, dx) = 0 $ and let the Maslov index $ \mathop{\rm ind} l = 0 $ for any closed path $ l $ lying on $ \Lambda $. One introduces a partition of unity of class $ C ^ \infty $ on $ \Lambda $:
$$ \sum _ {j = 1 } ^ \infty e _ {j} ( x) = 1 \ \textrm{ and } \ \ \supp e _ {j} \subset \Omega _ {j} , $$
and one fixes a point $ r ^ {0} \in \Omega _ {j _ {0} } $. The Maslov canonical operator is defined by
$$ ( K _ \Lambda \phi ( r)) ( x) = \ \sum _ { j } c _ {j} K ( \Omega _ {j} ) ( e _ {j} \phi ) ( x), $$
$$ c _ {j} = \mathop{\rm exp} \left ( - { \frac{i \pi }{2} } \gamma _ {j} \right ) , $$
and $ \gamma _ {j} $ is the Maslov index of a chain of charts joining the charts $ \Omega _ {j _ {0} } $ and $ \Omega _ {j} $.
A point $ r \in \Lambda $ is called non-singular if it has a neighbourhood in $ \Lambda $ given by an equation $ p = p ( x) $. Let the intersection of the charts $ \Omega _ {i} $ and $ \Omega _ {j} $ be non-empty and connected, let $ r \in \Omega _ {i} \cap \Omega _ {j} $ be a non-singular point and let $ ( p _ \alpha , x _ \beta ) $, $ ( p _ {\widetilde \alpha } , x _ {\widetilde \beta } ) $ be the coordinates in these charts. The number
$$ \gamma _ {ij} = \ \sigma _ {-} \left ( \frac{\partial x _ \alpha ( r) }{\partial p _ \alpha } \right ) - \sigma _ {-} \left ( \frac{\partial x _ {\widetilde \alpha } ( r) }{\partial p _ {\widetilde \alpha } } \right ) $$
is the Maslov index of the pair of charts $ \Omega _ {j} $ and $ \Omega _ {j} $, where $ \sigma _ {-} ( A) $ is the number of negative eigen values of the matrix $ A $. The Maslov index of a chain of charts is defined by additivity. The Maslov index of a path $ l $ is defined analogously. The Maslov index of a path (mod 4) on a Lagrangian manifold is an integer homotopy invariant (see [1], [3]). The Maslov canonical operator is invariant under the choice of the canonical atlas, of local coordinates in the charts and the partition of unity in the following sense: If $ K _ \Lambda $, $ \widetilde{K} _ \Lambda $ are two Maslov canonical operators, then in $ L _ {2} ( \mathbf R ^ {n} ) $,
$$ ( K _ \Lambda \phi - \widetilde{K} _ \Lambda \phi ) ( x) = \ O ( \lambda ^ {-} 1 ),\ \ \lambda \rightarrow \infty , $$
for any function $ \phi \in C _ {0} ^ \infty ( \Lambda ) $.
The most important result in the theory of Maslov canonical operators is the commutation formula for the Maslov canonical operator and the $ \lambda $- differential (or $ \lambda $- pseudo-differential [3]) operator.
Let $ L ( x, \lambda ^ {-} 1 D) $ be a differential operator with real symbol $ L ( x, p) $ of class $ C ^ \infty $( cf. Symbol of an operator) and suppose that $ L ( x, p) = 0 $ on $ \Lambda $. Suppose that $ \Lambda $ and the volume element $ d \sigma $ are invariant under the Hamiltonian system
$$ \frac{dx }{d \tau } = \ \frac{\partial L }{\partial p } ,\ \ \frac{dp }{d \tau } = \ - \frac{\partial L }{\partial x } . $$
Then the following commutation formula is true (here $ \phi \in C _ {0} ^ \infty ( \Lambda ) $, $ \lambda \rightarrow \infty $):
$$ \tag{1 } L ( x, \lambda ^ {-} 1 D) ( K _ \Lambda \phi ) ( x) = \ { \frac{1}{i \lambda } } K _ \Lambda [ R \phi + O ( \lambda ^ {-} 1 )], $$
$$ R \phi = \left [ { \frac{d}{d \tau } } - { \frac{1}{2} } \sum _ {j = 1 } ^ { n } \frac{\partial ^ {2} L ( x, p) }{\partial x _ {j} \partial p _ {j} } \right ] \phi , $$
where $ d/d \tau $ is the derivative along the integral curves of the flow of the Hamiltonian system. For the other terms in the expansion (1) and an estimate for the remainder term see [3]. The equation $ R \phi = 0 $ is called the transport equation. The commutation formula implies that if $ R \phi = 0 $, then the function $ u = K _ \Lambda \phi $ is a formal asymptotic solution of the equation $ L ( x, \lambda ^ {-} 1 D) u = 0 $.
The method of the Maslov canonical operator enables one to solve the following problems.
1) The construction of an asymptotic solution to the Cauchy problem with rapidly-oscillating initial data in the large (that is, over any finite time interval) for strictly-hyperbolic systems of partial differential equations, for Dirac and Maxwell systems, for systems in the theory of elasticity, for the Schrödinger equation (see [1], [9]–[6] and also Quasi-classical approximation) and also the construction of solutions to certain mixed problems [4].
2) The construction of asymptotic expansions for the series of eigen values of self-adjoint differential operators associated with Lagrangian manifolds that are invariant under the corresponding Hamiltonian system (see [1], [3]).
3) The construction of asymptotic expansions up to smooth functions for the fundamental solution of a strictly-hyperbolic system of partial differential equations (see [1], [5], [6]).
4) The construction of shortwave asymptotics of the Green function, of the solution to the scattering problem and of the scattering amplitude for the Schrödinger equation, and of the asymptotics for the spectral function (see [5]–[7]).
A new version of the Maslov canonical operator has been developed on Lagrangian manifolds with complex fibres (see [8], [9]).
The Fourier integral operator.
Let $ X $, $ Y $ be bounded domains in $ \mathbf R _ {x} ^ {N _ {1} } $, $ \mathbf R _ {y} ^ {N _ {2} } $, $ N = N _ {1} + N _ {2} $, let $ \Gamma = X \times Y \times ( \mathbf R _ \theta ^ {N} \setminus \{ 0 \} ) $ and let $ u ( y) \in C _ {0} ^ \infty ( Y) $. The operator
$$ \tag{2 } ( Au) ( x) = \ { \frac{1}{2 \pi ^ {( n + 2N)/4 } } } \int\limits _ {\mathbf R _ \theta ^ {N} } \int\limits _ { Y } e ^ {i \phi ( x, y, \theta ) } p ( x, y, \theta ) u ( y) dy d \theta $$
is called a Fourier integral operator. Here $ \phi $( the phase function) is real and positively homogeneous of degree 1 in $ \theta $, $ \phi \in C ^ \infty ( \Gamma ) $, and $ \nabla _ {x, y, \theta } \phi \neq 0 $ when $ \theta \neq 0 $. The function $ p \in C ^ \infty ( \Gamma ) $( the symbol) has in the simplest case an asymptotic expansion, as $ | \theta | \rightarrow \infty $,
$$ p = \ \sum _ {j = 0 } ^ \infty p _ {j} \left ( x, y, { \frac \theta {| \theta | } } \right ) \ | \theta | ^ {m - j + ( n - 2N)/4 } . $$
The integral (2) converges after corresponding regularization and defines a continuous linear operator $ A: C _ {0} ^ \infty ( Y) \rightarrow D ^ \prime ( X) $. The kernel of $ A $ is
$$ K ( x, y) = \ \frac{1}{( 2 \pi ) ^ {( n + 2N)/4 } } \int\limits _ {\mathbf R _ \theta ^ {N} } e ^ {i \phi ( x, y, \theta ) } p ( x, y, \theta ) d \theta . $$
The function $ K ( x, y) \in D ^ \prime ( X \times Y) $ is infinitely differentiable outside the projection $ \pi C $ on $ X \times Y $ of the set $ C = \{ {( x, y, \theta ) \in \Gamma } : {\phi _ \theta = 0 } \} $. The singularities of $ K $ depend only on the Taylor expansion of the symbol $ p $ in a neighbourhood of $ C $( for a fixed phase $ \phi $). Let the phase $ \phi $ be non-degenerate, that is, let the differentials $ d _ {x, y, \theta } \phi _ {\theta _ {j} } ^ \prime $, $ 1 \leq j \leq N $, be linearly independent on $ C $; then $ C $ is a smooth manifold of dimension $ n $. To the operator $ A $ corresponds a smooth, conic (in the variables $ ( \zeta , \eta ) $ dual to $ z = ( x, y) $) Lagrangian manifold $ \Lambda \subset T ^ {*} ( X \times Y) \setminus \{ 0 \} $ of dimension $ n $— it is the image of $ C $ under the mapping
$$ \tag{3 } C \ni ( z, \theta ) \rightarrow \ ( z, \phi _ {z} ^ \prime ) \in \Lambda . $$
From now on, the operator $ A $ is considered on densities $ u ( y) $ of order $ 1/2 $:
$$ A : C _ {0} ^ \infty ( X, \Omega _ {1/2} ) \rightarrow \ D ^ \prime ( Y, \Omega _ {1/2} ), $$
that is, $ u ( y) \rightarrow u ( \widetilde{y} ) \sqrt {| d \widetilde{y} /dy | } $ under the change of variables $ y \rightarrow \psi ( \widetilde{y} ) $. To the symbol $ p $ corresponds the density $ b ( z, \tau ) $ of order $ 1/2 $ on $ \Lambda $ that is the image of $ p \sqrt {d _ {C} } $ under the mapping (3), where $ d _ {C} = | D ( \lambda , \phi _ \theta )/D ( x, \theta ) | ^ {-} 1 $ and $ \lambda = ( \lambda _ {1} \dots \lambda _ {n} ) $ are the coordinates on $ \Lambda $, homogeneous of degree 1 in $ \tau $, carried over to $ C $ by means of (3). As $ | \tau | \rightarrow \infty $, the density $ b $ has an asymptotic expansion
$$ b ( z, \tau ) = \ \sum _ {j = 0 } ^ \infty b _ {j} \left ( z, { \frac \tau {| \tau | } } \right ) ^ {m - j - n/4 } , $$
the coefficient $ b _ {0} $ is called the principal symbol of the operator $ A $.
Let the operator $ A $ be represented in the form (2) but with another non-degenerate phase function $ \widetilde \phi ( x, y, \widetilde \theta ) $, $ \widetilde \theta \in \mathbf R ^ {N} $, and with another symbol $ \widetilde{p} ( x, y, \widetilde \theta ) $. Then for this representation the manifold $ \Lambda $ remains the same, the quantity $ \sigma = \mathop{\rm sign} \phi _ {\theta \theta } - \mathop{\rm sign} \widetilde \phi _ {\widetilde \theta \widetilde \theta } $ is constant and the principal symbol $ \widetilde{b} _ {0} $ is
$$ \widetilde{b} _ {0} = \ e ^ {( i \pi \sigma )/ 4 } b _ {0} . $$
The general definition of a Fourier integral operator is as follows. Let $ X $, $ Y $ be smooth manifolds of dimensions $ N _ {1} $, $ N _ {2} $ and let $ \Lambda \subset T ^ {*} ( X \times Y) \setminus \{ 0 \} $ be a conic smooth Lagrangian manifold of dimension $ n = N _ {1} + N _ {2} $. For any point $ \lambda \in \Lambda $ there is a non-degenerate phase function such that the Lagrangian manifold constructed with respect to it coincides locally with $ \Lambda $. Let $ \{ x _ {j} ^ \prime , y _ {j} ^ \prime , \phi _ {j} , N ^ {j} , \Gamma _ {j} , u _ {j} \} $ be the set of objects consisting of:
a) local coordinate neighbourhoods $ X ^ \prime \subset X $, $ Y ^ \prime \subset Y $ with local coordinates $ x \in \mathbf R ^ {N _ {1} } $, $ y \in \mathbf R ^ {N _ {2} } $, $ z = ( x, y) $;
b) an integer $ N $ and a non-degenerate phase function $ \phi $ defined on $ \Gamma = X ^ \prime \times Y ^ \prime \times ( \mathbf R ^ {N} \setminus \{ 0 \} ) $ such that the mapping
$$ \{ {( z, \theta ) \in \Gamma } : { \phi _ \theta ( z, \theta ) = 0 } \} \ \ni ( z, \theta ) \rightarrow ( z, \phi _ {z} ) $$
is a diffeomorphism onto an open subset $ U \subset \Lambda $. The operator
$$ A = \sum A _ {j} $$
is called a Fourier integral operator, where $ A _ {j} $ has the form (2), $ N = N ^ {j} $, $ \phi = \phi _ {j} - \pi N ^ {j} /4 $ and the support of the symbol $ p = p _ {j} $ lies in $ K _ {j} \times \mathbf R ^ {N ^ {j} } $, where $ K _ {j} $ is a compact set in $ X _ {j} ^ \prime \times Y _ {j} ^ \prime $. The class of such operators $ A $ is denoted by $ I ^ {m} ( \Lambda ) $.
Let $ \widetilde{S} {} ^ {m - n/4 } ( \Lambda , \Omega _ {1/2} ) $ be the set of homogeneous densities of order $ 1/2 $ that are of degree $ m - n/4 $ with respect to $ \tau $ on $ \Lambda $. From the principal symbols $ b _ {0} ^ {j} ( z, \tau ) $ of the operators $ A _ {j} $ one can construct in a natural way the principal symbol $ b _ {0} ( z, \tau ) \in \widetilde{S} {} ^ {m - n/4 } ( \Lambda , \Omega _ {1/2} \otimes L) $ of $ A $ such that the mapping
$$ I ^ {m} ( \Lambda )/I ^ {m - 1 } ( \Lambda ) \rightarrow \ \widetilde{S} {} ^ {m - n/4 } ( \Lambda , \Omega _ {1/2} \otimes L) $$
is an isomorphism (see [2], [14]).
The most important case for applications of Fourier integral operators to partial differential equations is when the projections $ \Lambda \rightarrow T ^ {*} ( Y) $ are local diffeomorphisms. Then $ N _ {1} = N _ {2} $, the density $ d _ {C} $ is equal to
$$ d _ {C} ^ {-} 1 = \ \mathop{\rm det} \left \| \begin{array}{cc} \phi _ {\theta \theta } &\phi _ {\theta x } \\ \phi _ {y \theta } &\phi _ {yx} \\ \end{array} \right \| , $$
and the operator
$$ I ^ {0} ( \Lambda ) \ni \ A : L _ { \mathop{\rm loc} } ^ {2} ( Y, \Omega _ {1/2} ) \rightarrow \ L _ { \mathop{\rm loc} } ^ {2} ( X, \Omega _ {1/2} ) $$
is bounded.
Just as for the Maslov canonical operator there are commutation formulas for Fourier integral operators with differential operators, as well as all implications following from these. Locally a Fourier integral operator can be represented as an integral with respect to a parameter over the Maslov canonical operator (see [10]). The Fourier integral operator is applied:
1) to construct parametrices and to study the micro-local structure of the singularities (wave front sets) of solutions to hyperbolic equations, equations of principal type and boundary value problems (see [2], [14]);
2) to investigate the question of the local and global solvability and subellipticity of equations (see [12]); and
3) to obtain asymptotic expansions for the spectral functions of pseudo-differential operators (see [13]).
References
| [1] | V.P. Maslov, "Théorie des perturbations et méthodes asymptotiques" , Dunod (1972) (Translated from Russian) Zbl 0247.47010 |
| [2] | L. Hörmander, "Fourier integral operators, I" Acta Math. , 127 (1971) pp. 79–183 MR0388463 Zbl 0212.46601 |
| [3] | V.P. Maslov, M.V. Fedoryuk, "Quasi-classical approximation for the equations of quantum mechanics" , Reidel (1981) (Translated from Russian) |
| [4] | M.V. Fedoryuk, "Singularities of the kernels of Fourier integral operators and the asymptotic behaviour of the solution of the mixed problem" Russian Math. Surveys , 32 : 6 (1977) pp. 67–120 Uspekhi Mat. Nauk , 32 : 6 (1977) pp. 67–115 |
| [5] | V.V. Kucherenko, "Semi-classical asymptotics of a point source function for a steady-state Schrödinger equation" Teoret. i Mat. Fiz. , 1 (1969) pp. 384–406 (In Russian) |
| [6] | B.R. Vainberg, "Asymptotic methods in the equations of mathematical physics" , Gordon & Breach (1988) (Translated from Russian) Zbl 0743.35001 |
| [7] | B.R. Vainberg, "A complete asymptotic expansion of the spectral function of second order elliptic operators in 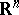 " Math. USSR-Sb. , 51 : 1 (1985) pp. 191–206 Mat. Sb. , 123 : 2 (1984) pp. 195–211 " Math. USSR-Sb. , 51 : 1 (1985) pp. 191–206 Mat. Sb. , 123 : 2 (1984) pp. 195–211 |
| [8] | V.V. Kucherenko, "Asymptotic solution of the Cauchy problem for equations with complex characteristics" J. Soviet Math. , 13 : 1 (1980) pp. 24–118 Itogi Nauk. i Tekhn. Sovr. Probl. Mat. , 8 (1977) pp. 41–136 Zbl 0457.35096 |
| [9] | V.P. Maslov, "Operational methods" , MIR (1976) (Translated from Russian) MR0512495 Zbl 0449.47002 |
| [10] | A.S. Mishchenko, B.Yu. Sternin, V.E. Shatalov, "Lagrangian manifolds and the method of the canonical operator" , Moscow (1978) (In Russian) MR0511792 |
| [11] | J. Leray, "Lagrangian analysis and quantum mechanics" , M.I.T. (1981) (Translated from French) MR0646266 MR0644633 Zbl 0483.35002 |
| [12] | Yu.B. Egorov, "Subelliptic operators" Russian Math. Surveys , 30 : 2 (1975) pp. 59–118 Uspekhi Mat. Nauk , 30 : 2 (1975) pp. 57–114 MR0410474 MR0410473 Zbl 0331.35054 Zbl 0318.35075 |
| [13] | M.A. Shubin, "Pseudo differential operators and spectral theory" , Springer (1987) (Translated from Russian) MR883081 |
| [14] | F. Trèves, "Introduction to pseudodifferential and Fourier integral operators" , 1–2 , Plenum (1980) MR0597145 MR0597144 Zbl 0453.47027 |
Comments
The approach through asymptotic expansions of rapidly-oscillating solutions to partial differential equations is given in [a5], [a6], while [a4] approaches Fourier integral operators from the study of fundamental solutions of hyperbolic equations.
Concerning singularities of the Lagrangian manifold $ \Lambda $ see [a5]. The fact that the Maslov index (mod 4) is a homotopy invariant can also be found in [a3]. Concerning (higher-order terms in) (1) see [a4], [a6]. For the use of the Maslov index see [a2], [a6].
For Fourier integral operators in the construction of parametrices, the structure of singularities and the solvability and subellipticity problems for equations see [a4].
The connection with asymptotic expansions can be found in [a7], [a8].
Fourier integral operators with complex phase functions were developed in [a9].
[a10] -[a14] are some (recent) textbooks.
References
| [a1] | L.V. Hörmander, "The analysis of linear partial differential operators" , 4. Fourier integral operators , Springer (1985) MR1540773 MR0781537 MR0781536 Zbl 0612.35001 Zbl 0601.35001 |
| [a2] | P.D. Lax, "Asymptotic solutions of oscillatory initial value problems" Duke Math. J. , 24 (1957) pp. 627–646 MR0097628 Zbl 0083.31801 |
| [a3] | V.I. Arnol'd, "Characteristic class entering in quantization conditions" Funct. Anal. Appl. , 1 (1967) pp. 1–13 Funkts. Anal. i Prilozhen. , 1 (1967) pp. 1–14 Zbl 0175.20303 |
| [a4] | J.J. Duistermaat, L. Hörmander, "Fourier integral operators II" Acta Math. , 128 (1972) pp. 183–269 MR0388464 Zbl 0232.47055 |
| [a5] | V.I. Arnol'd, "Integrals of rapidly oscillating functions and singularities of projections of Lagrangian manifolds" Funct. Anal. Appl. , 6 (1972) pp. 222–224 Funkts. Anal. i Prilozhen. , 6 (1972) pp. 61–62 Zbl 0278.57010 |
| [a6] | J.J. Duistermaat, "Oscillatory integrals, Lagrange immersions and unfoldings of singularities" Comm. Pure Appl. Math. , 27 (1974) pp. 207–281 MR0405513 Zbl 0285.35010 Zbl 0276.35010 |
| [a7] | J. Chazarain, "Formules de Poisson pour les variétés riemanniennes" Invent. Math. , 24 (1974) pp. 65–82 MR0343320 |
| [a8] | J.J. Duistermaat, V.W. Guillemin, "The spectrum of positive elliptic operators and periodic bicharacteristics" Invent. Math. , 29 (1975) pp. 39–79 MR0405514 Zbl 0344.35067 Zbl 0307.35071 |
| [a9] | A. Melin, J. Sjöstrand, "Fourier integral operators with complex phase functions and parametrix for an interior boundary value problem" Comm. Part. Diff. Equations , 1 (1976) pp. 313–400 MR0455054 Zbl 0364.35049 |
| [a10] | M.E. Taylor, "Pseudo-differential operators" , Princeton Univ. Press (1981) MR1567325 Zbl 0289.35001 Zbl 0207.45402 |
| [a11] | B.E. Petersen, "Introduction to the Fourier transform and pseudo-differential operators" , Pitman (1983) Zbl 0523.35001 |
| [a12] | J. Chazarain, A. Piriou, "Introduction to the theory of partial differential equations" , North-Holland (1982) (Translated from French) MR678605 Zbl 0487.35002 |
| [a13] | J.J. Duistermaat, "Fourier integral operators" , Courant Inst. Math. (1973) MR0451313 Zbl 0272.47028 |
| [a14] | J. Dieudonné, "Eléments d'analyse" , 7–8 , Gauthier-Villars (1978) MR0494182 MR0494181 |
Fourier integral operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fourier_integral_operator&oldid=46964