User:Boris Tsirelson/sandbox2
the method is as follows: \begin{equation} \begin{gathered} k_1 = h f(x_0,y_0), \quad y_0 = y(x_0),\\ k_2 = h f \big( x_0 + \tfrac13 h, y_0 + \tfrac13 k_1 \big) \, , \\ k_3 = h f \big( x_0 + \tfrac13 h, y_0 + \tfrac16 k_1 + \tfrac16 k_2 \big) \, , \\ k_4 = h f \big( x_0 + \tfrac12 h, y_0 + \tfrac18 k_1 + \tfrac38 k_3 \big) \, , \\ \end{gathered} \end{equation}
The number
the method is as follows:
 | (2) |
 |
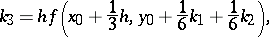 |
 |
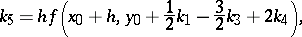 |
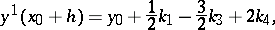 |
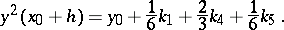 |
The number
$$\text{ $K$ compact}$$
\[\text{ '"`UNIQ-MathJax3-QINU`"' compact}\]
\begin{equation} \mu (B)= \sup \{\mu(K): K\subset B, \text{ '"`UNIQ-MathJax4-QINU`"' compact}\}\, \end{equation}
and having the following property: \begin{equation}\label{e:tight} \mu (B)= \sup \{\mu(K): K\subset B, \mbox{ '"`UNIQ-MathJax5-QINU`"' compact}\}\, \end{equation} (see [Sc]).
The total variation measure of a $\mathbb C$-valued measure is defined on $\mathcal{B}$ as:
\[
\abs{\mu}(B) :=\sup\left\{ \sum \abs{\mu(B_i)}: \text{$\{B_i\}\subset\mathcal{B}'"`UNIQ-MathJax8-QINU`"'B$}\right\}.
\]
In the real-valued case the above definition simplifies as
and the following identity holds: \begin{equation}\label{e:area_formula} \int_A J f (y) \, dy = \int_{\mathbb R^m} \mathcal{H}^0 (A\cap f^{-1} (\{z\}))\, d\mathcal{H}^n (z)\, . \end{equation}
Cp. with 3.2.2 of [EG]. From \eqref{e:area_formula} it is not difficult to conclude the following generalization (which also goes often under the same name):
\begin{equation}\label{ab}
E=mc^2
\end{equation}
By \eqref{ab}, it is possible. But see \eqref{ba} below:
\begin{equation}\label{ba}
E\ne mc^3,
\end{equation}
which is a pity.
Boris Tsirelson/sandbox2. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Boris_Tsirelson/sandbox2&oldid=32517