Tower of fields

field tower

An extension sequence

(*)

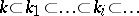of some field 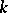. Depending on the properties of the extensions , the tower is called normal, Abelian, separable, etc. The concept of a field tower plays an important role in Galois theory, in which the problem of expressing the roots of equations by radicals is reduced to the possibility of including the splitting field of the equation into a normal Abelian field tower.

In class field theory the tower

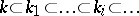occurs, where 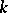is some algebraic number field, while each field is the Hilbert class field of (i.e. the maximal Abelian unramified extension of 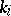). The Galois group of any extension 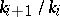is isomorphic to the ideal class group of the field 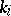(by Artin's reciprocity law) and, since the latter group is finite, all extensions 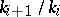are finite as well. The union 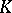of the fields is the maximal solvable unramified extension of 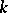. The question of the finiteness of the extension 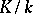(the class field tower problem) was posed in 1925 by Ph. Furtwängler and was negatively answered in 1964 [2]. An example of a field with an infinite class field tower is the extension of the field of rational numbers obtained by adjoining 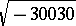. It is impossible to imbed such a field in an algebraic number field that has unique factorization. The solution of the problem has applications in algebraic number theory, e.g. in obtaining a precise estimate of the growth of discriminants of algebraic number fields.

References

[1]	J.W.S. Cassels (ed.) A. Fröhlich (ed.) , Algebraic number theory , Acad. Press (1967)
[2]	E.S. Golod, I.R. Shafarevich, "On class field towers" Transl. Amer. Math. Soc. (2) , 48 (1965) pp. 91–102 Izv. Akad. Nauk SSSR Ser. Mat. , 28 (1964) pp. 261–272