Tomita-Takesaki theory
M. Tomita [a4] defined the notion of a left Hilbert algebra as follows: An involutive algebra  over the field
over the field  of complex numbers, with involution
of complex numbers, with involution 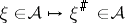 , that admits an inner product
, that admits an inner product 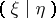 satisfying the following conditions:
satisfying the following conditions:
i) the mapping 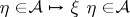 is continuous for every
is continuous for every 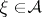 ;
;
ii) 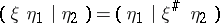 for all
for all 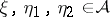 ;
;
iii)  is total in the Hilbert space
is total in the Hilbert space 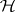 obtained by completion of
obtained by completion of 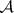 .
.
iv) 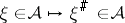 is a closeable conjugate-linear operator in
is a closeable conjugate-linear operator in 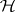 . Let
. Let 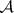 be a left Hilbert algebra in a Hilbert space
be a left Hilbert algebra in a Hilbert space 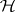 . For any
. For any 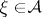 , let
, let 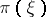 denote the unique continuous linear operator on
denote the unique continuous linear operator on 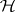 such that
such that 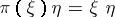 ,
, 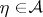 . The von Neumann algebra
. The von Neumann algebra 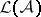 generated by
generated by 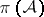 is called the left von Neumann algebra of
is called the left von Neumann algebra of 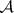 . Let
. Let  be the closure of the mapping
be the closure of the mapping 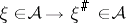 and let
and let 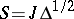 be the polar decomposition of
be the polar decomposition of 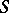 . Then
. Then 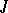 is an isometric involution and
is an isometric involution and  is a non-singular positive self-adjoint operator in
is a non-singular positive self-adjoint operator in 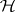 satisfying
satisfying 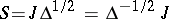 and
and 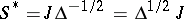 ;
; 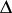 and
and 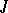 are called the modular operator and the modular conjugation operator of
are called the modular operator and the modular conjugation operator of 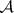 , respectively. Let
, respectively. Let 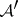 denote the set of vectors
denote the set of vectors 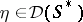 such that the mapping
such that the mapping 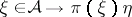 is continuous. For any
is continuous. For any 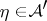 , denote by
, denote by 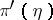 the unique continuous extension of
the unique continuous extension of 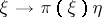 to
to  . Let
. Let 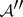 be the set of vectors
be the set of vectors 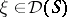 such that the mapping
such that the mapping 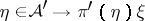 is continuous. For any
is continuous. For any  , denote by
, denote by  the unique continuous extension of
the unique continuous extension of  to
to 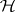 . Then
. Then 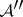 is a left Hilbert algebra in
is a left Hilbert algebra in 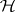 , equipped with the multiplication
, equipped with the multiplication 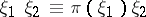 and the involution
and the involution 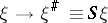 , and
, and 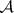 is equivalently contained in
is equivalently contained in 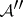 , that is,
, that is, 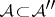 and they have the same modular (conjugation) operators. The set
and they have the same modular (conjugation) operators. The set 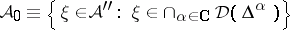 is a left Hilbert algebra which is equivalently contained in
is a left Hilbert algebra which is equivalently contained in 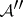 and
and 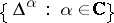 is a complex one-parameter group of automorphisms of
is a complex one-parameter group of automorphisms of 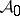 , called the modular automorphism group. It satisfies the conditions:
, called the modular automorphism group. It satisfies the conditions:
a) 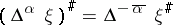 ,
, 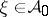 ,
,  ;
;
b)  ,
, 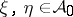 ,
, 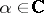 ;
;
c) 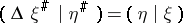 ,
,  ;
;
d) 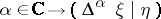 ,
, 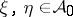 , is an analytic function on
, is an analytic function on  . Such a left Hilbert algebra is called a modular Hilbert algebra (or Tomita algebra). Using the theory of modular Hilbert algebras, M. Tomita proved that
. Such a left Hilbert algebra is called a modular Hilbert algebra (or Tomita algebra). Using the theory of modular Hilbert algebras, M. Tomita proved that 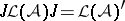 and
and  for all
for all 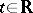 . This theorem is called the Tomita fundamental theorem. M. Takesaki [a3] arranged and deepened this theory and connected this theory with the Haag–Hugenholtz–Winnink theory [a2] for equilibrium states for quantum statistical mechanics. After that, Tomita–Takesaki theory was developed by A. Connes [a1], H. Araki, U. Haagerup and the others, and has contributed to the advancement of the structure theory of von Neumann algebras, non-commutative integration theory, and quantum physics. Using an integral formula relating the resolvent of the modular operator
. This theorem is called the Tomita fundamental theorem. M. Takesaki [a3] arranged and deepened this theory and connected this theory with the Haag–Hugenholtz–Winnink theory [a2] for equilibrium states for quantum statistical mechanics. After that, Tomita–Takesaki theory was developed by A. Connes [a1], H. Araki, U. Haagerup and the others, and has contributed to the advancement of the structure theory of von Neumann algebras, non-commutative integration theory, and quantum physics. Using an integral formula relating the resolvent of the modular operator 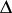 with the operators
with the operators 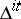 , A. van Daele [a5] has simplified a discussion in the complicated Tomita–Takesaki theory.
, A. van Daele [a5] has simplified a discussion in the complicated Tomita–Takesaki theory.
References
| [a1] | A. Connes, "Une classification des facteurs de type III" Ann. Sci. Ecole Norm. Sup. , 6 (1973) pp. 133–252 |
| [a2] | R. Haag, N.M. Hugenholts, M. Winnink, "On the equilibrium states in quantum mechanics" Comm. Math. Phys. , 5 (1967) pp. 215–236 |
| [a3] | M. Takesaki, "Tomita's theory of modular Hilbert algebras and its applications" , Lecture Notes Math. , 128 , Springer (1970) |
| [a4] | M. Tomita, "Standard forms of von Neumann algebras" , The Vth Functional Analysis Symposium of Math. Soc. Japan, Sendai (1967) |
| [a5] | A. Van Daele, "A new approach to the Tomita–Takesaki theory of generalized Hilbert algebras" J. Funct. Anal. , 15 (1974) pp. 378–393 |
Tomita-Takesaki theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tomita-Takesaki_theory&oldid=18663