Representation of the symmetric groups
A linear representation of the group 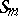 over a field
over a field 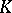 . If
. If 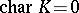 , then all finite-dimensional representations of the symmetric groups are completely reducible (cf. Reducible representation) and defined over
, then all finite-dimensional representations of the symmetric groups are completely reducible (cf. Reducible representation) and defined over 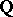 (in other words, irreducible finite-dimensional representations over
(in other words, irreducible finite-dimensional representations over  are absolutely irreducible).
are absolutely irreducible).
The irreducible finite-dimensional representations of 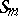 over
over 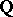 are classified as follows. Let
are classified as follows. Let 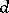 be a Young diagram corresponding to a partition
be a Young diagram corresponding to a partition 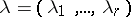 of the number
of the number  , let
, let 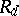 (respectively,
(respectively, 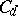 ) be the subgroup of
) be the subgroup of 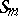 consisting of all permutations mapping each of the numbers
consisting of all permutations mapping each of the numbers  into a number in the same row (respectively, column) of
into a number in the same row (respectively, column) of 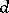 . Then
. Then
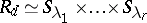 |
and
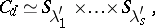 |
where 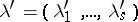 is the partition of
is the partition of 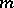 dual to
dual to  . There exists a unique irreducible representation
. There exists a unique irreducible representation 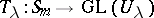 of
of 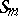 (depending on
(depending on 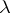 only) with the following properties: 1) in the space
only) with the following properties: 1) in the space 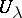 there is a non-zero vector
there is a non-zero vector 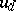 such that
such that 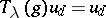 for any
for any 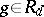 ; and 2) in
; and 2) in 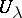 there exists a non-zero vector
there exists a non-zero vector 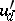 such that
such that 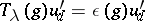 for any
for any 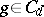 , where
, where 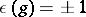 is the parity (sign) of
is the parity (sign) of  . Representations corresponding to distinct partitions are not equivalent, and they exhaust all irreducible representations of
. Representations corresponding to distinct partitions are not equivalent, and they exhaust all irreducible representations of 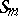 over
over 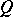 .
.
The vectors  and
and 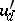 are defined uniquely up to multiplication by a scalar. For all diagrams corresponding to a partition
are defined uniquely up to multiplication by a scalar. For all diagrams corresponding to a partition  these vectors are normalized such that
these vectors are normalized such that 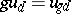 and
and 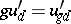 for any
for any 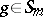 . Here
. Here 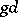 denotes the diagram obtained from
denotes the diagram obtained from 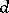 by applying to all numbers the permutation
by applying to all numbers the permutation 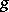 . The vectors
. The vectors  (respectively,
(respectively,  ) corresponding to standard diagrams
) corresponding to standard diagrams 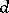 form a basis for
form a basis for 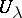 . In this basis the operators of the representation
. In this basis the operators of the representation 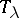 have the form of integral matrices. The dimension of
have the form of integral matrices. The dimension of  is
is
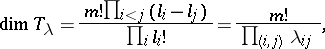 |
where  ,
, 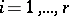 , and the product in the denominator of the last expression is taken over all cells
, and the product in the denominator of the last expression is taken over all cells 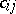 of the Young tableau
of the Young tableau 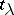 ;
; 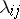 denotes the length of the corresponding hook.
denotes the length of the corresponding hook.
To the partition 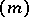 corresponds the trivial one-dimensional representation of
corresponds the trivial one-dimensional representation of 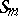 , while to the partition
, while to the partition  corresponds the non-trivial one-dimensional representation
corresponds the non-trivial one-dimensional representation  (the parity or sign representation). To the partition
(the parity or sign representation). To the partition 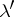 dual to
dual to 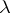 corresponds the representation
corresponds the representation 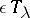 . The space
. The space  can be identified (in a canonical way, up to a homothety) with
can be identified (in a canonical way, up to a homothety) with 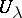 , so that
, so that 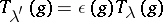 for any
for any 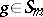 . Moreover, one may take
. Moreover, one may take 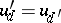 , where
, where 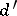 is the diagram obtained from
is the diagram obtained from 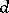 by transposition.
by transposition.
The construction of a complete system of irreducible representations of a symmetric group invokes the use of the Young symmetrizer, and allows one to obtain the decomposition of the regular representation. If 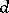 is the Young diagram corresponding to a partition
is the Young diagram corresponding to a partition 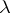 , then the representation
, then the representation  is equivalent to the representation of
is equivalent to the representation of 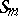 in the left ideal of the group algebra
in the left ideal of the group algebra 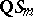 generated by the Young symmetrizer
generated by the Young symmetrizer 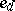 . An a posteriori description of
. An a posteriori description of  is the following:
is the following: 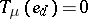 for
for 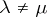 , and
, and 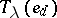 is the operator, of rank 1, acting by the formula
is the operator, of rank 1, acting by the formula 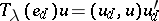 for any
for any 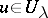 . Here
. Here 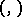 denotes the invariant scalar product in
denotes the invariant scalar product in 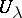 , normalized in a suitable manner. Moreover,
, normalized in a suitable manner. Moreover,
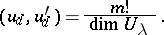 |
The Frobenius formula gives a generating function for the characters of 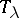 . However, recurrence relations are more convenient to use when computing individual values of characters. The Murnagan–Nakayama rule is most effective: Let
. However, recurrence relations are more convenient to use when computing individual values of characters. The Murnagan–Nakayama rule is most effective: Let  be the value of a character of
be the value of a character of 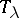 on the class
on the class 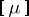 of conjugate elements of
of conjugate elements of 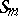 defined by a partition
defined by a partition 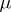 of
of 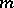 , and suppose that
, and suppose that 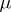 contains a number
contains a number 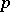 . Denote by
. Denote by  the partition of
the partition of 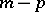 obtained from
obtained from  by deleting
by deleting 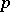 . Then
. Then
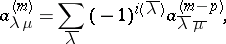 |
where the sum is over all partitions  of
of 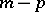 obtained by deleting a skew hook of length
obtained by deleting a skew hook of length 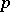 from the Young tableau
from the Young tableau 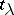 , and where
, and where 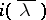 denotes the height of the skew hook taken out.
denotes the height of the skew hook taken out.
There is also a method (cf. [5]) by which one can find the entire table of characters of 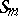 , i.e. the matrix
, i.e. the matrix 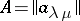 . Let
. Let 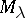 be the representation of
be the representation of  induced by the trivial one-dimensional representation of the subgroup
induced by the trivial one-dimensional representation of the subgroup 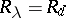 , where
, where 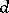 is the Young diagram corresponding to the partition
is the Young diagram corresponding to the partition 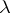 . Let
. Let 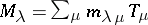 and
and 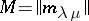 . If one assumes that the rows and columns of
. If one assumes that the rows and columns of 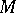 are positioned in order of lexicographically decreasing indices (partitions), then
are positioned in order of lexicographically decreasing indices (partitions), then 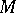 is a lower-triangular matrix with 1's on the diagonal. The value of a character of
is a lower-triangular matrix with 1's on the diagonal. The value of a character of 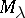 on a class
on a class 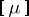 is equal to
is equal to
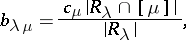 |
where 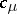 is the order of the centralizer of the permutations (a representative) from
is the order of the centralizer of the permutations (a representative) from 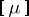 . The matrix
. The matrix 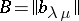 is upper triangular, and one has
is upper triangular, and one has 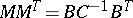 , where
, where 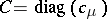 , from which
, from which  can be uniquely found. Then the matrix
can be uniquely found. Then the matrix 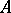 is determined by
is determined by
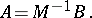 |
The restriction of a representation 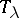 of
of 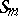 to the subgroup
to the subgroup 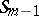 can be found by the ramification rule
can be found by the ramification rule
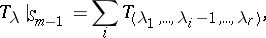 |
where the summation extends over all 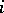 for which
for which 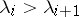 (including
(including  ). The restriction of
). The restriction of 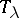 to the subgroup
to the subgroup 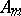 is absolutely irreducible for
is absolutely irreducible for  and splits for
and splits for  over a quadratic extension of
over a quadratic extension of 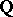 into a sum of two non-equivalent absolutely-irreducible representations of equal dimension. The representations of
into a sum of two non-equivalent absolutely-irreducible representations of equal dimension. The representations of 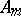 thus obtained exhaust all its irreducible representations over
thus obtained exhaust all its irreducible representations over  .
.
For representations of the symmetric groups in tensors see Representation of the classical groups.
The theory of modular representations of the symmetric groups has also been developed (see, e.g. [5]).
References
| [1] | H. Weyl, "The classical groups, their invariants and representations" , Princeton Univ. Press (1946) |
| [2] | F.D. Murnagan, "The theory of group representations" , Johns Hopkins Univ. Press (1938) |
| [3] | M. Hamermesh, "Group theory and its application to physical problems" , Addison-Wesley (1962) |
| [4] | C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962) |
| [5] | G.D. James, "The representation theory of the symmetric groups" , Springer (1978) |
Comments
Let 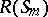 be the free Abelian group generated by the complex irreducible representations of the symmetric group on
be the free Abelian group generated by the complex irreducible representations of the symmetric group on 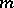 letters,
letters, 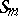 . Now consider the direct sum
. Now consider the direct sum
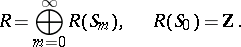 |
It is possible to define a Hopf algebra structure on 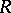 , as follows. First the multiplication. Let
, as follows. First the multiplication. Let  and
and  be, respectively, representations of
be, respectively, representations of 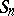 and
and 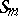 . Taking the tensor product defines a representation
. Taking the tensor product defines a representation 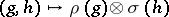 of
of 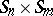 . Consider
. Consider 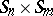 as a subgroup of
as a subgroup of 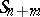 in the natural way. The product of
in the natural way. The product of 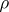 and
and  in
in 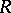 is now defined by taking the induced representation to
is now defined by taking the induced representation to 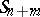 :
:
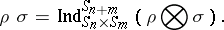 |
For the comultiplication restriction is used. Let 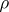 be a representation of
be a representation of 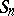 . For every
. For every 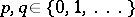 ,
,  , consider the restriction of
, consider the restriction of 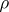 to
to 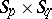 to obtain an element of
to obtain an element of 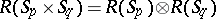 . The comultiplication of
. The comultiplication of  is now defined by
is now defined by
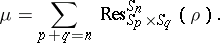 |
There is a unit mapping 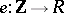 , defined by identifying
, defined by identifying 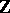 and
and  , and an augmentation
, and an augmentation 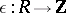 , defined by
, defined by 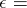 identity on
identity on 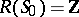 and
and 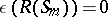 if
if 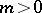 . It is a theorem that
. It is a theorem that 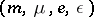 define a graded bi-algebra structure on
define a graded bi-algebra structure on 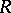 . There is also an antipode, making
. There is also an antipode, making 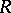 a graded Hopf algebra.
a graded Hopf algebra.
This Hopf algebra can be explicitly described as follows. Consider the commutative ring of polynomials in infinitely many variables 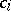 ,
, 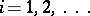 ,
,  ,
,
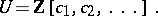 |
A co-algebra structure is given by
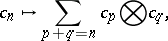 |
and a co-unit by 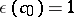 ,
, 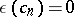 for
for 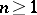 . There is also an antipode, making
. There is also an antipode, making  also a graded Hopf algebra. Perhaps the basic result in the representation theory of the symmetric groups is that
also a graded Hopf algebra. Perhaps the basic result in the representation theory of the symmetric groups is that 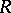 and
and 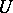 are isomorphic as Hopf algebras. The isomorphism is very nearly unique because, [a1],
are isomorphic as Hopf algebras. The isomorphism is very nearly unique because, [a1],
 |
The individual components 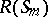 of
of 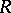 are also rings in themselves under the product of representations
are also rings in themselves under the product of representations 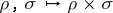 ,
,  . This defines a second multiplication on
. This defines a second multiplication on 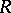 , which is distributive over the first, and
, which is distributive over the first, and 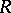 becomes a ring object in the category of co-algebras over
becomes a ring object in the category of co-algebras over 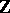 . Such objects have been called Hopf algebras, [a6], and quite a few of them occur naturally in algebraic topology. The ring
. Such objects have been called Hopf algebras, [a6], and quite a few of them occur naturally in algebraic topology. The ring 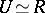 occurs in algebraic topology as
occurs in algebraic topology as 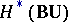 , the cohomology of the classifying space
, the cohomology of the classifying space 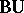 of complex
of complex 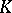 -theory, and there is a "natural direct isomorphism"
-theory, and there is a "natural direct isomorphism" 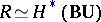 , [a3]. (This explains the notation used above for
, [a3]. (This explains the notation used above for 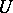 : the "ci" stand for Chern classes, cf. Chern class.)
: the "ci" stand for Chern classes, cf. Chern class.)
There is also an inner product on 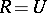 :
:  counts the number of irreducible representations that
counts the number of irreducible representations that 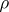 and
and  have in common, and with respect to this inner product
have in common, and with respect to this inner product 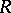 is (graded) self-dual. In particular, the multiplication and comultiplication are adjoint to one another:
is (graded) self-dual. In particular, the multiplication and comultiplication are adjoint to one another:
 |
which is the same as Frobenius reciprocity, cf. Induced representation, in this case.
As a coring object in the category of algebras 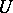 , being the representing object
, being the representing object 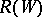 of the functor of Witt vectors, [a2], plays an important role in formal group theory. But, so far, no direct natural isomorphism has been found linking
of the functor of Witt vectors, [a2], plays an important role in formal group theory. But, so far, no direct natural isomorphism has been found linking 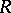 with
with  in this manifestation.
in this manifestation.
The ring 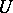 also carries the structure of a
also carries the structure of a 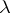 -ring and it is in fact the universal
-ring and it is in fact the universal 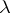 -ring on one generator,
-ring on one generator, 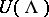 , [a4], and this gives a natural isomorphism
, [a4], and this gives a natural isomorphism  , cf.
, cf.  -ring for some more details.
-ring for some more details.
Finally there is a canonical notion of positivity on 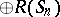 : the actual (i.e. not virtual) representations are positive and the multiplication and comultiplication preserve positivity. This has led to the notion of a PSH-algebra, which stands for positive self-adjoint Hopf algebra, [a5]. Essentially,
: the actual (i.e. not virtual) representations are positive and the multiplication and comultiplication preserve positivity. This has led to the notion of a PSH-algebra, which stands for positive self-adjoint Hopf algebra, [a5]. Essentially, 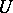 is the unique PSH-algebra on one generator and all other are tensor products of graded shifted copies of
is the unique PSH-algebra on one generator and all other are tensor products of graded shifted copies of 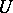 . This can be applied to other series of classical groups than the
. This can be applied to other series of classical groups than the 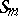 , [a5].
, [a5].
In combinatorics the algebra 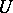 also has a long history. In modern terminology it is at the basis of the so-called umbral calculus, [a7].
also has a long history. In modern terminology it is at the basis of the so-called umbral calculus, [a7].
A modern reference to the representation theory of the symmetric groups, both ordinary and modular, is [a8].
References
| [a1] | A. Liulevicius, "Arrows, symmetries, and representation rings" J. Pure Appl. Algebra , 19 (1980) pp. 259–273 |
| [a2] | M. Hazewinkel, "Formal rings and applications" , Acad. Press (1978) |
| [a3] | M.F. Atiyah, "Power operations in K-theory" Quarterly J. Math. (2) , 17 (1966) pp. 165–193 |
| [a4] | D. Knutson, " -rings and the representation theory of the symmetric group" , Springer (1973) -rings and the representation theory of the symmetric group" , Springer (1973) |
| [a5] | A.V. Zelevinsky, "Representations of finite classical groups" , Springer (1981) |
| [a6] | D.C. Ravenel, "The Hopf ring for complex cobordism" J. Pure Appl. Algebra , 9 (1977) pp. 241–280 |
| [a7] | S. Roman, "The umbral calculus" , Acad. Press (1984) |
| [a8] | G. James, A. Kerber, "The representation theory of the symmetric group" , Addison-Wesley (1981) |
| [a9] | G. de B. Robinson, "Representation theory of the symmetric group" , Univ. Toronto Press (1961) |
| [a10] | J.A. Green, "Polynomial representations of 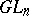 " , Lect. notes in math. , 830 , Springer (1980) " , Lect. notes in math. , 830 , Springer (1980) |
Representation of the symmetric groups. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Representation_of_the_symmetric_groups&oldid=15150