Random element
A generalization of the concept of a random variable. The term "random element" was coined by M. Fréchet [1], who pointed out that the development of probability theory and the extension of its domain of applications had led to the necessity of passing from schemes where the (random) results of an experiment can be described by a number or a finite collection of numbers to schemes where the results of an experiment are, for example, sequences, functions, curves, or transformations.
Subsequently, the term "random element" was used chiefly with reference to "randomly chosen" element in some linear topological space, especially a Hilbert space or a Banach space. The exact definition of a random element  in a Banach space
in a Banach space  , for example, is reminiscent of the definition of a random variable. Let
, for example, is reminiscent of the definition of a random variable. Let  be some probability space, let
be some probability space, let  be a Banach space and let
be a Banach space and let  be the dual space of
be the dual space of  . A mapping
. A mapping  from the space
from the space  of elementary events
of elementary events  into
into  is called a random element if every continuous linear functional
is called a random element if every continuous linear functional 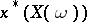 is actually a random variable, that is, an
is actually a random variable, that is, an  -measurable function.
-measurable function.
Let  be the smallest
be the smallest  -algebra of subsets of
-algebra of subsets of  with respect to which all continuous linear functionals are measurable.
with respect to which all continuous linear functionals are measurable.  is a random element if and only if the complete pre-image of all sets in
is a random element if and only if the complete pre-image of all sets in  are
are  -measurable. In the case when
-measurable. In the case when  is separable,
is separable, 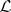 coincides with the
coincides with the  -algebra of Borel subsets of
-algebra of Borel subsets of 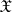 .
.
The basic concepts of probability theory, such as the characteristic function, the mathematical expectation and the covariance, among other things, can be extended to random elements. A random element 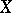 is called normal (Gaussian) if the probability distribution of every continuous linear functional
is called normal (Gaussian) if the probability distribution of every continuous linear functional 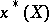 is normal (cf. Normal distribution). The weak law of large numbers, the strong law of large numbers, the law of the iterated logarithm, the central limit theorem, and other assertions of probability theory can be extended to sequences of random elements. Whether these theorems in their classical form carry over to the case of Banach spaces depends on the geometry of the space. It is important to note that this is a two-way connection, in that the probabilistic properties often turn out to be in fact probabilistic-geometric: not only is their validity in a given Banach space determined by the geometric properties of the space, but conversely it determines these properties. E.g., for any sequence of independent identically-distributed random elements
is normal (cf. Normal distribution). The weak law of large numbers, the strong law of large numbers, the law of the iterated logarithm, the central limit theorem, and other assertions of probability theory can be extended to sequences of random elements. Whether these theorems in their classical form carry over to the case of Banach spaces depends on the geometry of the space. It is important to note that this is a two-way connection, in that the probabilistic properties often turn out to be in fact probabilistic-geometric: not only is their validity in a given Banach space determined by the geometric properties of the space, but conversely it determines these properties. E.g., for any sequence of independent identically-distributed random elements 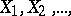 with values in
with values in  , zero mathematical expectations and
, zero mathematical expectations and  , the distribution of the normalized sum
, the distribution of the normalized sum  converges weakly to the distribution of a normal random element as
converges weakly to the distribution of a normal random element as 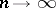 if and only if
if and only if 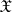 is a so-called type-2 space (see [4]).
is a so-called type-2 space (see [4]).
References
| [1] | M. Fréchet, "Les éléments aléatoires de nature quelconque dans un espace distancié" Ann. Inst. H. Poincaré , 10 (1948) pp. 215–310 |
| [2] | E. Mourier, "Eléments aléatoires dans un espace de Banach" , Paris (1955) (Thése) |
| [3] | N.N. [N.N. Vakhaniya] Vahanija, "Probability distributions on linear spaces" , North-Holland (1981) (Translated from Russian) |
| [4] | J. Hoffmann-Jørgensen, G. Pisier, "The law of large numbers and the central limit theorem in Banach spaces" Ann. Probab. , 4 (1976) pp. 587–599 |
Comments
References
| [a1] | L. Schwartz, "Geometry and probability on Banach spaces" , Springer (1981) |
| [a2] | J. Hoffmann-Jørgensen, "Probability in Banach spaces" , Springer (1977) |
| [a3] | Y. Yamasaki, "Measures on infinite dimensional spaces" , World Sci. (1985) |
| [a4] | N.N. Vakhania, V.I. Tarieladze, S.A. Chobanyan, "Probability distributions on Banach spaces" , Reidel (1987) (Translated from Russian) |
| [a5] | V. Paulaskas, A. Račkauskas, "Approximation theory in the central limit theorem. Exact results in Banach spaces" , Kluwer (1989) (Translated from Russian) |
Random element. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Random_element&oldid=11949