Quadratic reciprocity law
The relation
 |
connecting the Legendre symbols (cf. Legendre symbol)
 |
for different odd prime numbers  and
and 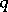 . There are two additions to this quadratic reciprocity law, namely:
. There are two additions to this quadratic reciprocity law, namely:
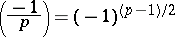 |
and
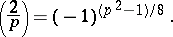 |
C.F. Gauss gave the first complete proof of the quadratic reciprocity law, which for this reason is also called the Gauss reciprocity law.
It immediately follows from this law that for a given square-free number 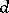 , the primes
, the primes 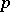 for which
for which 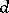 is a quadratic residue modulo
is a quadratic residue modulo 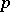 ly in certain arithmetic progressions with common difference
ly in certain arithmetic progressions with common difference 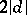 or
or 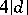 . The number of these progressions is
. The number of these progressions is 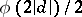 or
or 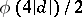 , where
, where 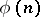 is the Euler function. The quadratic reciprocity law makes it possible to establish factorization laws in quadratic extensions
is the Euler function. The quadratic reciprocity law makes it possible to establish factorization laws in quadratic extensions 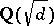 of the field of rational numbers, since the factorization into prime factors in
of the field of rational numbers, since the factorization into prime factors in 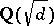 of a prime number that does not divide
of a prime number that does not divide  depends on whether or not
depends on whether or not  is reducible modulo
is reducible modulo 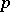 .
.
References
| [1] | I.M. Vinogradov, "Elements of number theory" , Dover, reprint (1954) (Translated from Russian) |
| [2] | Z.I. Borevich, I.R. Shafarevich, "Number theory" , Acad. Press (1966) (Translated from Russian) (German translation: Birkhäuser, 1966) |
Comments
See also Quadratic residue; Dirichlet character.
References
| [a1] | G.H. Hardy, E.M. Wright, "An introduction to the theory of numbers" , Oxford Univ. Press (1979) pp. Chapt. XIII |
Quadratic reciprocity law. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Quadratic_reciprocity_law&oldid=13467