Metric space
A set 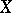 together with a metric
together with a metric 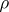 . The set-theoretic approach to the study of figures (spaces) is based on the study of the relative position of their elementary constituents. A fundamental characteristic of the relative position of points of a space is the distance between them. This approach leads to the idea of a metric space, first suggested by M. Fréchet [2] in connection with the discussion of function spaces. It turns out that sets of objects of very different types carry natural metrics. As metric spaces one may consider sets of states, functions and mappings, subsets of Euclidean spaces, and Hilbert spaces. Metrics are important in the study of convergence (of series, functions) and for the solution of questions concerning approximation.
. The set-theoretic approach to the study of figures (spaces) is based on the study of the relative position of their elementary constituents. A fundamental characteristic of the relative position of points of a space is the distance between them. This approach leads to the idea of a metric space, first suggested by M. Fréchet [2] in connection with the discussion of function spaces. It turns out that sets of objects of very different types carry natural metrics. As metric spaces one may consider sets of states, functions and mappings, subsets of Euclidean spaces, and Hilbert spaces. Metrics are important in the study of convergence (of series, functions) and for the solution of questions concerning approximation.
The development of the theory of metric spaces has proceeded in the following main directions.
General theory of metric spaces.
Here one studies properties of metric spaces which are invariant relative to isometries: one-to-one and onto mappings which preserve distance (cf. Isometric mapping). Such properties include completeness, boundedness, total boundedness, and widths. Properties of this type are called metric.
Topological theory of metric spaces.
Its subject is the properties of metric spaces which are preserved under homeomorphisms (cf. Homeomorphism). Among these are compactness, separability, connectedness, the Baire property, and zero dimensionality. Properties of this type are called topological.
Theory of spaces on which a metric has been given that is compatible with some additional algebraic structure (for example, a vector space or a group). Here one is concerned with Euclidean spaces, pre-Hilbert and Hilbert spaces (cf. Hilbert space) (of any weight), Banach spaces, Banach algebras, Banach lattices (cf. Banach space; Banach algebra; Banach lattice), and countably-normed spaces (cf. Countably-normed space). The facts available here are essentially connected with the discussion of important properties of metrics or norms, but the content, on the whole, belongs to the corresponding domains of algebra and functional analysis.
The discussion of particular metrics plays an important role in investigations in non-Euclidean geometries, differential geometry, mechanics, and physics. Here a central place is occupied by the notion of a Riemannian metric in a Riemannian space (see Riemannian geometry). A broader approach to the study of the surfaces and figures that arise in differential geometry is related to the concept of a 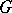 -space, resulting from the addition of certain conditions to the metric axioms (see Geodesic geometry), which creates a basis for the discussion of geodesics in
-space, resulting from the addition of certain conditions to the metric axioms (see Geodesic geometry), which creates a basis for the discussion of geodesics in 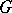 -spaces by ensuring their existence and nice properties. Typical here is the abandoning of the methods of differential calculus. In this connection it turns out that much of differential geometry is not connected with differentiability conditions, but is determined only by geometric axioms. Geodesic geometry is of interest not only as a generalization of Riemannian geometry, but also as an attempt to investigate geometric objects more geometrically, without using complicated computations.
-spaces by ensuring their existence and nice properties. Typical here is the abandoning of the methods of differential calculus. In this connection it turns out that much of differential geometry is not connected with differentiability conditions, but is determined only by geometric axioms. Geodesic geometry is of interest not only as a generalization of Riemannian geometry, but also as an attempt to investigate geometric objects more geometrically, without using complicated computations.
In each set  a metric
a metric 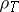 can be defined by the following rule:
can be defined by the following rule: 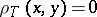 if
if 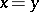 , and
, and 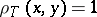 if
if 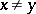 . This is called the trivial metric. Each metric
. This is called the trivial metric. Each metric 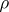 on a set
on a set 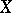 gives rise, in a natural way, to a topology
gives rise, in a natural way, to a topology 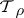 on
on 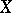 . The concept of a topological space axiomatizes the relation of absolute nearness of a point to a set, whereas the concept of a metric space formalizes the notion of relative nearness of points. The distance
. The concept of a topological space axiomatizes the relation of absolute nearness of a point to a set, whereas the concept of a metric space formalizes the notion of relative nearness of points. The distance 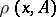 of a point
of a point  to a set
to a set 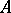 in a metric space
in a metric space 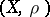 is defined as
is defined as 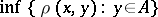 . A point
. A point  is said to be absolutely near to a set
is said to be absolutely near to a set 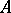 if
if 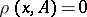 . The closure
. The closure 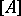 of
of 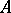 in
in 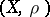 is the set of all points of
is the set of all points of 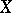 absolutely near to
absolutely near to 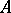 . The topology in
. The topology in 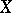 uniquely associated with this operation is called the topology generated in
uniquely associated with this operation is called the topology generated in 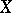 by the metric
by the metric 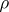 . The trivial metric leads to the trivial topology, in which all sets are closed.
. The trivial metric leads to the trivial topology, in which all sets are closed.
In research on metric spaces (particularly on their topological properties) the idea of a convergent sequence plays an important role. This is explained by the fact that the topology of a metric space can be completely described in the language of sequences.
Let  be a sequence of points in a metric space
be a sequence of points in a metric space 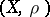 . It is said to converge to a point
. It is said to converge to a point 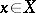 if for each
if for each 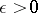 there is an integer
there is an integer 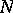 such that
such that  for all
for all 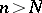 . The sequence
. The sequence 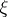 is called fundamental if for each
is called fundamental if for each 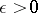 there is an integer
there is an integer 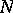 such that
such that  for all
for all  .
.
An important metric property is completeness. A metric space 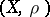 is called complete if each fundamental sequence in it converges to a point of it. The space
is called complete if each fundamental sequence in it converges to a point of it. The space 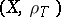 is always complete. Completeness of a metric space is not a topological property: A metric space homeomorphic to a complete metric space may be non-complete, for example, the real line
is always complete. Completeness of a metric space is not a topological property: A metric space homeomorphic to a complete metric space may be non-complete, for example, the real line 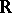 with the usual metric
with the usual metric  is homeomorphic to the interval
is homeomorphic to the interval 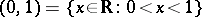 with the same metric; however, the first metric space is complete and the second is not.
with the same metric; however, the first metric space is complete and the second is not.
Examples of complete metric spaces are Euclidean and Banach spaces. An important property of complete metric spaces, preserved under homeomorphisms, is the Baire property, on the strength of which each complete metric space without isolated points is uncountable. Therefore the usual topological space of the rational numbers is not generated by any complete metric. However, each metric space may be represented as a subset of some complete metric space by the standard construction of completion. Two fundamental sequences 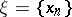 and
and 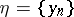 in a metric space
in a metric space 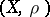 are called equivalent if
are called equivalent if
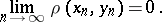 |
Let 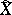 be the resulting collection of equivalence classes. A metric
be the resulting collection of equivalence classes. A metric 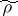 is introduced on
is introduced on 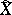 by the rule: If
by the rule: If 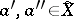 and
and 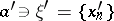 ,
, 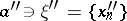 , then
, then
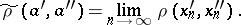 |
For 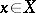 , let
, let 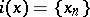 , where
, where 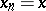 for all
for all  . Then
. Then 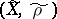 is a complete metric space and
is a complete metric space and 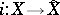 is an isometry of
is an isometry of 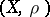 onto an everywhere-dense subset in
onto an everywhere-dense subset in 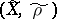 (
(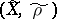 is called the completion of
is called the completion of 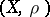 ).
).
Related to the discussion of completion is the Lavrent'ev theorem on the extension of homeomorphisms. This theorem implies that the property of a metric space being a 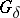 -set in its completion is a topological invariant (in contrast with the non-invariance of metric completeness itself relative to homeomorphisms).
-set in its completion is a topological invariant (in contrast with the non-invariance of metric completeness itself relative to homeomorphisms).
Two metrics 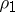 and
and 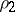 on a set
on a set 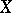 are called topologically equivalent if the topologies
are called topologically equivalent if the topologies 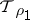 and
and 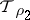 generated by them coincide. On a finite set all metrics are equivalent; they generate the discrete topology. The Aleksandrov–Hausdorff theorem: A metric
generated by them coincide. On a finite set all metrics are equivalent; they generate the discrete topology. The Aleksandrov–Hausdorff theorem: A metric 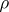 on a set
on a set 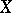 is topologically equivalent to some complete metric if and only if
is topologically equivalent to some complete metric if and only if 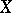 is a
is a 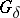 -set in the completion of
-set in the completion of 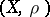 . In particular, the space of irrational numbers with the usual metric, relative to which it is not complete, is homeomorphic to the complete metric Baire space whose points are the infinite sequences
. In particular, the space of irrational numbers with the usual metric, relative to which it is not complete, is homeomorphic to the complete metric Baire space whose points are the infinite sequences 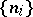 of natural numbers, with distance given by
of natural numbers, with distance given by 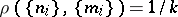 , where
, where 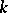 is such that
is such that  and
and  for all
for all 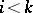 .
.
The following example of a complete metric space is important: The space 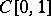 of all continuous functions on
of all continuous functions on 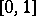 , with metric defined by the rule
, with metric defined by the rule
 |
for all 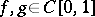 . The space
. The space  is separable — there is a countable everywhere-dense set in it (cf. Separable space). It turns out that each separable metric space is isometric to some subset of
is separable — there is a countable everywhere-dense set in it (cf. Separable space). It turns out that each separable metric space is isometric to some subset of  (the Banach–Mazur theorem). This result means that all metrics which generate separable topologies are obtained by restricting the natural metric on the set of continuous functions.
(the Banach–Mazur theorem). This result means that all metrics which generate separable topologies are obtained by restricting the natural metric on the set of continuous functions.
A subset 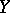 of a complete metric space
of a complete metric space 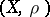 , equipped with the same metric
, equipped with the same metric  (more precisely, its restriction to
(more precisely, its restriction to 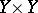 ), is a complete metric space if and only if
), is a complete metric space if and only if 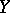 is closed in
is closed in 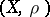 .
.
There is a fundamental connection between the ideas of completeness and compactness for a metric space. Compactness of a metric space 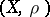 is equivalent to any of the following conditions: 1) any sequence in
is equivalent to any of the following conditions: 1) any sequence in 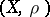 contains a convergent subsequence; 2) each countable open covering (cf. Covering (of a set)) of
contains a convergent subsequence; 2) each countable open covering (cf. Covering (of a set)) of 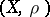 contains a finite subcovering; 3) in any open covering of
contains a finite subcovering; 3) in any open covering of 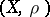 there is a finite subcovering; 4) each decreasing sequence of non-empty closed sets in
there is a finite subcovering; 4) each decreasing sequence of non-empty closed sets in 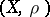 has a non-empty intersection; and 5) every closed discrete subset of
has a non-empty intersection; and 5) every closed discrete subset of 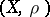 is finite.
is finite.
The simplest examples of compact metric spaces are: finite discrete spaces, any interval (together with its end points), a square, a circle, and a sphere. In general, a subset of the Euclidean space 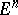 , with the usual metric, is compact if and only if it is closed and bounded.
, with the usual metric, is compact if and only if it is closed and bounded.
The conditions listed are not all equivalent outside the class of metric spaces (see Compact space). H. Lebesgue (1911) established that for each open covering 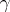 of a compact metric space
of a compact metric space 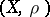 there is a number
there is a number 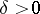 such that each set
such that each set 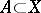 of diameter
of diameter 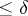 is contained in some element of
is contained in some element of 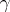 . This implies the fundamental property of compact metric spaces: Every continuous mapping of such a space into an arbitrary metric space is uniformly continuous (cf. Uniform continuity). Further, a metric space is compact if and only if each real-valued continuous function on it is bounded (and attains its least and greatest values).
. This implies the fundamental property of compact metric spaces: Every continuous mapping of such a space into an arbitrary metric space is uniformly continuous (cf. Uniform continuity). Further, a metric space is compact if and only if each real-valued continuous function on it is bounded (and attains its least and greatest values).
Each compact metric space is complete, but the converse is false; the simplest example is an infinite discrete space with the trivial metric. However, the following characteristic property holds: A metric space is compact if and only if every metric space homeomorphic to it is complete.
It is intuitively clear that compactness implies, besides completeness, some sort of boundedness; this is confirmed by the consideration of compact subsets of 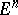 . In general, a metric space
. In general, a metric space 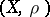 is called bounded if there is a real number
is called bounded if there is a real number  such that
such that 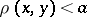 for all
for all 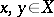 . Every compact metric space is bounded. The space
. Every compact metric space is bounded. The space 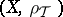 is complete and bounded, but not compact if
is complete and bounded, but not compact if 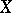 is infinite; thus, completeness and boundedness together are not sufficient for compactness in the class of metric spaces. In general, each metric on a set is topologically equivalent to some bounded metric, which is complete if the given metric is complete. In this connection, there is the important notion of total boundedness. A metric space
is infinite; thus, completeness and boundedness together are not sufficient for compactness in the class of metric spaces. In general, each metric on a set is topologically equivalent to some bounded metric, which is complete if the given metric is complete. In this connection, there is the important notion of total boundedness. A metric space 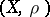 is called totally bounded if for each
is called totally bounded if for each 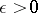 there is a finite set
there is a finite set 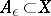 such that
such that 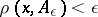 for all
for all 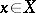 . The set
. The set 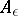 here is called an
here is called an  -net in
-net in 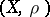 . A metric space
. A metric space 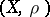 is compact if and only if it is complete and totally bounded, and
is compact if and only if it is complete and totally bounded, and 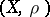 is totally bounded if and only if it is isometric to a subset of some compact metric space. More precisely, total boundedness of a metric space is equivalent to compactness of its completion
is totally bounded if and only if it is isometric to a subset of some compact metric space. More precisely, total boundedness of a metric space is equivalent to compactness of its completion 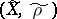 . Each subspace of a totally-bounded metric space is totally bounded. All totally-bounded metric spaces (in particular, all compact metric spaces) are separable and have a countable base. Compactness, in general, is not inherited by subsets; a set
. Each subspace of a totally-bounded metric space is totally bounded. All totally-bounded metric spaces (in particular, all compact metric spaces) are separable and have a countable base. Compactness, in general, is not inherited by subsets; a set 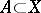 is relatively compact in a metric space
is relatively compact in a metric space 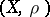 if the closure of
if the closure of 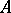 in
in  is a compact metric space. If
is a compact metric space. If 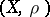 is complete, then relative compactness of a set
is complete, then relative compactness of a set 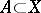 in
in 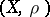 is equivalent to total boundedness of
is equivalent to total boundedness of 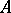 equipped with the metric
equipped with the metric 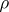 .
.
An important role in functional analysis is played by a criterion for compactness of a set 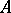 of continuous functions on
of continuous functions on 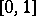 in the metric space
in the metric space 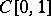 . This criterion is the following (the Arzelà–Ascoli theorem): A set
. This criterion is the following (the Arzelà–Ascoli theorem): A set  is relatively compact in
is relatively compact in 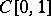 if and only if: 1) there is a number
if and only if: 1) there is a number 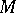 such that
such that 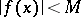 for all
for all 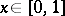 and all
and all 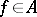 ; and 2) for each
; and 2) for each 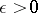 there is a
there is a 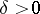 such that
such that 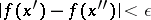 for all
for all 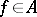 and all
and all 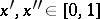 for which
for which 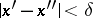 .
.
A mapping 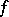 of a metric space
of a metric space 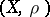 into itself is called a contraction if there is a real number
into itself is called a contraction if there is a real number 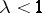 such that
such that
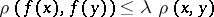 |
for all 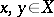 . An important theorem for complete metric spaces is the contracting- (contraction-) mapping principle (cf. also Contraction-mapping principle): For each such mapping of a (non-empty) complete metric space
. An important theorem for complete metric spaces is the contracting- (contraction-) mapping principle (cf. also Contraction-mapping principle): For each such mapping of a (non-empty) complete metric space 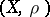 into itself there is precisely one fixed point.
into itself there is precisely one fixed point.
The topological theory of metric spaces is significantly simpler than the general theory of topological spaces. Below the most important topological properties of metric spaces 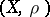 are given. Here one has in mind properties of the topology
are given. Here one has in mind properties of the topology 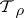 that is generated by the metric
that is generated by the metric  .
.
Each metric space is normal and even collectionwise normal (cf. Normal space). This permits the extension of continuous real-valued functions from closed subsets of a metric space to the whole space. A stronger result is: For each closed subset 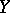 of a metric space
of a metric space 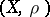 there is a linear mapping
there is a linear mapping 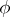 of the space of all continuous real-valued functions on
of the space of all continuous real-valued functions on 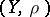 to the space of all continuous real-valued functions on
to the space of all continuous real-valued functions on  such that (for any
such that (for any 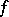 )
) 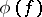 is an extension of
is an extension of 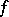 and
and
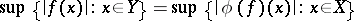 |
(Dugundji's theorem). This theorem is related to Hausdorff's theorem on the extension of metrics: If a closed subspace 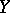 of a metrizable space
of a metrizable space 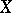 is already metrizable with a metric
is already metrizable with a metric 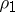 (generating the topology on
(generating the topology on 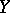 as a subspace of
as a subspace of  ), then it is possible to extend
), then it is possible to extend  to a metric
to a metric 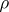 on the whole of
on the whole of 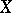 , generating the original topology on
, generating the original topology on 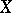 . Similar results are valid for totally-bounded metrics and complete metrics.
. Similar results are valid for totally-bounded metrics and complete metrics.
Research into the topological properties of metric spaces is, to a large extent, based on the following theorem of A.H. Stone: A metric space is paracompact, that is, any open covering 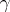 has an open locally finite refinement
has an open locally finite refinement 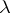 (locally finite means that each point has a neighbourhood intersecting only a finite number of elements of
(locally finite means that each point has a neighbourhood intersecting only a finite number of elements of 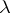 , cf. also Paracompact space). The Nagata–Smirnov metrization criterion (see Metrizable space) is based on the paracompactness of metric spaces.
, cf. also Paracompact space). The Nagata–Smirnov metrization criterion (see Metrizable space) is based on the paracompactness of metric spaces.
For a metric space there are important theorems on the equivalence of topological properties which are distinct in general topological spaces. Thus, the following cardinal-valued invariants coincide: the density, the character, the weight, the Suslin number, and the Lindelöf number (cf. also Cardinal characteristic). For metric spaces countable compactness, pseudo-compactness and compactness are equivalent. For metric spaces the dimensions 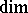 (the covering dimension) and
(the covering dimension) and 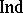 (the large inductive dimension) coincide, and for separable metric spaces the small inductive dimension
(the large inductive dimension) coincide, and for separable metric spaces the small inductive dimension 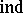 coincides with
coincides with 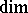 and
and 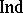 (see Dimension theory).
(see Dimension theory).
Each metric space 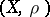 is star normal; any open covering
is star normal; any open covering 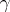 of
of 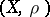 has an open star refinement
has an open star refinement 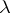 , that is, for each point
, that is, for each point 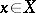 there is a
there is a 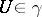 containing every
containing every 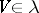 for which
for which 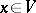 . Related to this theorem is the following metrizability criterion (Stone–Arkhangel'skii): A regular space is metrizable by a totally-bounded metric if the space has a countable base. But even a countable regular space need not be metrizable. The simplest example is obtained by adjoining to the discrete natural numbers one exterior point from its Stone–Čech compactification. The criterion for metrizability of a metric space
. Related to this theorem is the following metrizability criterion (Stone–Arkhangel'skii): A regular space is metrizable by a totally-bounded metric if the space has a countable base. But even a countable regular space need not be metrizable. The simplest example is obtained by adjoining to the discrete natural numbers one exterior point from its Stone–Čech compactification. The criterion for metrizability of a metric space 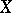 by a complete metric is unexpected: It is necessary and sufficient that
by a complete metric is unexpected: It is necessary and sufficient that 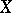 be a
be a 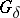 -set in some (and then in any) Hausdorff compactification of
-set in some (and then in any) Hausdorff compactification of  . However, Hausdorff compactifications of metric spaces carry complete information on the topology of the latter; this is clear from Čech's theorem: Metric spaces are homeomorphic if and only if their Stone–Čech compactifications are homeomorphic.
. However, Hausdorff compactifications of metric spaces carry complete information on the topology of the latter; this is clear from Čech's theorem: Metric spaces are homeomorphic if and only if their Stone–Čech compactifications are homeomorphic.
A metric space need not have a countable base, but it always satisfies the first axiom of countability: it has a countable base at each point. In addition, each compact set in a metric space has a countable base. Moreover, in each metric space there is a base such that each point of the space belongs to only countably many of its elements — a point-countable base, but this property is weaker than metrizability, even for paracompact Hausdorff spaces. Regular separable spaces satisfying the first axiom of countability need not be metrizable.
The condition for metrizability of a separated topological group is easily found: It is necessary and sufficient that the space of the group satisfies the first axiom of countability; there are then both left-invariant and right-invariant metrics generating the topology.
Connected with each metric space 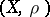 , in a standard way, there is another metric space, namely the space
, in a standard way, there is another metric space, namely the space 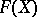 of its non-empty bounded closed subsets with the Hausdorff metric, which is defined as follows:
of its non-empty bounded closed subsets with the Hausdorff metric, which is defined as follows:
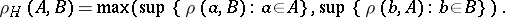 |
The space 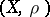 is isometric to a closed subspace of
is isometric to a closed subspace of 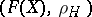 . If
. If 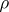 is complete, then
is complete, then 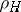 is complete. But topological equivalence of two metrics
is complete. But topological equivalence of two metrics 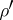 and
and 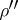 given on
given on 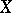 does not imply, in general, that the corresponding Hausdorff metrics
does not imply, in general, that the corresponding Hausdorff metrics 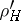 and
and  are topologically equivalent.
are topologically equivalent.
A continuous image of a metric space need not be homeomorphic to any metric space, even when the Hausdorff separation axiom is satisfied. This also applies to quotient spaces of metric spaces. For example, if in the plane one shrinks a fixed line to a point, taking as individual elements of the decomposition all the points of the plane not on the line, then one obtains a non-metrizable separable space, at whose special point the first axiom of countability is not satisfied. There is a general criterion for the metrizability of a quotient space of a metric space (see [6]). In particular, the quotient space associated with a continuous decomposition of a metric space into compact sets is always metrizable. Every Hausdorff space which is a continuous image of a compact metric space is metrizable and compact. This is a particular case of a general proposition on the non-increase of the weight of a topological space under a continuous mapping into a compact set. But even when the image 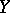 of a metric space
of a metric space 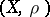 is metrizable, the metric realizing the metrization of
is metrizable, the metric realizing the metrization of 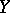 need not be obtained from
need not be obtained from  by means of any formula. Instead of a metric on
by means of any formula. Instead of a metric on 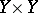 , with respect to
, with respect to 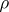 it is natural to define a function
it is natural to define a function 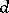 by means of the rule: For
by means of the rule: For 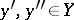 ,
,  is equal to the distance in the sense of
is equal to the distance in the sense of 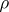 between the inverse images of the points
between the inverse images of the points 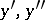 under the mapping under discussion. Often (for example, if
under the mapping under discussion. Often (for example, if  is the decomposition space of a metric space into compact sets)
is the decomposition space of a metric space into compact sets) 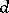 is closely related to the topology of
is closely related to the topology of 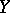 and is symmetric. This means that
and is symmetric. This means that  for all
for all 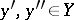 ,
, 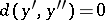 if and only if
if and only if 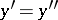 . The symmetric relation
. The symmetric relation 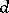 thus defined almost never satisfies the triangle axiom (cf. Metric), but if the decomposition into compact sets is continuous, then
thus defined almost never satisfies the triangle axiom (cf. Metric), but if the decomposition into compact sets is continuous, then  has topological properties which successfully replace the triangle axiom and guarantee the metrizability of the image by a "true" metric. A topological space which is the image of a metric space under a continuous open and closed mapping is itself homeomorphic to a metric space. However, under continuous open mappings, metrizability is not always preserved: All spaces satisfying the first axiom of countability, and only they, are the images of metric spaces under continuous open mappings.
has topological properties which successfully replace the triangle axiom and guarantee the metrizability of the image by a "true" metric. A topological space which is the image of a metric space under a continuous open and closed mapping is itself homeomorphic to a metric space. However, under continuous open mappings, metrizability is not always preserved: All spaces satisfying the first axiom of countability, and only they, are the images of metric spaces under continuous open mappings.
Among the generalizations of metric spaces the most important are pseudo-metric spaces, spaces with a symmetric relation and spaces with  -metrics [7]. These are defined axiomatically by a natural weakening of the axioms of a metric space. However, the distance here, as usual, is expressed by a non-negative real number. One may consider generalized metrics with values in ordered semi-groups, semi-fields, etc. (see [8]). In this way a generalized metrization of an arbitrary completely-regular space can be obtained.
-metrics [7]. These are defined axiomatically by a natural weakening of the axioms of a metric space. However, the distance here, as usual, is expressed by a non-negative real number. One may consider generalized metrics with values in ordered semi-groups, semi-fields, etc. (see [8]). In this way a generalized metrization of an arbitrary completely-regular space can be obtained.
A fundamental generalization of the concept of a metric space is the notion of a uniform space. Further, there are purely topological extensions of the class of metric spaces, among which are the important classes of spaces with a uniform base, Moore spaces, feathered and paracompact feathered spaces, and lattice spaces (cf. Moore space; Feathered space). The class of paracompact spaces is too broad a generalization of the class of metric spaces, because paracompactness is not even preserved under finite products. On the contrary, the class of paracompact feathered spaces is a successful simultaneous generalization of the class of spaces homeomorphic to metric spaces and the class of compact spaces. In another direction the idea of a metric generalizes to a  -metric and a
-metric and a  -metric [4]. The concept of a statistical metric space, introduced by K. Menger, turns out to be topologically equivalent to the idea of a space with a symmetric relation.
-metric [4]. The concept of a statistical metric space, introduced by K. Menger, turns out to be topologically equivalent to the idea of a space with a symmetric relation.
References
| [1] | P.S. Aleksandrov, "Einführung in die Mengenlehre und die allgemeine Topologie" , Deutsch. Verlag Wissenschaft. (1984) (Translated from Russian) |
| [2] | M. Fréchet, "Sur quelques points du calcul fonctionnel" Rend. Circ. Mat. Palermo , 22 (1906) pp. 1–74 |
| [3] | A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian) |
| [4] | E.V. Shchepin, "Topology of limit spaces of uncountable inverse spectra" Russian Math. Surveys , 31 : 5 (1976) pp. 155–191 Uspekhi Mat. Nauk , 31 : 5 (1976) pp. 191–226 |
| [5] | R. Engelking, "General topology" , Heldermann (1989) |
| [6] | A.V. Arkhangel'skii, "Factor-mappings of metric spaces" Soviet Math. Dokl. , 5 : 2 (1964) pp. 368–371 Dokl. Akad. Nauk SSSR , 155 : 2 (1964) pp. 247–250 |
| [7] | S.I. Nedev, " -metrizable spaces" Trans. Moscow Math. Soc. , 24 (1971) pp. 213–247 Trudy Moskov. Mat. Obshch. , 24 (1971) pp. 201–236 -metrizable spaces" Trans. Moscow Math. Soc. , 24 (1971) pp. 213–247 Trudy Moskov. Mat. Obshch. , 24 (1971) pp. 201–236 |
| [8] | M.Ya. Antonovskii, V.G. Boltyanskii, T.A. Sarymsakov, "An outline of the theory of topological semi-fields" Russian Math. Surveys , 21 : 4 (1966) pp. 163–192 Uspekhi Mat. Nauk , 21 : 4 (1966) pp. 185–218 |
| [9] | S.I. Nedev, M.M. Choban, " -metrics and proximity spaces. Metrization of proximity spaces" Serdica , 1 (1975) pp. 12–28 (In Russian) -metrics and proximity spaces. Metrization of proximity spaces" Serdica , 1 (1975) pp. 12–28 (In Russian) |
Comments
The trivial metric is also called the discrete metric. A fundamental sequence is also called a Cauchy sequence. Star-normal spaces are also called fully normal.
There are fairly obvious numerical invariants of metric spaces such as width (diameter) and (various kinds of) dimension. A rather more hidden numerical invariant is the Gross dispersion number or rendezvous number, whose existence and uniqueness is guaranteed by the following theorem, [a12]. Let 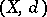 be a compact connected metric space; then there is a unique number
be a compact connected metric space; then there is a unique number 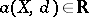 such that for all
such that for all 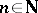 and all sets of
and all sets of  points
points 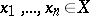 there is a point
there is a point 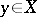 such that
such that
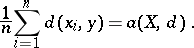 | (a1) |
Some examples are as follows. If 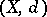 is a ball of radius
is a ball of radius 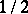 in Euclidean
in Euclidean  -space, then
-space, then 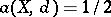 ; if
; if 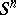 is the
is the  -dimensional sphere of unit diameter in Euclidean
-dimensional sphere of unit diameter in Euclidean 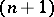 -space, then, [a15],
-space, then, [a15],
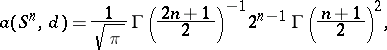 |
where 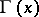 is the gamma-function; if
is the gamma-function; if 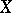 is an equilateral triangle in
is an equilateral triangle in 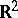 , then
, then  . The theorem guaranteeing the existence of
. The theorem guaranteeing the existence of 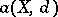 generalizes in two directions. First,
generalizes in two directions. First, 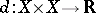 can be replaced by any symmetric function
can be replaced by any symmetric function 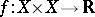 (where symmetric means
(where symmetric means  ), [a13], and further the average on the left of (a1) can be replaced by an integral, [a14]. Thus, for a compact connected Hausdorff space
), [a13], and further the average on the left of (a1) can be replaced by an integral, [a14]. Thus, for a compact connected Hausdorff space 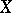 and a symmetric function
and a symmetric function  there exists a unique real number
there exists a unique real number 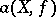 such that for any regular Borel probability measure
such that for any regular Borel probability measure 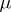 on
on 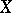 there is a point
there is a point 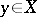 such that
such that
 |
The metric spaces on which every continuous function (to any metric space, or just to the real line) is uniformly continuous are studied in [a2]. The simplest description is this: There is a compact subset 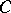 such that the complement of any neighbourhood of
such that the complement of any neighbourhood of 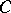 is discrete.
is discrete.
Beyond the completion of a metric space 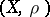 is the injective envelope
is the injective envelope 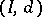 . In general,
. In general, 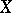 is not dense in
is not dense in 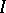 (e.g., if
(e.g., if 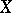 is a circle,
is a circle, 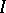 is infinite dimensional), but
is infinite dimensional), but 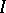 is an essential extension of
is an essential extension of 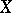 ; this means that a non-expansive mapping
; this means that a non-expansive mapping 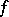 from
from 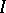 to any metric space
to any metric space 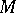 whose restriction to
whose restriction to 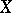 preserves all distances must preserve all distances in
preserves all distances must preserve all distances in 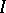 (non-expansive means that
(non-expansive means that 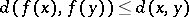 for all
for all 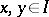 ).
). 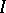 is characterized, up to a unique isometry, as an essential extension of
is characterized, up to a unique isometry, as an essential extension of 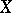 which has no further essential extension. This is equivalent to injectivity in the sense of extendability of mappings, and also to the following Helly-type property: Any family of spherical neighbourhoods
which has no further essential extension. This is equivalent to injectivity in the sense of extendability of mappings, and also to the following Helly-type property: Any family of spherical neighbourhoods 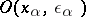 satisfying the consistency conditions
satisfying the consistency conditions  , for all
, for all  , has a common point. Because of this equivalence, the injective metric spaces are also called hyperconvex. See [a1], [a5].
, has a common point. Because of this equivalence, the injective metric spaces are also called hyperconvex. See [a1], [a5].
The injective envelope of a real Banach space is itself a real Banach space in a unique compatible way. This result is known only from a highly non-constructive proof combining H. Cohen's construction of relative injective envelopes in the category of real Banach spaces [a3], the Aronszain–Panitchpakdi theorem that an injective real Banach algebra is an injective metric space [a1], and the Mazur–Ulam theorem that every isometry of real Banach spaces is affine [a9]. Compare [a6].
Injective spaces support a much stronger fixed-point theorem than the contraction-mapping theorem: Every non-expansive mapping of a bounded injective metric space into itself has a fixed point (the Sine–Soardi theorem). However, the extensive development of fixed-point theory of non-expansive mappings has been done mostly in the important special case of convex subsets of Banach spaces. A survey of it, up to 1980, is in [a8]. Compare [a7].
It should be noted that the Stone–Arkhangel'skii metrization criterion involves A.H. Stone, who also proved the paracompactness of metric spaces. In the Stone–Čech compactification, it is M.H. Stone.
It was noted above that two topologically-equivalent metrics on a space  do not, in general, give topologically-equivalent Hausdorff metrics on the hyperspace
do not, in general, give topologically-equivalent Hausdorff metrics on the hyperspace 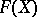 . In fact, they do so if and only if they are uniformly equivalent [a4].
. In fact, they do so if and only if they are uniformly equivalent [a4].
References
| [a1] | N. [N. Aronszain] Aronszajn, P. Panitchpakdi, "Extensions of uniformly continuous transformations and hyperconvex metric spaces" Pacific J. Math. , 6 (1956) pp. 405–439 |
| [a2] | M. Atsuji, "Uniform continuity of continuous functions of metric spaces" Pacific J. Math. , 8 (1958) pp. 11–16 |
| [a3] | H. Cohen, "Injective envelopes of Banach spaces" Bull. Amer. Math. Soc. , 70 (1964) pp. 723–726 |
| [a4] | D. Hammond Smith, "Hyperspaces of a uniformizable space" Proc. Cambridge Philos. Soc. , 62 (1966) pp. 25–28 |
| [a5] | J. Isbell, "Six theorems about injective metric spaces" Comment. Math. Helv. , 39 (1964) pp. 65–76 |
| [a6] | J. Isbell, "Three remarks on injective envelopes of Banach spaces" J. Math. Anal. Appl. , 66 (1969) pp. 301–306 |
| [a7] | V.I. Istraţescu, "Fixed point theory" , Reidel (1981) |
| [a8] | W.A. Kirk, "Fixed point theory for nonexpansive mappings" , Fixed Point Theory , Lect. notes in math. , 886 , Springer (1981) pp. 484–505 |
| [a9] | S. Mazur, S. Ulam, "Sur les transformations isométriques d'espaces vectoriels" C.R. Acad. Sci. Paris , 194 (1932) pp. 946–948 |
| [a10] | J. Dugundji, "Topology" , Allyn & Bacon (1966) |
| [a11] | J.L. Kelley, "General topology" , Springer (1975) |
| [a12] | O. Gross, "The rendezvous value of a metric space" M. Dresher (ed.) L.S. Shapley (ed.) A.W. Tucker (ed.) , Advances in game theory , Princeton Univ. Press (1964) pp. 49–53 |
| [a13] | W. Stadje, "A property of compact connected spaces" Arch. Math. , 36 (1981) pp. 275–280 |
| [a14] | J. Cleary, S.A. Morris, D. Yost, "Numerical geometry—numbers for shapes" Amer. Math. Monthly , 93 (1986) pp. 260–275 |
| [a15] | S.A. Morris, P. Nickolas, "On the average distance property of compact spaces" Arch. Math. , 40 (1983) pp. 459–463 |
Metric space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Metric_space&oldid=13873