Lyapunov stability
of a point relative to a family of mappings
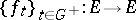 |
of a certain space 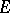
Equicontinuity of this family of mappings at the point (here  is the set of non-negative numbers in
is the set of non-negative numbers in  ; for example, the real numbers
; for example, the real numbers 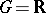 or the integers
or the integers  ). Lyapunov stability of a point relative to the family of mappings
). Lyapunov stability of a point relative to the family of mappings
is equivalent to the continuity at this point of the mapping 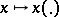 of a neighbourhood of this point into the set of functions
of a neighbourhood of this point into the set of functions 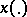 defined by the formula
defined by the formula 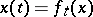 , equipped with the topology of uniform convergence on
, equipped with the topology of uniform convergence on  . Lyapunov stability of a point relative to a mapping is defined as Lyapunov stability relative to the family of non-negative powers of this mapping. Lyapunov stability of a point relative to a dynamical system
. Lyapunov stability of a point relative to a mapping is defined as Lyapunov stability relative to the family of non-negative powers of this mapping. Lyapunov stability of a point relative to a dynamical system  is Lyapunov stability of this point relative to the family
is Lyapunov stability of this point relative to the family 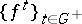 . Lyapunov stability of the solution
. Lyapunov stability of the solution  of an equation
of an equation 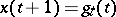 given on
given on 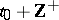 is Lyapunov stability of the point
is Lyapunov stability of the point 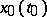 relative to the family of mappings
relative to the family of mappings 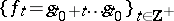 .
.
Lyapunov stability of the solution 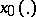 of a differential equation
of a differential equation 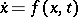 given on
given on 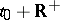 is Lyapunov stability of the point
is Lyapunov stability of the point 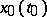 relative to the family of mappings
relative to the family of mappings 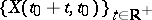 , where
, where 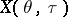 is the Cauchy operator of this equation. Lyapunov stability of the solution
is the Cauchy operator of this equation. Lyapunov stability of the solution 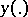 of a differential equation
of a differential equation
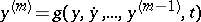 |
of order 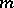 , given on
, given on 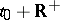 , is Lyapunov stability of the solution
, is Lyapunov stability of the solution 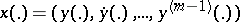 of the corresponding first-order differential equation
of the corresponding first-order differential equation 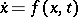 , given on
, given on 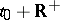 , where
, where
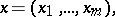 |
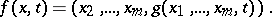 |
The definitions 1–7 given below are some concrete instances of the above and related definitions.
1) Let a differential equation 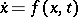 be given, where
be given, where  lies in an
lies in an  -dimensional normed space
-dimensional normed space 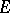 . A solution
. A solution 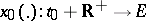 of this equation is called Lyapunov stable if for every
of this equation is called Lyapunov stable if for every 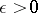 there exists a
there exists a 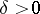 such that for every
such that for every  satisfying the inequality
satisfying the inequality 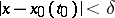 , the solution
, the solution 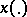 of the Cauchy problem
of the Cauchy problem
 |
is unique, defined on  , and for each
, and for each 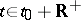 satisfies the inequality
satisfies the inequality  . If, in addition, one can find a
. If, in addition, one can find a 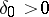 such that for every solution
such that for every solution 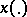 of the equation
of the equation 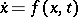 whose initial value satisfies the inequality
whose initial value satisfies the inequality
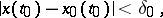 |
the equation
 |
holds (respectively, the inequality
 |
holds; here and elsewhere one puts 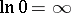 ), then the solution
), then the solution 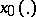 is called asymptotically (respectively, exponentially) stable.
is called asymptotically (respectively, exponentially) stable.
A solution of the equation
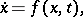 | (2) |
where  or
or 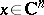 , is called Lyapunov stable (asymptotically, exponentially stable) if it becomes such after equipping the space
, is called Lyapunov stable (asymptotically, exponentially stable) if it becomes such after equipping the space 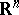 (or
(or 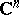 ) with a norm. This property of the solution does not depend on the choice of the norm.
) with a norm. This property of the solution does not depend on the choice of the norm.
2) Let a mapping 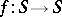 be given, where
be given, where 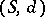 is a metric space. The point
is a metric space. The point 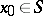 is called Lyapunov stable relative to the mapping
is called Lyapunov stable relative to the mapping 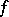 if for every
if for every 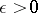 there exists a
there exists a 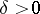 such that for any
such that for any  satisfying the inequality
satisfying the inequality  , the inequality
, the inequality
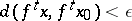 |
holds for each 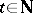 . If, moreover, one can find a
. If, moreover, one can find a 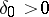 such that for each
such that for each 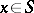 satisfying
satisfying 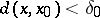 one has the equation
one has the equation
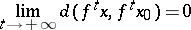 |
(the inequality
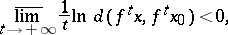 |
respectively), then the point 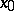 is called asymptotically (respectively, exponentially) stable relative to
is called asymptotically (respectively, exponentially) stable relative to  .
.
Let 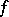 be a mapping from a compact topological space
be a mapping from a compact topological space  into itself. A point
into itself. A point  is called Lyapunov stable (asymptotically stable) relative to
is called Lyapunov stable (asymptotically stable) relative to 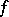 if it becomes such after equipping
if it becomes such after equipping  with a metric. This property of the point does not depend on the choice of the metric.
with a metric. This property of the point does not depend on the choice of the metric.
If 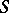 is a compact differentiable manifold, then a point
is a compact differentiable manifold, then a point 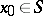 is called exponentially stable relative to a mapping
is called exponentially stable relative to a mapping  if it becomes such after equipping
if it becomes such after equipping  with a certain Riemannian metric. This property of the point does not depend on the choice of the Riemannian metric.
with a certain Riemannian metric. This property of the point does not depend on the choice of the Riemannian metric.
3) Suppose that a differential equation (2) is given, where  lies in a topological vector space
lies in a topological vector space  . A solution
. A solution 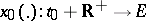 of this equation is called Lyapunov stable if for each neighbourhood of zero
of this equation is called Lyapunov stable if for each neighbourhood of zero 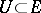 there is a neighbourhood
there is a neighbourhood  of
of  in
in 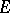 such that for every
such that for every 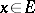 the solution
the solution 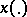 of the Cauchy problem (2),
of the Cauchy problem (2),  , is unique, defined on
, is unique, defined on 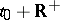 and satisfies the relation
and satisfies the relation 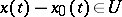 for all
for all  . If, in addition, one can find a neighbourhood
. If, in addition, one can find a neighbourhood 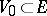 of the point
of the point 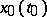 such that for every solution
such that for every solution  of (2) satisfying
of (2) satisfying 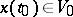 one has the equation
one has the equation
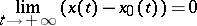 |
(respectively,
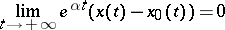 |
for a certain 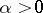 ), then the solution
), then the solution  is called asymptotically (respectively, exponentially) stable. If
is called asymptotically (respectively, exponentially) stable. If  is a normed space, then this definition may be formulated as in 1 above, if as norm
is a normed space, then this definition may be formulated as in 1 above, if as norm  one takes any norm compatible with the topology on
one takes any norm compatible with the topology on 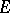 .
.
4) Let a differential equation (2) be given on a Riemannian manifold 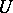 (for which a Euclidean or a Hilbert space can serve as a model) or, in a more general situation, on a Finsler manifold
(for which a Euclidean or a Hilbert space can serve as a model) or, in a more general situation, on a Finsler manifold  (for which a normed space can serve as a model); the distance function in
(for which a normed space can serve as a model); the distance function in 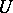 is denoted by
is denoted by 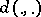 . A solution
. A solution 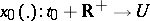 of this equation is called Lyapunov stable if for each
of this equation is called Lyapunov stable if for each 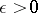 one can find a
one can find a 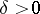 such that for each
such that for each  satisfying
satisfying 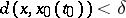 , the solution
, the solution 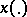 of the Cauchy problem (2),
of the Cauchy problem (2),  , is unique, defined for
, is unique, defined for 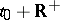 and satisfies the inequality
and satisfies the inequality  for all
for all 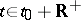 . If, in addition, one can find a
. If, in addition, one can find a 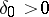 such that for every solution
such that for every solution 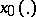 of (2) whose initial value satisfies the inequality
of (2) whose initial value satisfies the inequality 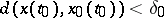 one has the equation
one has the equation
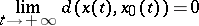 |
(the inequality
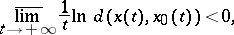 |
respectively), then the solution 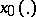 is called asymptotically (respectively, exponentially) stable.
is called asymptotically (respectively, exponentially) stable.
Suppose that the differential equation (2) is given on a compact differentiable manifold  . A solution of this equation is called Lyapunov stable (asymptotically, exponentially stable) if it becomes such when the manifold
. A solution of this equation is called Lyapunov stable (asymptotically, exponentially stable) if it becomes such when the manifold 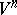 is equipped with some Riemannian metric. This property of the solution does not depend on the choice of the Riemannian metric.
is equipped with some Riemannian metric. This property of the solution does not depend on the choice of the Riemannian metric.
5) Let 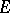 be a uniform space. Let
be a uniform space. Let
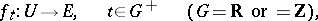 |
be a mapping defined on an open set 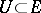 . A point
. A point 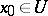 is called Lyapunov stable relative to the family of mappings
is called Lyapunov stable relative to the family of mappings 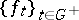 if for every entourage
if for every entourage 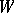 there exists a neighbourhood
there exists a neighbourhood  of
of  such that the set of all
such that the set of all 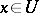 satisfying
satisfying 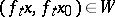 for all
for all 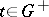 , is a neighbourhood of
, is a neighbourhood of 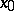 . If, in addition, there exists a neighbourhood
. If, in addition, there exists a neighbourhood 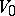 of
of 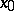 such that for every
such that for every 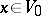 and every entourage
and every entourage 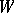 one can find a
one can find a 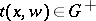 such that
such that 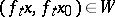 for all
for all 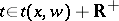 , then the point
, then the point 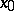 is called asymptotically stable.
is called asymptotically stable.
If 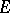 is a compact topological space and
is a compact topological space and  ,
, 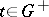 , is a mapping given on some open set
, is a mapping given on some open set  , then the point
, then the point 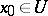 is called Lyapunov stable (asymptotically stable) relative to the family of mappings
is called Lyapunov stable (asymptotically stable) relative to the family of mappings  if it becomes such after the space
if it becomes such after the space  is equipped with the unique uniform structure that is compatible with the topology on
is equipped with the unique uniform structure that is compatible with the topology on  .
.
6) Let 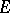 be a topological space and
be a topological space and 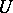 an open subspace in it. Let
an open subspace in it. Let 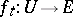 ,
, 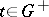 , where
, where 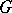 is
is 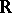 or
or 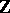 , be a mapping having
, be a mapping having 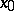 as fixed point. The fixed point
as fixed point. The fixed point  is called Lyapunov stable relative to the family of mappings
is called Lyapunov stable relative to the family of mappings 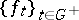 if for every neighbourhood
if for every neighbourhood 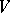 of
of 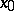 there exists a neighbourhood
there exists a neighbourhood 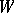 of the same point such that
of the same point such that 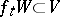 for all
for all 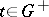 . If, in addition, there exists a neighbourhood
. If, in addition, there exists a neighbourhood 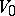 of
of  such that
such that 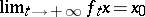 for every
for every 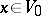 , then the point
, then the point 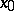 is called asymptotically stable relative to the family of mappings
is called asymptotically stable relative to the family of mappings 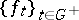 .
.
7) Lyapunov stability (asymptotic, exponential stability) of a solution  of an equation of arbitrary order,
of an equation of arbitrary order,  , is understood to mean Lyapunov stability (respectively asymptotic, exponential stability) of the solution
, is understood to mean Lyapunov stability (respectively asymptotic, exponential stability) of the solution 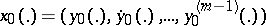 of the corresponding first-order equation (2), where
of the corresponding first-order equation (2), where  ,
,  .
.
Definitions 1, 2, 4, 6, 7 include stable motions of systems with a finite number of degrees of freedom (where the equations on manifolds arise naturally when considering mechanical systems with a constraint). Definitions 2–7 include stable motions in the mechanics of continuous media and in other parts of physics, stable solutions of operator equations, functional-differential equations (in particular, equations with retarded arguments) and other equations.
Study of the stability of an equilibrium position of an autonomous system.
Let 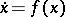 be an autonomous differential equation defined in a neighbourhood of a point
be an autonomous differential equation defined in a neighbourhood of a point 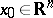 , where the function
, where the function 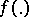 is continuously differentiable and vanishes at this point. If the real parts of all eigen values of the derivative
is continuously differentiable and vanishes at this point. If the real parts of all eigen values of the derivative 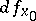 are negative, then the fixed point
are negative, then the fixed point  of
of 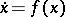 is exponentially stable (Lyapunov's theorem on stability in a first approximation); to facilitate the verification of the condition in this theorem one applies criteria for stability. If under these conditions at least one of the eigen values of the derivative
is exponentially stable (Lyapunov's theorem on stability in a first approximation); to facilitate the verification of the condition in this theorem one applies criteria for stability. If under these conditions at least one of the eigen values of the derivative 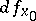 has positive real part (this condition may be checked without finding the eigen values themselves, cf. Stability criterion), then the fixed point of the differential equation
has positive real part (this condition may be checked without finding the eigen values themselves, cf. Stability criterion), then the fixed point of the differential equation  is unstable.
is unstable.
Example. The equation of the oscillation of a pendulum with friction is
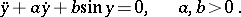 |
The lower equilibrium position 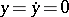 is exponentially stable, since the roots of the characteristic equation
is exponentially stable, since the roots of the characteristic equation 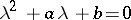 of the variational equation (cf. Variational equations) have negative real parts. The upper equilibrium position
of the variational equation (cf. Variational equations) have negative real parts. The upper equilibrium position 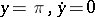 is unstable, since the characteristic equation
is unstable, since the characteristic equation 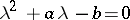 of the variational equation
of the variational equation  has a positive root. This instability takes place even in the absence of friction
has a positive root. This instability takes place even in the absence of friction 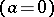 . The lower equilibrium position of a pendulum without friction is one of the so-called critical cases, when all eigen values of the derivative
. The lower equilibrium position of a pendulum without friction is one of the so-called critical cases, when all eigen values of the derivative 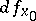 are contained in the left complex half-plane, and at least one of them lies on the imaginary axis.
are contained in the left complex half-plane, and at least one of them lies on the imaginary axis.
For the study of stability in critical cases, A.M. Lyapunov proposed the so-called second method for studying stability (cf. Lyapunov function). For a pendulum without friction,
 |
the lower equilibrium position is Lyapunov stable, since there exists a Lyapunov function
 |
— the total energy of the pendulum; the condition of non-positivity for the derivative of this function is a consequence of the law of conservation of energy.
A fixed point 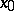 of a differentiable mapping
of a differentiable mapping  is exponentially stable relative to
is exponentially stable relative to 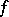 if all eigen values of the derivative
if all eigen values of the derivative 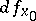 are less than 1 in modulus, and it is unstable if at least one of them has modulus
are less than 1 in modulus, and it is unstable if at least one of them has modulus 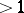 .
.
The study of the stability of periodic points of differentiable mappings reduces to the study of stability of fixed points relative to the powers of these mappings. Periodic solutions of autonomous differential equations are not asymptotically stable (cf. Orbit stability; Andronov–Witt theorem).
It should not be believed that exponential stability of the null solution of the variational equation of the autonomous differential equation 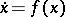 along a solution
along a solution 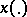 implies stability of the solution. This is shown by Perron's example (cf. [2], [3]):
implies stability of the solution. This is shown by Perron's example (cf. [2], [3]):
 | (3) |
for  the null solution of the system of variational equations,
the null solution of the system of variational equations,
 | (4) |
of the system (3) (along the null solution) is exponentially stable (the Lyapunov characteristic exponents of the system (4) are 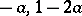 , cf. Lyapunov characteristic exponent), but for
, cf. Lyapunov characteristic exponent), but for  the null solution of the system (3) is unstable. However, stability in the first approximation is typical, in a sense explained below.
the null solution of the system (3) is unstable. However, stability in the first approximation is typical, in a sense explained below.
Let 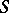 be the set of diffeomorphisms
be the set of diffeomorphisms  of a Euclidean space
of a Euclidean space  onto itself having uniformly continuous derivatives that satisfy the inequality
onto itself having uniformly continuous derivatives that satisfy the inequality
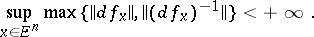 |
For each diffeomorphism 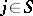 denote by
denote by 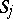 the set of all
the set of all 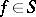 satisfying the inequality
satisfying the inequality
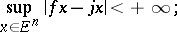 |
one endows 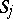 with the distance function
with the distance function
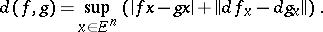 |
For each 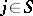 there is in
there is in 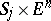 an everywhere-dense set
an everywhere-dense set 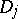 of type
of type  with the following property: If
with the following property: If  is such that for every
is such that for every 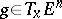 the inequality
the inequality
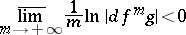 |
holds, then there is a neighbourhood 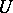 of
of 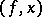 in
in 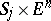 such that for every
such that for every  the point
the point 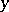 is exponentially stable relative to the diffeomorphism
is exponentially stable relative to the diffeomorphism  .
.
For a dynamical system given on a compact differentiable manifold, an analogous theorem can be formulated more simply and as a differential-topologically invariant statement. Let 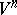 be a closed differentiable manifold. The set
be a closed differentiable manifold. The set 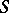 of all diffeomorphisms
of all diffeomorphisms 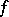 of class
of class 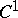 mapping
mapping 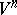 to
to 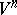 can be equipped with the
can be equipped with the 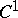 -topology. In the space
-topology. In the space 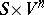 there is an everywhere-dense set
there is an everywhere-dense set 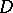 of type
of type  with the following property: If for an
with the following property: If for an  the inequality
the inequality
 |
holds for all 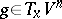 , then there is a neighbourhood
, then there is a neighbourhood 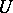 of
of 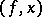 in
in 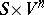 such that for each
such that for each  the point
the point 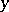 is exponentially stable relative to the diffeomorphism
is exponentially stable relative to the diffeomorphism  .
.
The concepts of Lyapunov stability, asymptotic stability and exponential stability were introduced by Lyapunov [1] in order to develop methods for studying stability in the sense of these definitions (cf. Lyapunov stability theory).
References
| [1] | A.M. Lyapunov, "Stability of motion" , Acad. Press (1966) (Translated from Russian) |
| [2] | O. Perron, "Ueber Stabilität und asymptotisches Verhalten der Integrale von Differentialgleichungssystemen" Math. Z. , 29 (1928) pp. 129–160 |
| [3] | R.E. Bellman, "Stability theory of differential equations" , Dover, reprint (1969) |
Comments
For stability questions of differential equations with discontinuous right-hand sides cf. [a6].
References
| [a1] | J.P. Lasalle, S. Lefschetz, "Stability by Lyapunov's direct method with applications" , Acad. Press (1961) |
| [a2] | M.W. Hirsch, S. Smale, "Differential equations, dynamical systems, and linear algebra" , Acad. Press (1974) |
| [a3] | W. Hahn, "Stability of motion" , Springer (1967) pp. 422 |
| [a4] | N.P. Bhatia, G.P. Szegö, "Stability theory of dynamical systems" , Springer (1970) pp. 30–36 |
| [a5] | M.I. Rabinovich, D.I. Trubetskov, "Oscillations and waves in linear and nonlinear systems" , Kluwer (1989) pp. Chapt. 6–7 (Translated from Russian) |
| [a6] | A.F. Filippov, "Differential equations with discontinuous righthand sides" , Kluwer (1989) pp. §15 (Translated from Russian) |
Lyapunov stability. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lyapunov_stability&oldid=14083