Limit elements
boundary elements, prime ends, of a domain
Elements of a domain 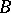 in the complex plane that are defined as follows. Let
in the complex plane that are defined as follows. Let 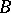 be a simply-connected domain of the extended complex plane, and let
be a simply-connected domain of the extended complex plane, and let 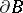 be the boundary of
be the boundary of 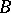 . A section
. A section  of
of 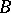 is defined as any simple Jordan arc
is defined as any simple Jordan arc  , closed in the spherical metric, with ends
, closed in the spherical metric, with ends  and
and 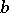 (including the cases
(including the cases 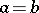 and
and  ), such that
), such that 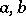 belong to
belong to 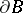 , and such that the arc
, and such that the arc  subdivides
subdivides 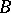 into two subdomains such that the boundary of each of them contains a point belonging to
into two subdomains such that the boundary of each of them contains a point belonging to 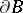 and different from
and different from  and
and 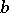 . A sequence
. A sequence 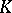 of sections
of sections 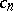 of a domain
of a domain 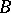 is called a chain if: 1) the diameter of
is called a chain if: 1) the diameter of 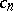 tends to zero as
tends to zero as 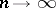 ; 2) for each
; 2) for each  the intersection
the intersection 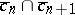 is empty; and 3) any path connecting a fixed point
is empty; and 3) any path connecting a fixed point 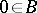 in
in 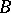 with the section
with the section 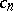 ,
,  , intersects
, intersects 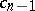 . Two chains
. Two chains 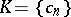 and
and  in
in 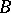 are equivalent if any section
are equivalent if any section 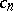 separates in
separates in 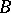 the point
the point  from all sections
from all sections 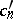 , except for a finite number of them. An equivalence class of chains in
, except for a finite number of them. An equivalence class of chains in 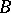 is called a limit element, or prime end, of
is called a limit element, or prime end, of 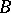 .
.
Let 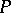 be a prime end of
be a prime end of 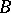 defined by a chain
defined by a chain 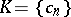 , and let
, and let  be that one of the two subdomains into which
be that one of the two subdomains into which  is subdivided by
is subdivided by 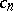 which does not contain
which does not contain  . The set
. The set 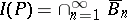 is called the impression or the support of the prime end. The impression of a prime end consists of boundary points and does not depend on the selection of the chain
is called the impression or the support of the prime end. The impression of a prime end consists of boundary points and does not depend on the selection of the chain  in the equivalence class. A principal point of a prime end is a point of it to which sections of at least one of the chains defining the prime end converge. A neighbouring, or subsidiary, point of a prime end is any point of it which is not a principal point of it. Any prime end contains at least one principal point. The principal points of a prime end form a closed set. The following is the Carathéodory classification [1] of prime ends: Elements of the first kind contain a single principal point and no subsidiary points; elements of the second kind contain one principal point and infinitely many subsidiary points; elements of the third kind contain a continuum of principal points and no subsidiary points; elements of the fourth kind contain a continuum of principal points and infinitely many subsidiary points.
in the equivalence class. A principal point of a prime end is a point of it to which sections of at least one of the chains defining the prime end converge. A neighbouring, or subsidiary, point of a prime end is any point of it which is not a principal point of it. Any prime end contains at least one principal point. The principal points of a prime end form a closed set. The following is the Carathéodory classification [1] of prime ends: Elements of the first kind contain a single principal point and no subsidiary points; elements of the second kind contain one principal point and infinitely many subsidiary points; elements of the third kind contain a continuum of principal points and no subsidiary points; elements of the fourth kind contain a continuum of principal points and infinitely many subsidiary points.
Another, equivalent, definition was given by P. Koebe [2]. It is based on equivalence classes of paths. The principal theorem in the theory of prime ends is the theorem of Carathéodory: Under a univalent conformal mapping of a domain  onto the unit disc
onto the unit disc 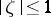 there is a one-to-one correspondence between the points of the circle and the prime ends of
there is a one-to-one correspondence between the points of the circle and the prime ends of 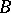 , and each sequence of points of
, and each sequence of points of 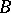 which converges to a prime end
which converges to a prime end 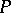 becomes a sequence of points in the unit disc which converge to a point
becomes a sequence of points in the unit disc which converge to a point 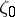 ,
, 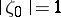 , this point being the image of
, this point being the image of  .
.
References
| [1] | C. Carathéodory, "Ueber die Begrenzung einfach zusammenhängender Gebiete" Math. Ann. , 73 (1913) pp. 323–370 |
| [2] | P. Koebe, "Abhandlungen zur Theorie der konformen Abbildung. I" J. Reine Angew. Math. , 145 (1915) pp. 177–223 |
| [3] | G.D. Suvorov, "Families of plane topological mappings" , Novosibirsk (1965) (In Russian) |
| [4] | A.I. Markushevich, "Theory of functions of a complex variable" , 3 , Chelsea (1977) (Translated from Russian) |
| [5] | E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966) pp. Chapt. 9 |
Comments
Instead of "section" the phrase cross cut or cut is also used.
The (only) point of a prime end of the first kind is an accessible boundary point (cf. Attainable boundary point). See also Conformal mapping, boundary properties of a.
Instead of prime end one also finds Carathéodory end in the literature.
There is a second, not entirely dissimilar notion in the literature which goes by the name "end of a topological spaceend" . This refers to the ends of a topological space.
References
| [a1] | M. Ohtsuka, "Dirichlet problem, extremal length and prime ends" , v. Nostrand (1967) |
Limit elements. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Limit_elements&oldid=18944