Lebesgue decomposition
The Lebesgue decomposition of a function of bounded variation is a canonical representation of this function as a sum of at most three terms. If  is a function of bounded variation on the interval
is a function of bounded variation on the interval  , then it can be represented in the form
, then it can be represented in the form
 |
where 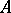 is an absolutely-continuous function (see Absolute continuity),
is an absolutely-continuous function (see Absolute continuity),  is a singular function and
is a singular function and 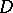 is a jump function. In certain cases, for example, if
is a jump function. In certain cases, for example, if 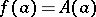 , this representation is unique. The Lebesgue decomposition was established by H. Lebesgue (1904) (see [1]).
, this representation is unique. The Lebesgue decomposition was established by H. Lebesgue (1904) (see [1]).
References
| [1] | H. Lebesgue, "Leçons sur l'intégration et la récherche des fonctions primitives" , Gauthier-Villars (1928) |
| [2] | I.P. Natanson, "Theorie der Funktionen einer reellen Veränderlichen" , H. Deutsch , Frankfurt a.M. (1961) (Translated from Russian) |
| [3] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) |
B.I. Golubov
The Lebesgue decomposition of a 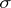 -finite signed measure
-finite signed measure 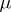 defined on a measurable space
defined on a measurable space 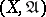 (
(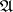 is a
is a  -algebra), with respect to a
-algebra), with respect to a 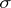 -finite signed measure
-finite signed measure  defined on this space, is a representation of
defined on this space, is a representation of 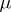 in the form
in the form 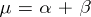 , where
, where 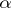 and
and 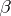 are
are 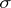 -finite signed measures,
-finite signed measures,  is absolutely continuous (cf. Absolute continuity) with respect to
is absolutely continuous (cf. Absolute continuity) with respect to  , and
, and  is singular with respect to
is singular with respect to  (cf. Mutually-singular measures). Such a representation is always possible and unique.
(cf. Mutually-singular measures). Such a representation is always possible and unique.
References
| [1] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) |
| [2] | N. Dunford, J.T. Schwartz, "Linear operators" , 1–3 , Interscience (1958–1971) |
Lebesgue decomposition. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lebesgue_decomposition&oldid=13177