Difference between revisions of "Hodgkin-Huxley system"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Hodgkin–Huxley system to Hodgkin-Huxley system: ascii title) |
(No difference)
| |
Revision as of 18:52, 24 March 2012
A system of four reaction-diffusion equations (cf. Reaction-diffusion equation) modelling the electrical activity of nerve cells. The equations have the form
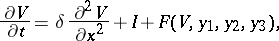 |
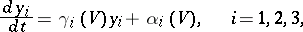 |
where 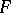 ,
, 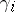 and
and  are non-linear functions, fitted into experimental data and corresponding to a biochemical model,
are non-linear functions, fitted into experimental data and corresponding to a biochemical model,  is time and
is time and  is one-dimensional space.
is one-dimensional space.
When  , undamped travelling-wave solutions, the action potentials (cf. Action potential), have been studied using the Conley index. They include single-pulse solutions, trains of finitely many impulses and periodic solutions.
, undamped travelling-wave solutions, the action potentials (cf. Action potential), have been studied using the Conley index. They include single-pulse solutions, trains of finitely many impulses and periodic solutions.
The case 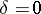 corresponds to a special experimental setting called a current clamp. The equations reduce to a four-dimensional autonomous system of ordinary differential equations, its homoclinic and periodic solutions, called stationary action potentials, arising through Hopf (or more degenerate) bifurcations (cf. also Homoclinic point; Homoclinic bifurcations; Hopf bifurcation).
corresponds to a special experimental setting called a current clamp. The equations reduce to a four-dimensional autonomous system of ordinary differential equations, its homoclinic and periodic solutions, called stationary action potentials, arising through Hopf (or more degenerate) bifurcations (cf. also Homoclinic point; Homoclinic bifurcations; Hopf bifurcation).
Modifications in the equation that retain the form above, with possibly more variables, abound in the biological literature, accounting for variations in the biochemistry of cells. There is also a simplified version that has been much studied by mathematicians, the FitzHugh–Nagumo equations.
References
| [a1] | A.L. Hodgkin, A. F. Huxley, "A quantitative description of membrane current and its application to conduction and excitation in nerve" J. Physiology , 117 (1952) pp. 500–544 (Reprint: Bull. Math. Biology 52 (1990), 25–71) |
| [a2] | J. Rinzel, "Electrical excitability of cells, theory and experiment: review of the Hodgkin–Huxley foundation and an update" Bull. Math. Biology , 52 (1990) pp. 5–23 |
Hodgkin-Huxley system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hodgkin-Huxley_system&oldid=22581