Exceptional analytic set

An analytic set 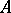in a complex space 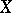for which there exists an analytic mapping 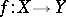such that 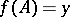is a point in the complex space 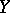, while 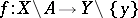is an analytic isomorphism. The modification 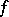is called a contraction of to .

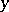The problem of characterizing exceptional sets arose in algebraic geometry in relation to the study of birational transformations (cf. Birational transformation and also Exceptional subvariety). Very general criteria for exceptional sets have been found in analytic geometry. More precisely, let be a connected compact analytic set of positive dimension in a complex space 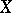. The set 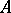is exceptional if and only if there is a relatively-compact pseudo-convex neighbourhood of it in in which it is a maximal compact analytic subset.

Let 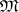be a coherent sheaf of ideals whose zero set coincides with and let 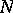be the restriction to 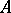of the linear space over 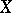dual to 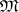(cf. Vector bundle, analytic). For 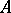to be exceptional it is sufficient that 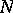be weakly negative (cf. Positive vector bundle). If 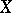is a manifold and 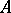is a submanifold of it, then 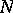is the normal bundle over 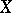. Sometimes, the bundle 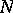being weakly negative is also necessary (e.g. if 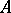is a submanifold of codimension 1, isomorphic to , or if is a two-dimensional manifold). In particular, a curve 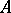on a complex surface 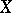is exceptional if and only if the intersection matrix of its irreducible components is negative definite (cf. [1], [2]). The structure of a neighbourhood of an exceptional analytic set 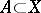is completely determined by the ringed space 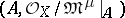for sufficiently large 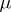. Exceptional analytic sets have the following transitiveness condition: If 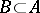is a compact analytic space in 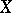and is exceptional in , while 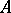is exceptional in 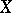, then 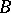is exceptional in 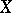[6]. There are relative generalizations of the concept of an exceptional analytic set. These consider, roughly speaking, the simultaneous contraction of a family of analytic sets in an analytic family of complex spaces. An analogue of Grauert's criterion mentioned above is valid in this case (cf. [2]).

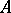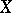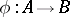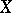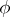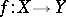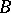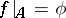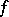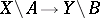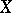Another natural generalization of the concept of an exceptional analytic set is as follows. Let be a subspace in and let a proper surjective holomorphic mapping be given. A contraction of along is a proper surjective holomorphic mapping , where contains as a subspace, such that and induces an isomorphism . 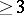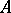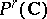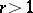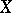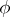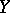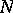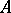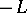If is a manifold of dimension , is a compact submanifold of codimension one in it, and is a fibration with fibre , , then a necessary and sufficient condition for to be contractible along onto a manifold is: The normal bundle over (which in this case coincides with the bundle corresponding to the divisor ) must induce a bundle on each fibre , where 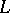is determined by a hyperplane in 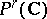. The corresponding contraction is the inverse to the monoidal transformation with centre at 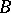(cf. [3]). On the other hand, for each modification 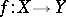, where 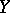is a manifold, 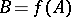is a submanifold of it, 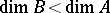, and is an isomorphism, the mapping 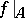is a fibration with fibre 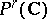. Criteria for contractibility along 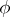, as well as in more general situations, are known (cf. [4]). If 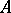is exceptional in 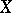and is a holomorphic retract of it (e.g. 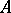is the zero section of a weakly-negative vector bundle), then 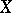has a contraction along any 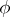. If, moreover, the dimensions of the fibres of the retract 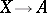are equal to at least , one can completely recover the initial space from the data 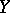obtained after contraction [5].

References

[1]	H. Grauert, "Ueber Modifikationen und exzeptionelle analytische Mengen" Math. Ann. , 146 (1962) pp. 331–368
[2]	V. Ancona, "Un teorema di contrattibilità relativa" Boll. Unione Mat. Ital. , 9 : 3 (1974) pp. 785–790
[3]	A. Fujiki, S. Nakano, "Supplement to "On the inverse of monodial transformations" " Publ. Res. Inst. Math. Sci. , 7 : 3 (1972) pp. 637–644
[4]	A. Fujiki, "On the blowing down of analytic spaces" Publ. Res. Inst. Math. Sci. , 10 : 2 (1975) pp. 473–507
[5]	K. Takijima, T. Suzuki, "On the trivial extension of equivalence relations on analytic spaces" Trans. Amer. Math. Soc. , 219 (1976) pp. 369–377
[6]	V.A. Krasnov, "Transitivity of exceptional subspaces" Math. USSR-Izv. , 9 : 1 (1975) pp. 13–20 Izv. Akad. Nauk SSSR Ser. Mat. , 39 : 1 (1975) pp. 15–22

References

[a1]	R. Hartshorne, "Algebraic geometry" , Springer (1977)