Elliptic geometry
A geometry in a space with a Riemannian curvature that is constant and positive in any two-dimensional direction. Elliptic geometry is a higher-dimensional generalization of the Riemann geometry.
Comments
Thus, elliptic geometry is the geometry of real projective space endowed with positive sectional curvature (i.e. the geometry of the sphere in  with antipodal points, or antipodes, identified). An exposition of it is given in [a1], Chapt. 19; generalizations are given in [a2]. Some details follow.
with antipodal points, or antipodes, identified). An exposition of it is given in [a1], Chapt. 19; generalizations are given in [a2]. Some details follow.
Let  be an
be an  -dimensional Euclidean space and
-dimensional Euclidean space and  the associated projective space of all straight lines through the origin. For
the associated projective space of all straight lines through the origin. For  let
let  be the angle (in the Euclidean sense) between the lines
be the angle (in the Euclidean sense) between the lines  and
and  in
in  . If
. If  and
and  are two lines in
are two lines in  intersecting in
intersecting in  , then the angle between
, then the angle between  and
and 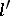 is the angle in
is the angle in  between the corresponding planes
between the corresponding planes  and
and  in
in  (which intersect in the line
(which intersect in the line  ). The space
). The space  with this metric (and this notion of angle) is called the elliptic space associated with
with this metric (and this notion of angle) is called the elliptic space associated with  . It is of course closely related to the spherical geometry of
. It is of course closely related to the spherical geometry of  , being in fact a quotient. The topology induced by the metric is the usual one.
, being in fact a quotient. The topology induced by the metric is the usual one.
Consider for the moment the spherical geometry of 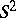 , i.e. the lines are great circles. Take e.g. the equator. Then all lines in
, i.e. the lines are great circles. Take e.g. the equator. Then all lines in  perpendicular to the equator meet in the North and South poles, the polar points of the equator. Identifying antipodal points one obtains
perpendicular to the equator meet in the North and South poles, the polar points of the equator. Identifying antipodal points one obtains  , in which therefore for every line
, in which therefore for every line  there is unique point point
there is unique point point 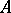 , the (absolute) polar of
, the (absolute) polar of  through which every line perpendicular to
through which every line perpendicular to 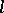 passes. Conversely, to every point
passes. Conversely, to every point  of
of  there corresponds an (absolute) polar line.
there corresponds an (absolute) polar line.
This generalizes. Let 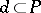 be an
be an  -dimensional plane in
-dimensional plane in 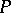 , then the (absolute) polar of
, then the (absolute) polar of 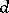 in
in  is the plane
is the plane  of dimension
of dimension  consisting of all points
consisting of all points  such that for all
such that for all  ,
,  . Thus, for
. Thus, for  the polar of a line is a line.
the polar of a line is a line.
References
| [a1] | M. Berger, "Geometry" , II , Springer (1987) |
| [a2] | H. Busemann, "Recent synthetic differential geometry" , Springer (1970) |
Elliptic geometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Elliptic_geometry&oldid=15989