E-function
The concept of 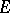 -functions was introduced by C.L. Siegel in [a1], p. 223, in his work on generalizations of the Lindemann–Weierstrass theorem.
-functions was introduced by C.L. Siegel in [a1], p. 223, in his work on generalizations of the Lindemann–Weierstrass theorem.
Consider a Taylor series of the form
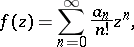 |
where the numbers 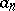 belong to a fixed algebraic number field (cf. also Algebraic number; Field)
belong to a fixed algebraic number field (cf. also Algebraic number; Field) 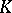 (
(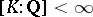 ). Suppose it satisfies the following conditions:
). Suppose it satisfies the following conditions:
i) 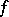 satisfies a linear differential equation with polynomial coefficients;
satisfies a linear differential equation with polynomial coefficients;
ii) for any  one has
one has  . Then
. Then 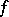 is called an
is called an 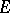 -function. Here, the notation
-function. Here, the notation  stands for the so-called projective height, given by
stands for the so-called projective height, given by
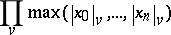 |
for any 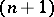 -tuple
-tuple 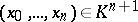 . The product is taken over all valuations
. The product is taken over all valuations 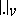 of
of 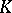 (cf. also Norm on a field). When the
(cf. also Norm on a field). When the 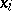 are rational numbers,
are rational numbers, 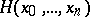 is simply the maximum of the absolute values of the
is simply the maximum of the absolute values of the 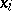 times their common denominator. As suggested by their name,
times their common denominator. As suggested by their name, 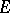 -functions are a variation on
-functions are a variation on 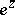 . A large class of examples is given by the hypergeometric functions (cf. Hypergeometric function) of the form
. A large class of examples is given by the hypergeometric functions (cf. Hypergeometric function) of the form
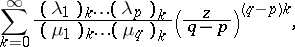 |
where 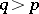 ,
, 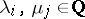 for all
for all 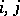 and
and 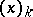 is the Pochhammer symbol, given by
is the Pochhammer symbol, given by 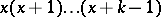 . Motivated by the success of the Lindemann–Weierstrass theorem and techniques of A. Thue and W. Maier, Siegel was the first to define and study them. He found a number of transcendence results on values of
. Motivated by the success of the Lindemann–Weierstrass theorem and techniques of A. Thue and W. Maier, Siegel was the first to define and study them. He found a number of transcendence results on values of  -functions at algebraic points. These results were published in 1929 and later, in 1949, a more systematic account appeared in [a2]. Unfortunately, Siegel's main result contains a normality condition on the differential equations which, in practice, seemed very hard to verify. This condition was removed by A.B. Shidlovskii, around 1955 [a3]. Roughly speaking, if
-functions at algebraic points. These results were published in 1929 and later, in 1949, a more systematic account appeared in [a2]. Unfortunately, Siegel's main result contains a normality condition on the differential equations which, in practice, seemed very hard to verify. This condition was removed by A.B. Shidlovskii, around 1955 [a3]. Roughly speaking, if 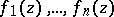 are
are 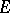 -functions that are algebraically independent over
-functions that are algebraically independent over 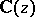 (cf. Algebraic independence), then the values
(cf. Algebraic independence), then the values 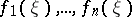 are algebraically independent over
are algebraically independent over 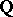 for all algebraic
for all algebraic  excepting a known finite set. Thus, proving the algebraic independence of values of
excepting a known finite set. Thus, proving the algebraic independence of values of 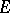 -functions at algebraic points has been reduced to the problem of showing algebraic independence over
-functions at algebraic points has been reduced to the problem of showing algebraic independence over 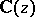 of functions satisfying linear differential equations. During the last thirty years the latter problem has been the object of study of a school of Russian mathematicians and a few non-Russian mathematicians as well. Many of these results are contained in [a4]. In recent years, F. Beukers, W.D. Brownawell and G. Heckman studied these problems with the powerful techniques from differential Galois theory, see [a5], [a6], [a7], and also Galois differential group.
of functions satisfying linear differential equations. During the last thirty years the latter problem has been the object of study of a school of Russian mathematicians and a few non-Russian mathematicians as well. Many of these results are contained in [a4]. In recent years, F. Beukers, W.D. Brownawell and G. Heckman studied these problems with the powerful techniques from differential Galois theory, see [a5], [a6], [a7], and also Galois differential group.
See also  -function.
-function.
References
| [a1] | C.L. Siegel, "Über einige Anwendungen diophantischer Approximationen" , Ges. Abhandlungen , I , Springer (1966) |
| [a2] | C.L. Siegel, "Transcendental numbers" , Ann. Math. Studies , 16 , Princeton Univ. Press (1949) |
| [a3] | A.B. Shidlovskii, "A criterion for algebraic independence of the values of a class of entire functions" Amer. Math. Soc. Transl. Ser. 2 , 22 (1962) pp. 339–370 Izv. Akad. SSSR Ser. Math. , 23 (1959) pp. 35–66 |
| [a4] | A.B. Shidlovskii, "Transcendental numbers" , De Gruyter (1989) (In Russian) |
| [a5] | F. Beukers, W.D. Brownawell, G. Heckman, "Siegel normality" Ann. of Math. , 127 (1988) pp. 279–308 |
| [a6] | N.M. Katz, "Differential Galois theory and exponential sums" , Ann. Math. Studies , Princeton Univ. Press (1990) |
| [a7] | F. Beukers, "Differential Galois theory" M. Waldschmidt (ed.) P. Moussa (ed.) J.M. Luck (ed.) C. Itzykson (ed.) , From Number Theory to Physics , Springer (1995) pp. Chapt. 8 |
E-function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=E-function&oldid=14699