Difference between revisions of "Cornu spiral"
(TeX) |
m (OldImage template added) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 2: | Line 2: | ||
''clothoid'' | ''clothoid'' | ||
| − | A transcendental plane curve (see Fig.) whose natural equation is | + | A transcendental plane curve (see Fig.) whose [[natural equation]] is |
$$r=\frac as,$$ | $$r=\frac as,$$ | ||
| Line 8: | Line 8: | ||
where $r$ is the radius of curvature, $a=\text{const}$ and $s$ is the arc length. It can be parametrized by the [[Fresnel integrals|Fresnel integrals]] | where $r$ is the radius of curvature, $a=\text{const}$ and $s$ is the arc length. It can be parametrized by the [[Fresnel integrals|Fresnel integrals]] | ||
| − | $$x=\int\limits_0^t\cos\frac{s^2}{2a}ds,\quad y=\int\limits_0^t\sin\frac{s^2}{2a}ds,$$ | + | $$x=\int\limits_0^t\cos\frac{s^2}{2a}\,ds,\quad y=\int\limits_0^t\sin\frac{s^2}{2a}\,ds,$$ |
| − | which are well-known in diffraction theory. The spiral of Cornu touches the horizontal axis at the origin. The asymptotic points are $M_1(\sqrt{\pi a}/2,\sqrt{\pi a}/2)$ and $ | + | which are well-known in diffraction theory. The spiral of Cornu touches the horizontal axis at the origin. The asymptotic points are $M_1(\sqrt{\pi a}/2,\sqrt{\pi a}/2)$ and $M_2(-\sqrt{\pi a}/2,-\sqrt{\pi a}/2)$. |
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/c026510a.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/c026510a.gif" /> | ||
| Line 19: | Line 19: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> E. Jahnke, F. Emde, F. Lösch, "Tafeln höheren Funktionen" , Teubner (1966)</TD></TR></table> | + | <table> |
| + | <TR><TD valign="top">[1]</TD> <TD valign="top"> E. Jahnke, F. Emde, F. Lösch, "Tafeln höheren Funktionen" , Teubner (1966)</TD></TR> | ||
| + | <TR><TD valign="top">[a1]</TD> <TD valign="top"> J.D. Lawrence, "A catalog of special plane curves" , Dover, reprint (1972) </TD></TR> | ||
| + | </table> | ||
| − | + | {{OldImage}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 10:58, 26 March 2023
clothoid
A transcendental plane curve (see Fig.) whose natural equation is
$$r=\frac as,$$
where $r$ is the radius of curvature, $a=\text{const}$ and $s$ is the arc length. It can be parametrized by the Fresnel integrals
$$x=\int\limits_0^t\cos\frac{s^2}{2a}\,ds,\quad y=\int\limits_0^t\sin\frac{s^2}{2a}\,ds,$$
which are well-known in diffraction theory. The spiral of Cornu touches the horizontal axis at the origin. The asymptotic points are $M_1(\sqrt{\pi a}/2,\sqrt{\pi a}/2)$ and $M_2(-\sqrt{\pi a}/2,-\sqrt{\pi a}/2)$.
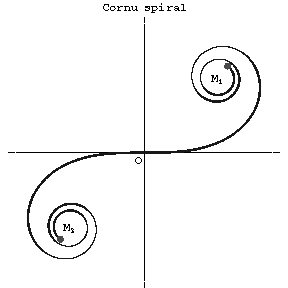
Figure: c026510a
The spiral of Cornu is sometimes called the spiral of Euler after L. Euler, who mentioned it first (1744). Beginning with the works of A. Cornu (1874), the spiral of Cornu is widely used in the calculation of diffraction of light.
References
| [1] | E. Jahnke, F. Emde, F. Lösch, "Tafeln höheren Funktionen" , Teubner (1966) |
| [a1] | J.D. Lawrence, "A catalog of special plane curves" , Dover, reprint (1972) |
Cornu spiral. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cornu_spiral&oldid=32541