Analytic vector

in the space 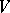of a representation 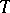of a Lie group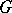

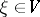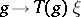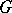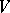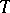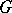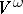A vector for which the mapping is a real-analytic vector function on with values in (cf. Representation theory). If is a weakly-continuous representation of a Lie group in a Banach space , then the set of analytic vectors is dense in [1], [2], [3]. This theorem has been generalized to a wide class of representations in locally convex spaces [5]. 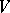It has been proved [6] that a representation of a connected Lie group in a Banach space is uniquely determined by the corresponding representation of the Lie algebra of the Lie group in the space .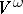

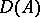An analytic vector for an unbounded operator on a Banach space , defined on a domain , is defined as a vector

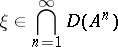for which the series

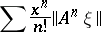has a positive radius of convergence. This notion, which was introduced in [2], is a special case of the general concept of an analytic vector; here the set of points on the real line with the addition operation plays the role of the Lie group . It was found useful in the theory of operators on Banach spaces and in the theory of elliptic differential operators.

References

[1]	P. Cartier, J. Dixmier, "Vecteurs analytiques dans les répresentations de groupes de Lie" Amer. J. Math. , 80 (1958) pp. 131–145
[2]	E. Nelson, "Analytical vectors" Ann. of Math. , 70 (1969) pp. 572–615
[3]	L. Gårding, "Vecteurs analytiques dans les répresentations des groups" Bull. Soc. Math. France , 88 (1960) pp. 73–93
[4]	P. Cartier, "Vecteurs analytiques" , Sem. Bourbaki 1958/1959 , 181 (1959) pp. 12–27
[5]	R.T. Moore, "Measurable, continuous and smooth vectors for semigroup and group representations" Mem. Amer. Math. Soc. , 78 (1968)
[6]	Harish-Chandra, "Representations of a semisimple Lie group on a Banach space I" Trans. Amer. Math. Soc. , 75 (1953) pp. 185–243

Comments

Integrability to the corresponding (simply-connected and connected) Lie group 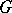of a representation of a Lie algebra 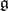in a Hilbert space follows from the existence of a dense set of analytic vectors for the "Laplacian" 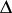, the sum of the squares of skew-symmetric representatives with a common dense invariant domain 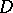, of linear generators of (Nelson's criterion, [2]). More practical criteria were developed later, e.g. the existence of common analytic vectors for Lie generators (the criterion, [a2], [a3], [a4]), as well as similar results in more general spaces and a study of separate versus joint analyticity.

References

[a1]	G. Warner, "Harmonic analysis on semi-simple Lie groups" , 1 , Springer (1972)
[a2]	M. Flato, D. Sternheimer, "Deformations of Poisson brackets, separate and joint analyticity in group representations, nonlinear group representations and physical applications" J.A. Wolf (ed.) M. Cahen (ed.) M. De Wilde (ed.) , Harmonic Analysis and Representations of Semisimple Lie Groups , Reidel (1980) pp. 385–448
[a3]	J. Simon, "On the integrability of representations of finite dimensional real Lie algebras" Commun. Math. Phys. , 28 (1972) pp. 39–46
[a4]	M. Flato, J. Simon, H. Snellman, D. Sternheimer, "Simple facts about analytic vectors and integrability" Ann. Scient. Ec. Norm. Sup. Ser. 4 , 5 (1972) pp. 423–434